Locus of the intersection of the two straight lines passing through respectively and including an angle of can be a circle with
curve and radius
centre and radius 2
centre and radius
centre and radius
The correct Answer is:C, D
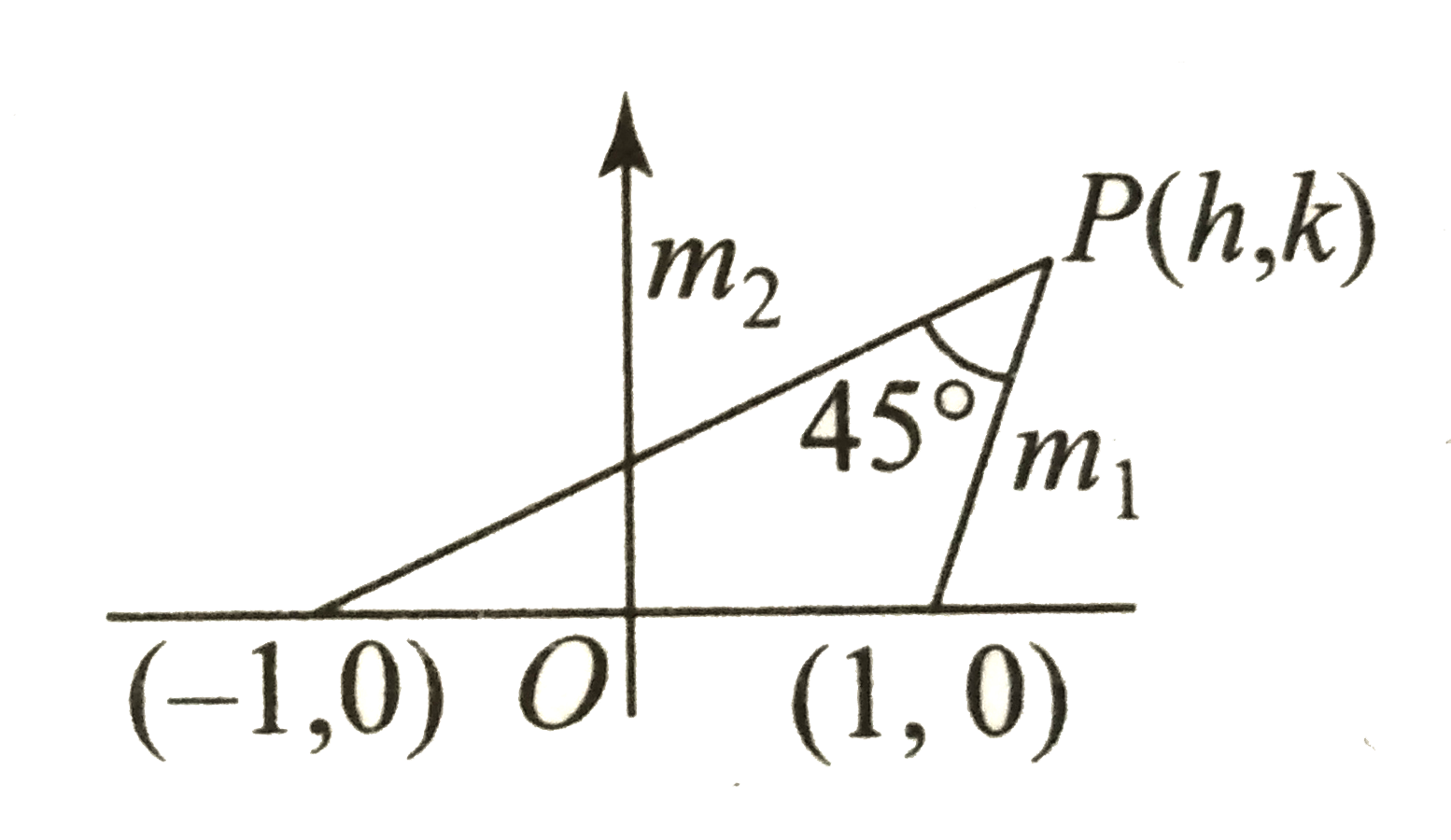
Clearly, and
Locus is:
or
Thus, centre is (0,1) or and radius is .
Topper's Solved these Questions
- CIRCLESBook:CENGAGEChapter:CIRCLESExercise:Comprehension Type
Explore 8 Videos
- CIRCLESBook:CENGAGEChapter:CIRCLESExercise:Single Correct Answer Type
Explore 58 Videos
- CIRCLE Book:CENGAGEChapter:CIRCLE Exercise:JEE Advanced (Single Correct Answer Type)
Explore 14 Videos
- COMPLEX NUMBERSBook:CENGAGEChapter:COMPLEX NUMBERSExercise:Question Bank
Explore 30 Videos
Similar Questions
Knowledge Check
The area (in square units ) of the triangle formed by y-axis , the straight line L passing through (1,1) and (2,0) and the straight line perpendicular to the line L and passing through (1/2, 0) , is
A258B254C2516D252
Find the equations of the straight lines passing through (2,-10 and making and angle of 45∘ with the line 6x+5y−8=0
View SolutionFind the equations t the straight lines passing through the point (2,-3) and inclined at an angle of 45∘ to the line y=3x+4.
View SolutionFind the equations of the straight lines passing through the point (-3, 2) and making an angle of 45° with the straight line 3x−y+4=0.
View SolutionThe locus of the foot of the perpendicular from the origin to a straight line passing through (1,1) is
View SolutionFind the equation f eth straight lines passing through the origins and making an angle of 45∘ with the straight line √3x+y=11.
View SolutionThe equation of straight line passing through the point (-3,2) and making an angle 45∘ with the straight line 3x-y+4=0 is
View SolutionFind the equation of the straight liens passing through the origin making an angle 450 with straight line √3x+y=11
View Solution