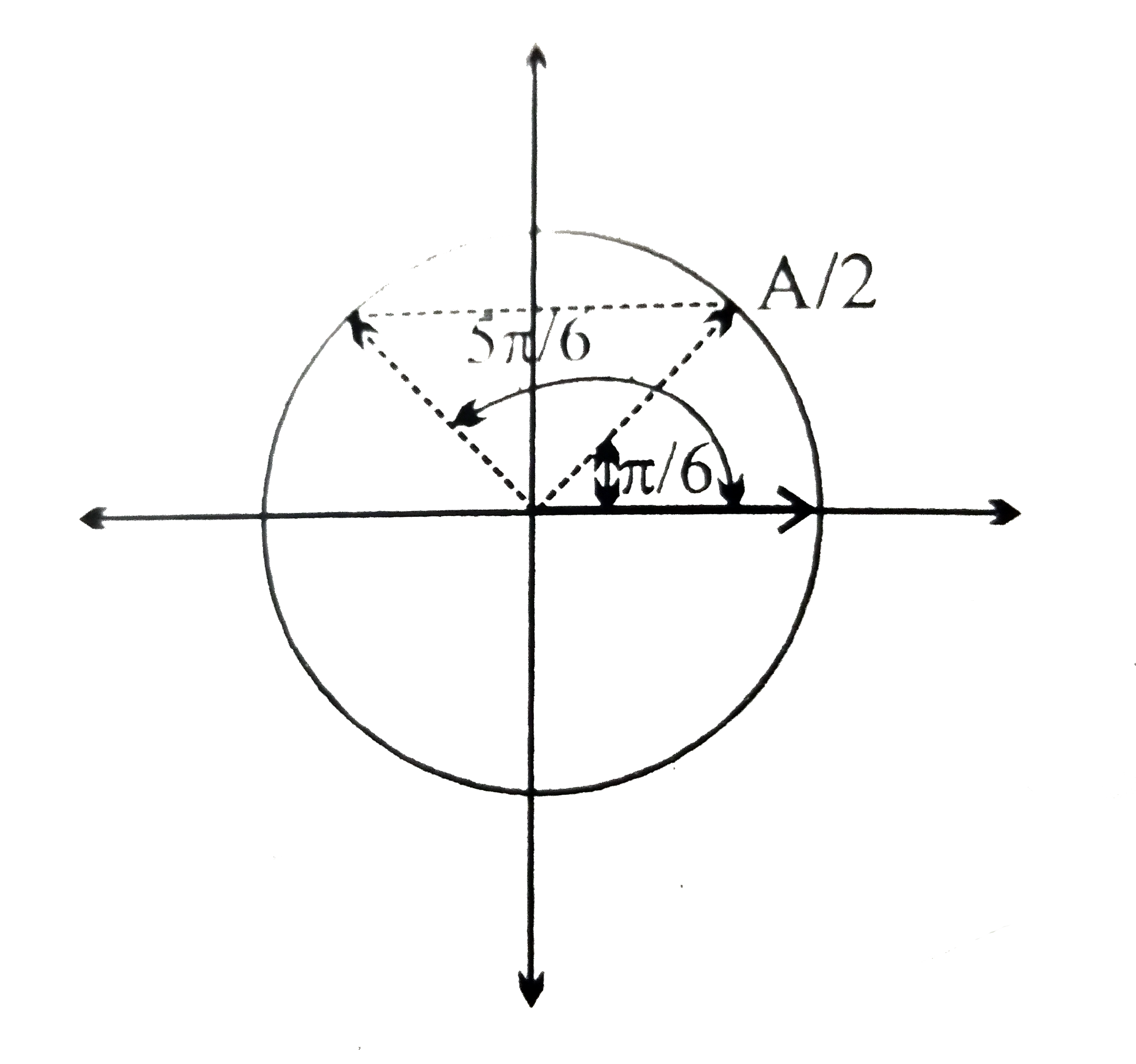
A particle performing S.H.M . Undergoes displacement A/2 (where A= amplitude of S.H.M. ) in one second. At t=0 the particle was located at either extreme position or mean position. The time period of S.H.M. can be :( consider all possible cases )

12s

2.4 s

6 s

1.2s

Text Solution

Verified by Experts

ABCD
$\infty = \frac{θ}{t}$
$\infty_{1}^{2} = π / 6, \frac{2 π}{T_{1}} = \frac{π}{6}, T_{1} = 12$
$\infty_{2} = 5 π / 6, \frac{2 π}{T_{2}} = \frac{π}{6}, T_{2} = 2.4$
$T_{1} = 12 sec$
$T_{2} = 2.4 sec$

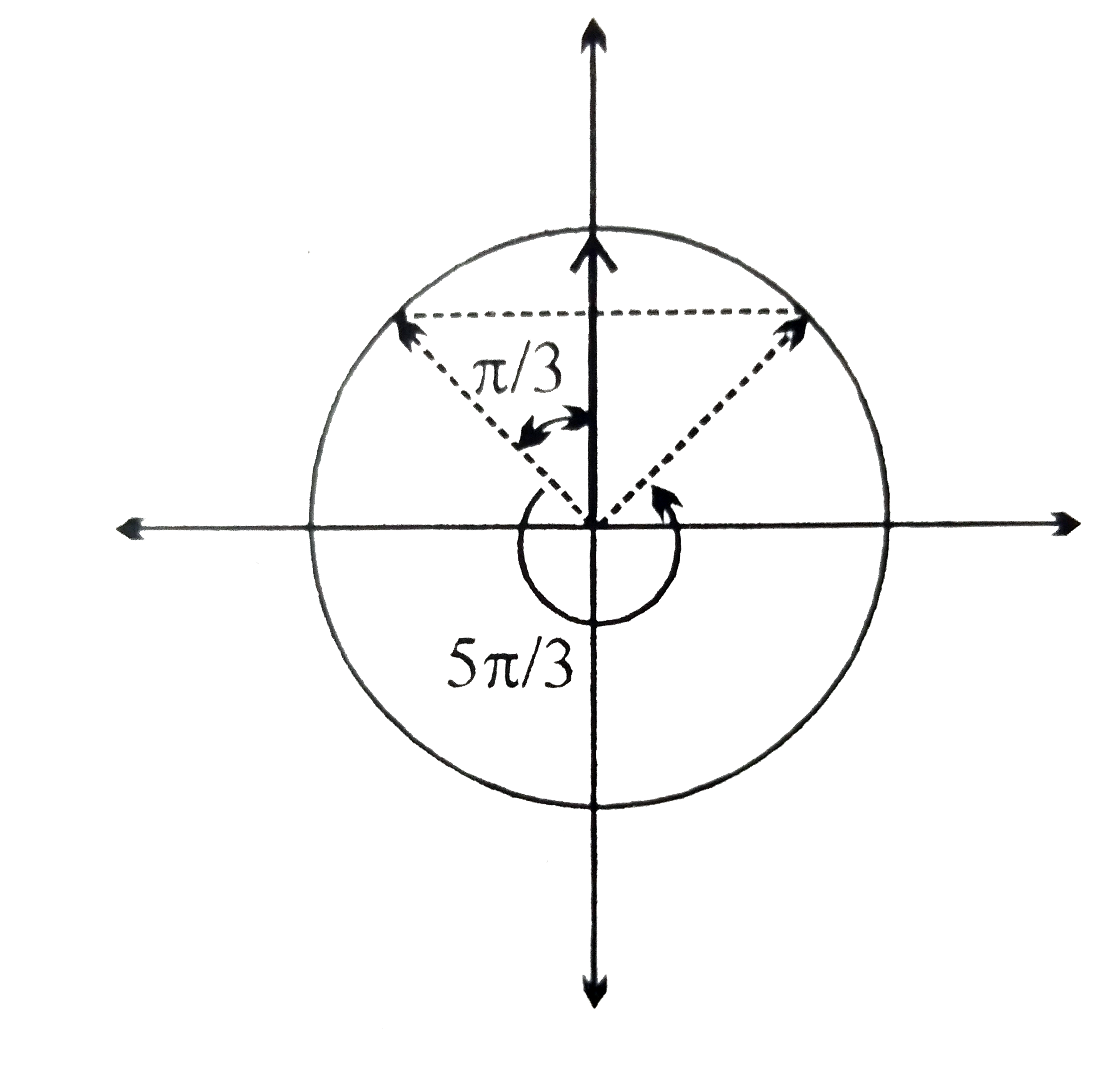
$θ_{1}^{1} = \frac{π / 3}{1}, \frac{2 π}{T_{1}^{1}} = \frac{π}{3}, T_{1}^{1} = 6 sec$
$θ_{2}^{1} = \frac{5 π / 3}{1}, \frac{2 π}{T_{2}^{1}} = \frac{5 π}{3}, T_{2}^{1} = 1.2 sec$

Updated on:21/07/2023

A particle performing S.H.M undergoes displacement of $A / 2$ (where A = amplitude of S.H.M.) in one second. At $t = 0$ be the particle was located at either position or mean position. The time period of S.H.M. can be : (consider all possible cases)
A12s
B2.4
C6s
D1.2s
The particle performing S.H.M., about mean position it has
Amaximum acceleration and maximum velocity
Bminimum acceleration and maximum velocity
Cmaximum acceleration and minimum velocity
Dminimum acceleration and minimum velocity
A particle performing S.H.M., its velocity when the particle moves from mean to extreme position is
Aslower initially and faster laterally
Buniformly moves
Cfaster initially and momentraily falls to zero
Dfast moves and stop at extreme position