Doubtnut Promotions Sticky

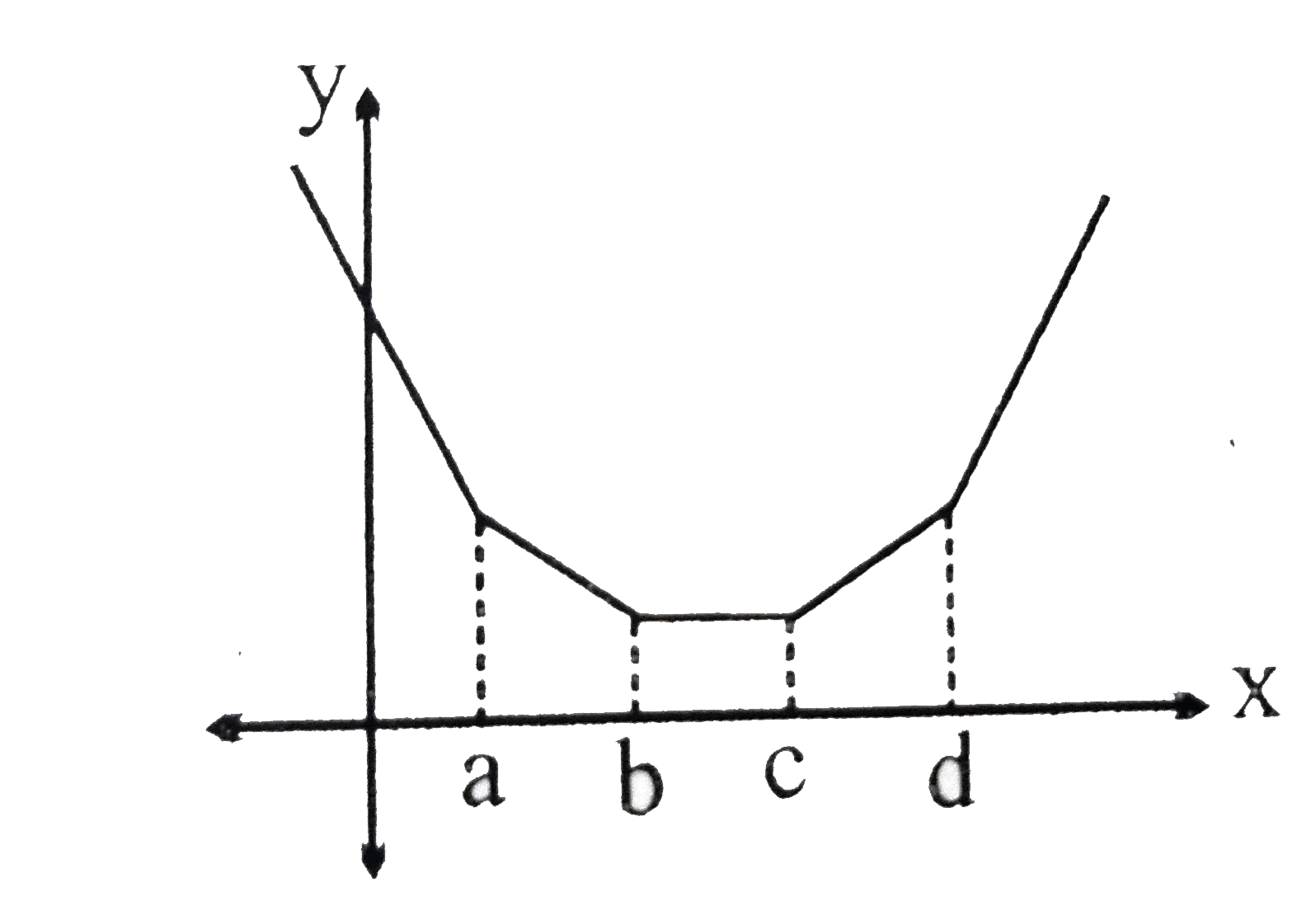
Let a,b,c,d ∈ N and a<b<c<d such that the equation |x−a|+|x−b|+|x−c|+|x−d|=20−2(a+b) has infinite solutions then find the number of possible quadruplets (a,b,c,d).

Text Solution
Verified by Experts

552
f(b)=f( c)=d+c-a-b=20-2(a+b)
∴a+b+c+d=20
Let a=x,b=a+y,c=b+z, d=c+w
∴ 4x+3y+2z+w=20
As 23×4≠552.
ocr_image

Doubtnut Promotions Banner
|
Updated on:21/07/2023
Class 12MATHSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One or More

    If a<b<c<d, then the quadratic equation (x−a)(x−c)+2(x−b)(x−d)=0 has its roots

    Areal
    Bimaginary
    Cone root in (a,c)
    Dboth roots in (a,c)
  • Question 2 - Select One

    If a<b<c<d, then the roots of the equation (x-a)(x-c)+2(x-b)(x-d)=0 are

    Areal and distinct
    Breal and equal
    Cimaginary
    DNone of the above
  • Question 3 - Select One

    If a<b<c<d, then the eqution 3(x−a)(x−c)+5(x−b)(x−d)=0 has

    Areal and distinct roots
    Breal and equal roots
    Cpurely imaginary roots
    Dnone of these