Three capacitors of capacitance are connected in parallel. Determine the cahrge on each capacitor, if the combination is connected to a 100 V supply?
To solve the problem of finding the charge on each capacitor connected in parallel, we can follow these steps:
1. Identify the Given Values:
- Capacitor 1 (C1) = 2 pF = 2×10−12 F
- Capacitor 2 (C2) = 3 pF = 3×10−12 F
- Capacitor 3 (C3) = 4 pF = 4×10−12 F
- Voltage (V) = 100 V
2. Understand the Parallel Connection:
- In a parallel connection, the voltage across each capacitor is the same. Therefore, the voltage across each capacitor is 100 V.
3. Use the Formula for Charge:
- The charge (Q) on a capacitor can be calculated using the formula:
Q=C×V
- Where:
- Q is the charge in coulombs,
- C is the capacitance in farads,
- V is the voltage in volts.
4. Calculate the Charge on Each Capacitor:
- For Capacitor 1 (C1):
Q1=C1×V=(2×10−12F)×(100V)=2×10−10C
- For Capacitor 2 (C2):
Q2=C2×V=(3×10−12F)×(100V)=3×10−10C
- For Capacitor 3 (C3):
Q3=C3×V=(4×10−12F)×(100V)=4×10−10C
5. Summarize the Charges:
- Charge on Capacitor 1 (Q1) = 2×10−10 C
- Charge on Capacitor 2 (Q2) = 3×10−10 C
- Charge on Capacitor 3 (Q3) = 4×10−10 C
Final Results:
- Q1 = 2 × 10^(-10) C
- Q2 = 3 × 10^(-10) C
- Q3 = 4 × 10^(-10) C
- Capacitor 1 (C1) = 2 pF = 2×10−12 F
- Capacitor 2 (C2) = 3 pF = 3×10−12 F
- Capacitor 3 (C3) = 4 pF = 4×10−12 F
- Voltage (V) = 100 V
- In a parallel connection, the voltage across each capacitor is the same. Therefore, the voltage across each capacitor is 100 V.
- The charge (Q) on a capacitor can be calculated using the formula:
Q=C×V
- Where:
- Q is the charge in coulombs,
- C is the capacitance in farads,
- V is the voltage in volts.
- For Capacitor 1 (C1):
Q1=C1×V=(2×10−12F)×(100V)=2×10−10C
Q2=C2×V=(3×10−12F)×(100V)=3×10−10C
Q3=C3×V=(4×10−12F)×(100V)=4×10−10C
- Charge on Capacitor 1 (Q1) = 2×10−10 C
- Charge on Capacitor 2 (Q2) = 3×10−10 C
- Charge on Capacitor 3 (Q3) = 4×10−10 C
- Q1 = 2 × 10^(-10) C
- Q2 = 3 × 10^(-10) C
- Q3 = 4 × 10^(-10) C
Topper's Solved these Questions
- BOOK - MBD -HARYANA BOARDCHAPTER - ELECTROSTATICSEXERCISE - LONG ANSWER TYPE QUESTIONS14videos
ELECTROSTATICS
- BOOK - MBD -HARYANA BOARDCHAPTER - ELECTROSTATICSEXERCISE - OBJECTIVE TYPE QUESTIONS23videos
ELECTROSTATICS
- BOOK - MBD -HARYANA BOARDCHAPTER - ELECTROSTATICSEXERCISE - OBJECTIVE TYPE QUESTIONS23videos
ELECTROSTATICS
- BOOK - MBD -HARYANA BOARDCHAPTER - Electromagnetic WavesEXERCISE - Example7videos
Electromagnetic Waves
- BOOK - MBD -HARYANA BOARDCHAPTER - MAGNETISMEXERCISE - OBJECTIVE TYPE QUESTIONS17videos
MAGNETISM
Similar Questions
Three capacitors of capacitances 2pF,3pFand4pF are connected in parallel. Determine the charge on each capacitor, if the combination is connected to a 100 V suppy ?
View SolutionThree capacitors of capacitance 2pF,3pFand4pF are connected in parallel. (a) what is the total capacitance of the combination ? (b) Determine the charge on each capacitor, If the combination is connected to 100V supply.
View Solution
Knowledge Check
- Question 1 - Select One
Four condensers having capacities 2pF, 3pF, 4pF and 6pF are connected in series. The equivalent capacity of the combination is
A8.0 pFB0.8 pFC1.8 pFD0.4 pF - Question 2 - Select One
What is the total capacitance if three capacitors of capacitance 2 pF, 3 pF and 4 pF are connected in parallel?
A9pFB1213pFC1312pFD294pF - Question 3 - Select One
In the figure, three capacitors each of capacitance 6pF are connected in series. The total capacitance of the combination will be
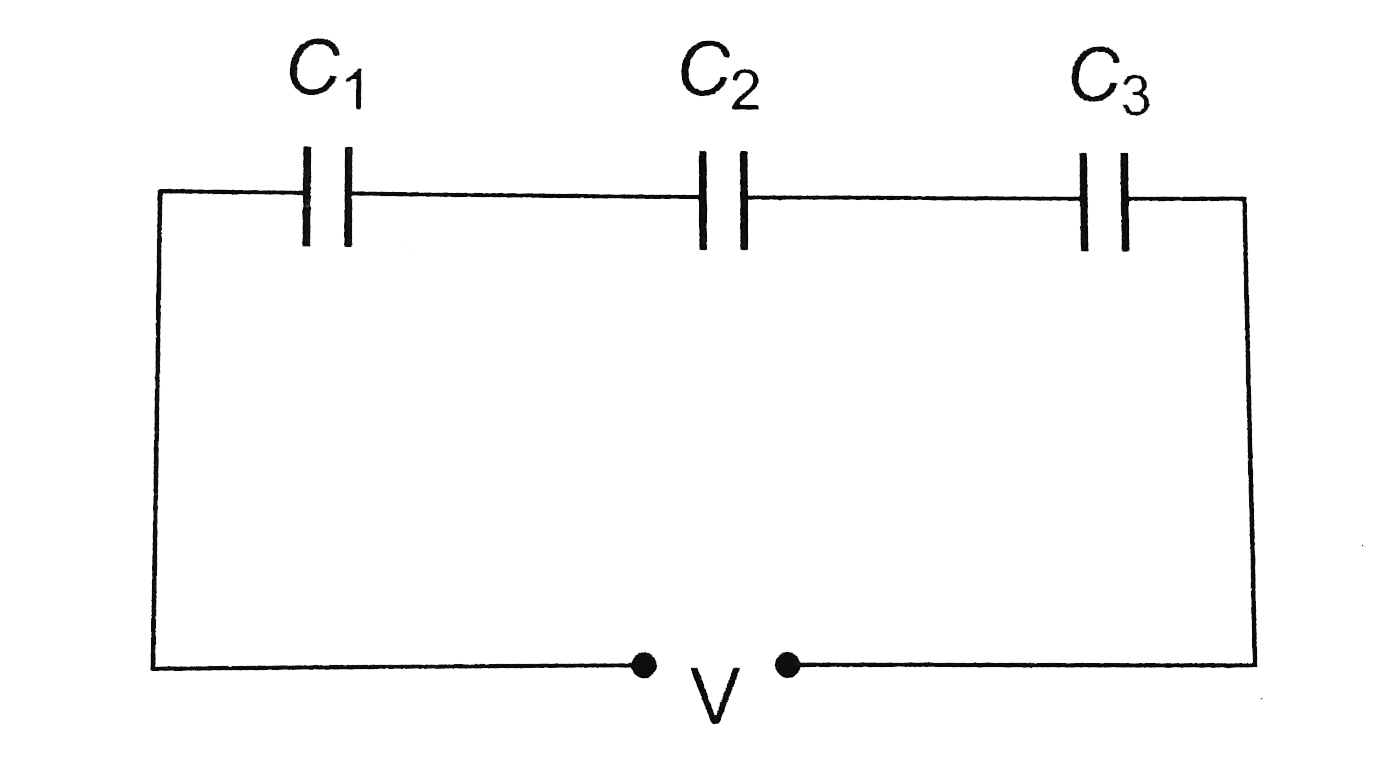 A9×10−12FB6×10−12FC3×10−12FD2×10−12F
A9×10−12FB6×10−12FC3×10−12FD2×10−12F
Three capacitors of capacitances 2 pF, 3 pF and 4 pF are connected in parallel.
(a) What is the total capacitance of the combination ?
(b) Determine the charge on each capacitor if the combination is connected to a 100 V supply.View SolutionThree capacitors each of capacitance 9 pF are connected in series.
(a) What is the total capacitance of the combination ?
(b) What is the potential difference across each capacitor if the combination is connected to a 120 V supply ?View SolutionThree capacitors each of capacitance 1μF are connected in parallel.To this combination,a fourth capacitor of capacitance 1μFis connected in series.The resultant capacitance of the system
View SolutionA network of four capacitors of 6 μF each is connected to a 240 V supply. Determine the charge on each capacitor.
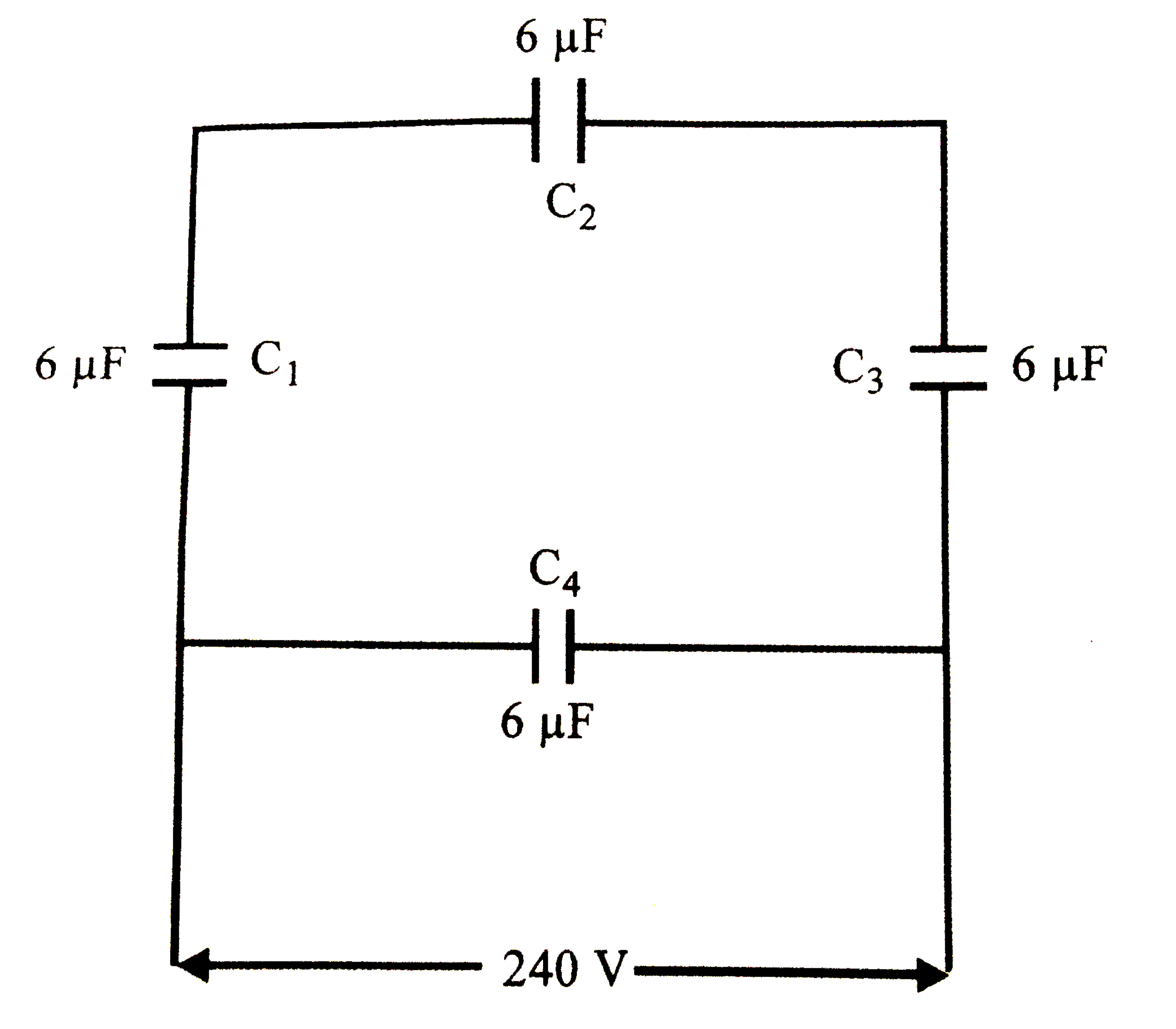 View Solution
View SolutionThree capacitors each of capacitance 1μF are connected in parallel. To this combination, a fourth capacitor capacitance 1μF is connected in series. The resultant capacitance of the system is
View Solution

