If P = $8 x^{4} + 6 x^{3} - 15 x^{2} + 27 x - 20$ and Q = $2 x^{2} + 3 x - 4$ , then find the remainder when P is divided by Q .

A

0

B

$- 1$

C

$- 8$

D

$- 4$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

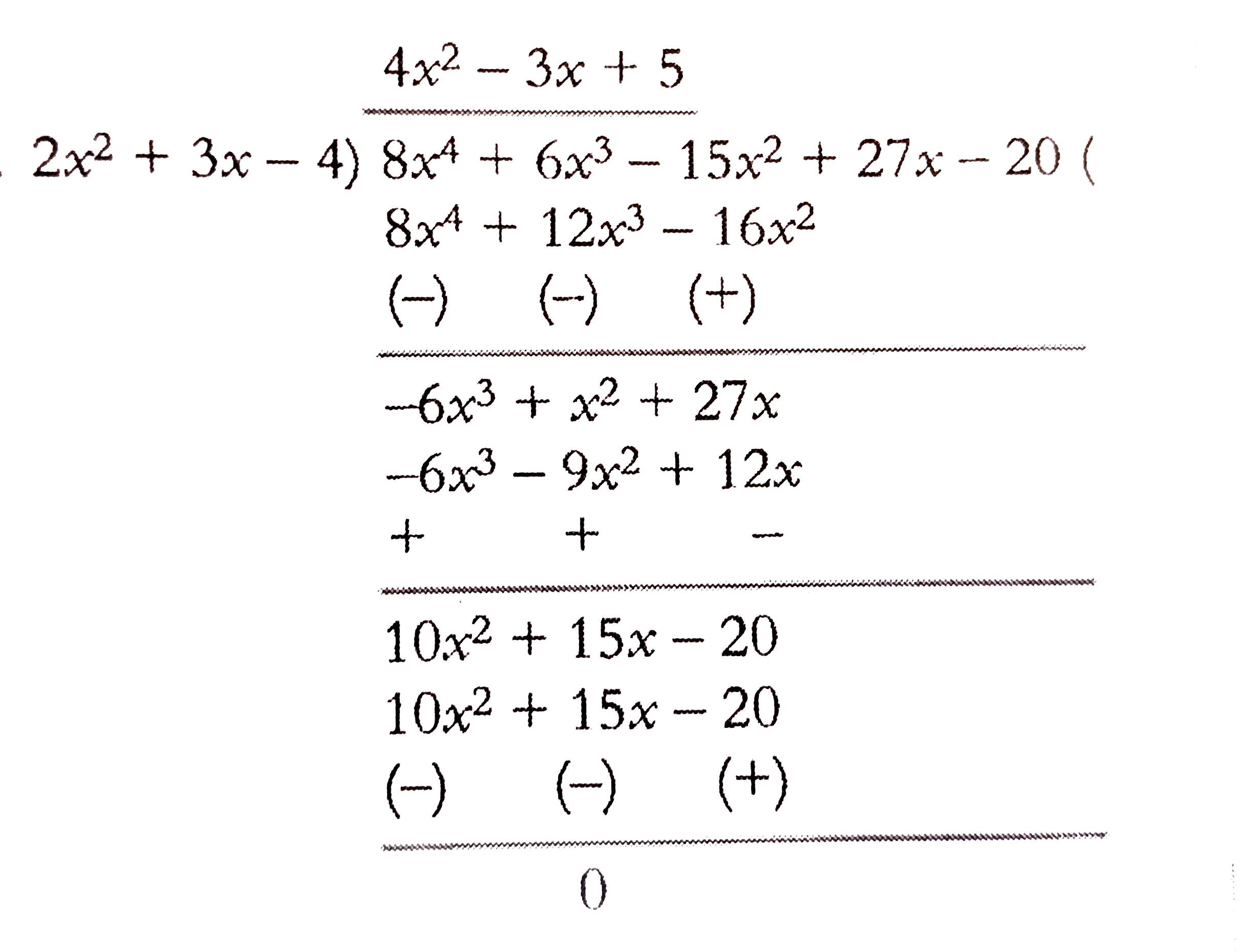
$∴$ The remainder = 0 .
Hence , the correct option is (a) .

|

Updated on:21/07/2023

Class 7 MATHS EXPRESSIONS AND SPECIAL PRODUCTS

Topper's Solved these Questions

BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - EXPRESSIONS AND SPECIAL PRODUCTS EXERCISE - Assessment Tests (Test 1)
36videos
EXPRESSIONS AND SPECIAL PRODUCTS
BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - EXPRESSIONS AND SPECIAL PRODUCTS EXERCISE - Assessment Tests (Test 2)
12videos
EXPRESSIONS AND SPECIAL PRODUCTS
BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - EXPRESSIONS AND SPECIAL PRODUCTS EXERCISE - Level 2
19videos
EXPRESSIONS AND SPECIAL PRODUCTS
BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - EQUATIONS AND THEIR APPLICATIONSEXERCISE - Level 3
23videos
EQUATIONS AND THEIR APPLICATIONS
BOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - FORMULAEEXERCISE - TEST 2
11videos
FORMULAE

Similar Questions

Knowledge Check

Question 1 - Select One
If P = $8 x^{4} + 6 x^{3} - 15 x^{2} + 27 x - 20$ and Q = $2 x^{2} + 3 x - 4$ , then find the remainder when P is divided by Q .
A0
B $- 1$
C $- 8$
D $- 4$
Question 2 - Select One
If $x^{4} - 8 x^{3} + x^{2} + 3 x - 6$ is divided by $(x + 2),$ then find the remainder.
A45
B50
C48
D72
Question 3 - Select One
If $x^{4} - 8 x^{3} + x^{2} + 3 x - 6$ is divided by (x+2), then find the remainder.
A45
B50
C48
D72

PEARSON IIT JEE FOUNDATION-EXPRESSIONS AND SPECIAL PRODUCTS -Level 3

Home
Ask Doubt
Profile