Consider the differential equation dydx=yx+logx
i. Find the integrating factor of the differential equation
ii. Evaluate ∫1xlogxdx
iii. Hence find the general solution of the given differential equation.Solution in Tamil
Step by step video & image solution for Consider the differential equation (dy)/(dx)=(y)/(x)+logx i. Find the integrating factor of the differential equation ii. Evaluate int(1)/(x)logxdx iii. Hence find the general solution of the given differential equation. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DIFFERENTIAL EQUATIONSBOOK - NEW JOYTHI PUBLICATIONCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - CONTINUOUS EVALUATION (ASSIGNMENT)
1 Videos
- DIFFERENTIAL EQUATIONSBOOK - NEW JOYTHI PUBLICATIONCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - OBJECTIVE TYPE QUESTION
19 Videos
- DIFFERENTIAL EQUATIONSBOOK - NEW JOYTHI PUBLICATIONCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - NCERT MISCELLANEOUS EXERCISE
22 Videos
- CONIC SECTIONS BOOK - NEW JOYTHI PUBLICATIONCHAPTER - CONIC SECTIONS EXERCISE - EXERCISE - HYPERBOLA
9 Videos
- INTEGRALS BOOK - NEW JOYTHI PUBLICATIONCHAPTER - INTEGRALS EXERCISE - OBJECTIVE TYPE QUESTIONS
34 Videos
Similar Questions
Complete the following tables
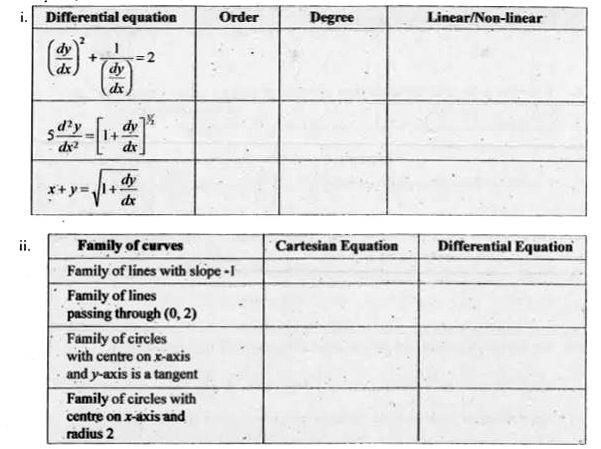
View SolutionThe degree of the differential equation [5+(dydx)2]53=x5(d2ydx2) is
View SolutionFor each of the differential equations given below, indicate its order and degree ( if defined ).
d2ydx2+5y(dydx)2−6y=logx
View SolutionThe vertices of the hyperbola 9x2−16y2=144
View Solution∫ealogxdx is equal to
View SolutionFind the general solution of the differential equations dydx=sin−1x
View SolutionFind the general solution of the differential equations:(x+y)dxdy=1
View SolutionFind the general solution of the differential equation dydx−y=cosx
View SolutionFind the general solution of the differential equation dydx=log(x+1).
View SolutionFind the general solution of the differential equation
dydx=sin−1x.
View SolutionFind the general solution of the differential equation (x+y)dydx=1.
View SolutionConsider the differential equation xcos(yx)dydx=ycos(yx)+x
i. Show that the differential equation is homogeneous.
ii. Hence find the gerenal solution
View SolutionFind the general solution of the differential equations dydx=sin−1x
View SolutionFind the general solution of the differential equations:(x+y)dxdy=1
View SolutionFind the general solution of the differential equation (x+y)dydx=1
View Solution
