A disc of mass 500 g and radius 10 cm can freely rotate about a fixed axis as shown in figure. Light and inextensible string is wound several turns around it and 100 g body is suspended at its free end. Find the acceleration of this mass. [Given: The string makes the disc to rotate and does not slip over it.
![A disc of mass 500 g and radius 10 cm can freely rotate about a fixed axis as shown in figure. Light and inextensible string is wound several turns around it and 100 g body is suspended at its free end. Find the acceleration of this mass. [Given: The string makes the disc to rotate and does not slip over it. g -10ms^(-2)]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/FM_PHY_XI_V01_C05_SLV_018_Q01.png)
Let the mass of the dise be m and its radius R. The mass of the suspended body is my
As the light inextensible string is wound around the disc several times it makes the disc rotate without slipping over it. The translational acceleration of m, and tangential acceleration of m, will be the same. Let us draw the free body diagram (FBD) of m, and m, separately.
FBD of the dise: Its gravitational force acts downward and normal force N exerted by the fixed support at the center acts upward. The tension Tacts downward at the edge. The gravitational force and the normal force (N) cancel each other. W.8=N The tension T produces a torque (RT), which produces a rotational motion in the disc with angular acceleration, . Hene, a is the linear acceleration of a point at the edge of the disc. If the moment of inertia of the disc is I and its radius of gyration is K, then

FBD of the body: Its gravitational force acts downward and the tension Tacts upward. As there is a resultant force acting me on it downward.

Substituting for T from the equation for disc,
The expression for a disc rotating about an axis passing through the center and perpendicular to the plane is, Ref Pg. No. 244-245 Table 5.3) Now the expression for acceleration further simplifies as
Substituting the values
Topper's Solved these Questions
- BOOK - FULL MARKSCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIESEXERCISE - TEXTUAL QUESTIONS SOLVED ( MULTIPLE CHOICE QUESTIONS:)15videos
MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES
- BOOK - FULL MARKSCHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODIESEXERCISE - TEXTUAL QUESTIONS SOLVED ( SHORT ANSWER QUESTIOS)17videos
MOTION OF SYSTEM OF PARTICLES AND RIGID BODIES
- BOOK - FULL MARKSCHAPTER - LAW OF MOTION EXERCISE - ADDITIONAL QUESTIONS SOLVED (SHORT ANSWER QUESTIONS (3 MARKS & NUMERICALS))16videos
LAW OF MOTION
- BOOK - FULL MARKSCHAPTER - NATURE OF PHYSICAL WORLD AND MEASUREMENT EXERCISE - ADDITIONAL QUESTIONS SOLVED ( SHORT ANSWER QUESTIONS (2 MARK))20videos
NATURE OF PHYSICAL WORLD AND MEASUREMENT
Similar Questions
A disc of mass m and radius r is free to rotate about its centre as shown in the figure. A string is wrapped over its rim and a block of mass m is attached to the free end of the string. The system is released from rest. The speed of the block as it descends through a height h, is :-
 View Solution
View SolutionA disc of mass m and radius r is free to rotate about its centre as shown in the figure. A string is wrapped over its rim and a block of mass m is attached to the free end of the string. The system is released from rest. The speed of the block as it descends through a height h, is :-
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A disc of mass 1 kg and radius 10 cm is rotating about its axis with an anuglar velocity of 2 rad/s. The linear momentum of the disc,
A0.2 kg-m/sBzeroC0.4 kg-m/sD0.02 kg-m/s - Question 2 - Select One
A block of mass m is attached at the end of an inextensible string which is wound over a rough pulley of mass M and radius R. Assume the string does not slide over the pulley. Find the acceleration of the block when released.
Amg2m+MB2mgm+MCmgm+MD2mg2m+M - Question 3 - Select One
A wheel of radius 0.4m can rotate freely about its axis as shown in the figure. A string is wrapped over its rim and a mass of 4kg is hung. An angular acceleration of 8rad/s2 is produced in it due to the torque. Then, the moment of inertia of the wheel is (g=10m/s2)
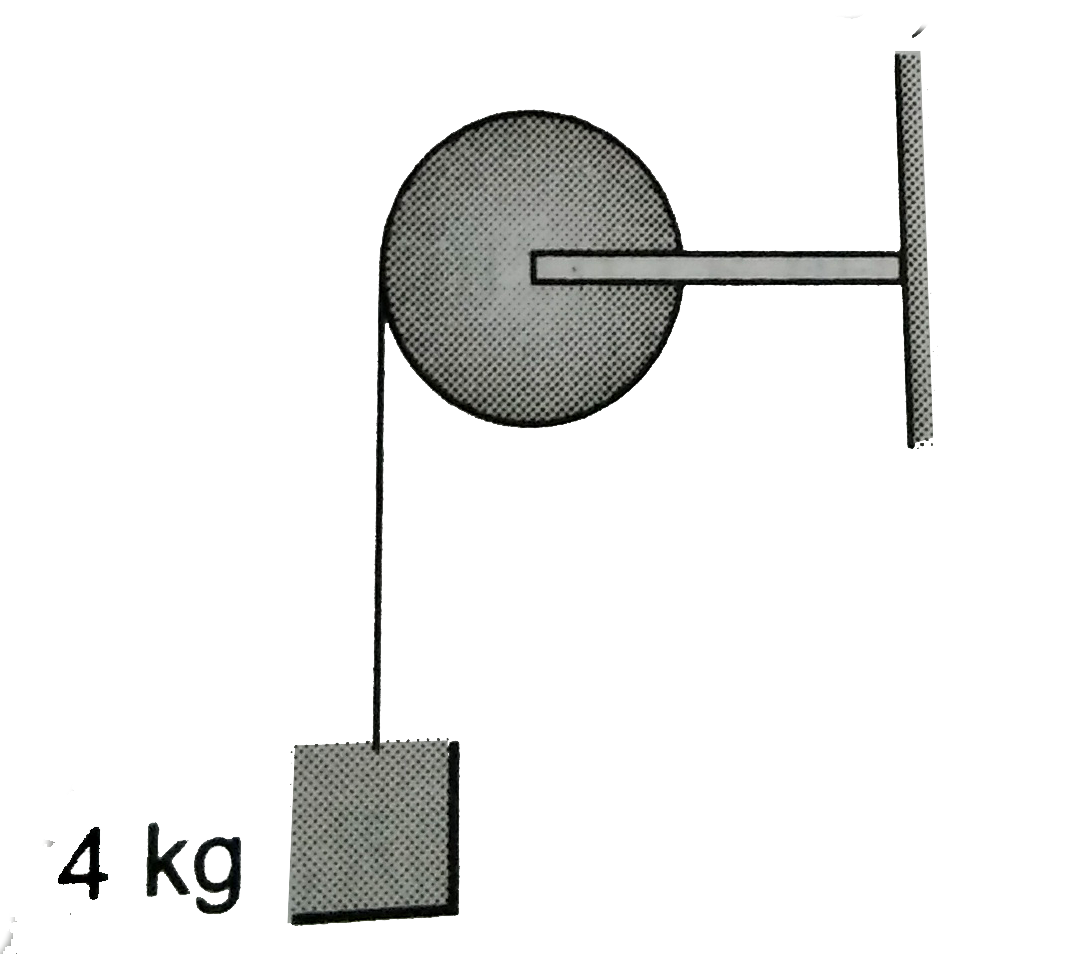 A2kg−m2B1.36kg−m2C4kg−m2D8kg−m2
A2kg−m2B1.36kg−m2C4kg−m2D8kg−m2
A circular disc of mass 100 g and radius 10 cm' is making 2 rps about an axis passing through its centre and perpendicular to its plane. Calculate its kinetic energy.
View SolutionIn the figure A and B are two blocks of mass 4kg and 2kg respectively attached to the two ends of light string passing overa disc C of mass 40kg and radius 0.1m. The disc is free to rotate about a fixed horizontal the string does not string does not slip over the disc. Find.
(i) The linear acceleration of mass B.
(ii) the number of revolutions made by the disc at the end of 10sec. from the start.
(iii) the tension in teh string segment supporting the block A.
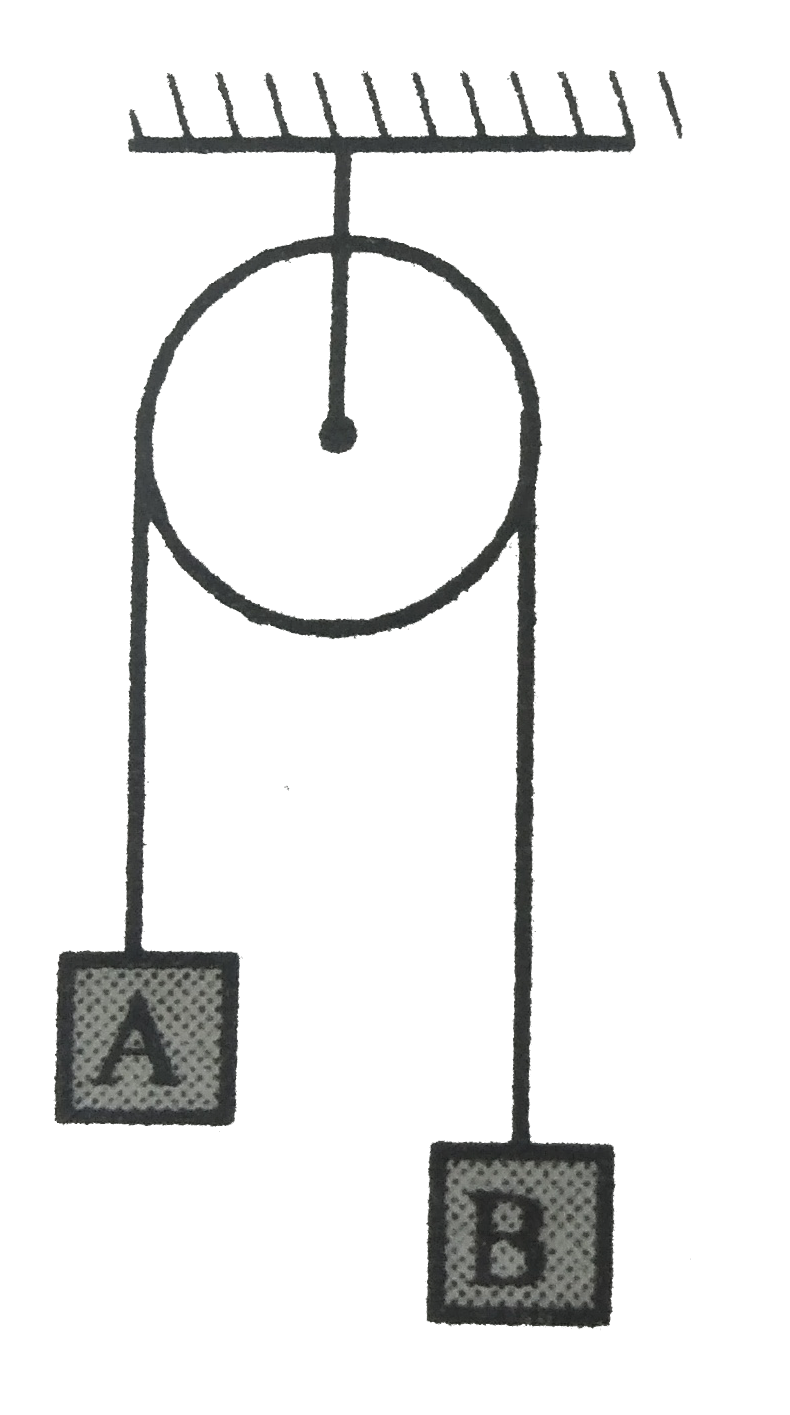 .View Solution
.View SolutionA block of mass m is attached at the end of an inextensible string which is wound over a rough pulley of mass M and radius R figure a. Assume the string does not slide over the pulley. Find the acceleration of the block when released.
View SolutionA wheel of radius 0.4m can rotate freely about its axis as shown in the figure. A string is wrapped over its rim and a mass of 4kg is hung. An angular acceleration of 8rad/s2 is produced in it due to the torque. Then, the moment of inertia of the wheel is (g=10m/s2)
 View Solution
View SolutionA uniform disc of radius R and mass M is free to rotate only about its axis. A string is wrapped over its rim and a body of mass m is tied to the free end of the string as shown in the figure. The body is released from rest. Then the acceleration of the body is
 View Solution
View Solution
