What is the magnetic field along the axis and equatorial line of a bar magnet ? Solution in Tamil

Video Solution

Text Solution

Verified by Experts

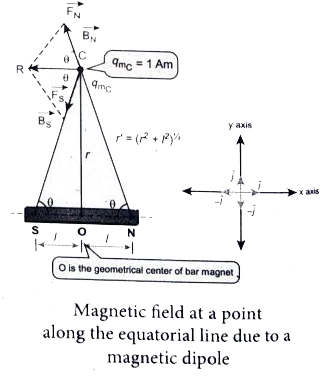
Consider a bar magnet NS. Let N be the north pole and S be the south pole of the bar magnet, each with pole strength qm and separated by a distance of 21. The magnetic field at a point C (lies along the equatorial line) at a distance r from the geometrical center O of the bar magnet can be computed by keeping unit north pole ( $q_{m} C$ = 1 A m) at C. The force experienced by the unit north pole at C due to pole strength N-S can be computed using Coulomb’s law of magnetism as follows:
The force of repulsion between North Pole of the bar magnet and unit north pole at point C (in free space) is
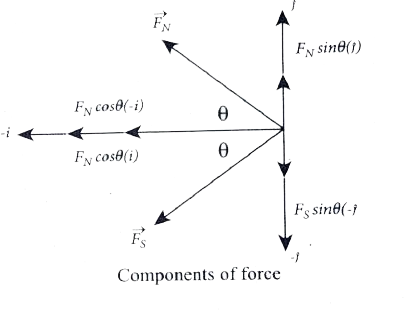
${\to F}_{N} = - F_{N} cos θ ˆ i + F_{N} sin θ ˆ j$ ...(1)
Where $F_{N} = \frac{μ_{0} q_{m}}{4 π r .^{2}}$
The force of attraction (in free space) between south pole of the bar magnet and unit norm pole at point C is
${\to F}_{s} = - F_{S} cos θ ˆ i - F_{s} sin θ j$
Where ${\to F}_{S} = \frac{μ_{0} q_{m}}{4 π r .^{2}}$
From equation (1) and equation (2), the net force at point C is $\to F = {\to F}_{N} + F_{s}$ . This net force is equal to the magnetic field at the point C.
$\to B = - (F_{N} + F_{S}) cos θ ˆ i$
Since $F_{N} = F_{S}$
$\to B = \frac{2 μ_{0}}{4 π} \frac{q_{m}}{(r^{2} + l^{2})} cos θ ˆ i$ ...(3)
In a right angle triangle NOC as shown in the Figure 1
$cos θ = \frac{adjacent}{hypotenuse} = \frac{1}{r .} = \frac{1}{{(r^{2} + l^{2})}^{\frac{1}{2}}}$ ...(4)
Substituting equation 4 in equation 3 we get
$\to B = - \frac{μ_{0}}{4 π} \frac{Q_{M} \times (2 L)}{{(r^{2} + l^{2})}^{\frac{3}{2}}}$ ...(5)
Since, magnitude of magnetic dipole moment is $∣ ∣ {\to P}_{m} ∣ ∣ = P_{m} = q_{m} .2 l$ and substituting in equation (5), the magnetic field at a point C is
${\to B}_{equational} = \frac{μ_{0}}{4 π} \frac{P_{m}}{{(r^{2} + l^{2})}^{\frac{3}{2}}} ˆ i$ ...(6)
If the distance between two poles in a bar magnet are small (looks like short magnet) when compared to the distance between geometrical center O of bar magnet and the location of point C i.e., r>> I, then,
${(r^{2} + l^{2})}^{\frac{3}{2}} = r^{3}$ ...(7)
Therefore, using equation (7) in equation (6), we get
${\to B}_{equational} = \frac{μ_{0}}{4 π} \frac{P_{m}}{r^{3}} ˆ i$
Since $P_{m} ˆ i = {\to P}_{m}$ , in general, the magnetic field at equitorial point is given by
${\to B}_{equational} = \frac{μ_{0}}{4 π} \frac{P_{m}}{r^{3}}$ ...(8)
Note that magnitude of $B_{axial}$ is twice that of magnitude of $B_{equational}$ and the direction of $B_{axial}$ and $B_{equational}$ are opposite.

|

Updated on:21/07/2023

Class 12 PHYSICS SAMPLE PAPER -10 (SOLVED)

Topper's Solved these Questions

Similar Questions

FULL MARKS-SAMPLE PAPER -10 (SOLVED)-PART - IV

Home
Ask Doubt
Profile