Question

In a free fall the velocity of a stone is increasing equally ion equal intervals of time under the effect of gravitational force of the earth. Then what can you say about the motion of this stone? Whether the stone is having:

Options

A. -uniform acceleration
B. Non-uniform acceleration
C. retardation
D. constant speed

Answer

a

Explanation

An object is in motion with initial velocity u attains a final velocity v in time t due to acceleration a, with displacement s.
Let us try to derive these equations by graphical method. Equations of motion from velocity-time graph:

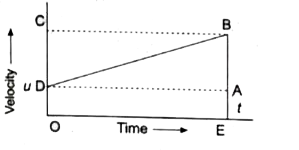
Graph shows the change in velocity with time for an uniformly accelerated object. The object starts from the point D in the graph with velocity u. Its velocity keeps increasing and after time t it reaches the point B on the graph .
The initial velocity of the object =u = OD = EA
The final velocity of the object =v=OC = EB
Time = t= OE = DA
Also from the graph we know that , AB=DC
For first equation of motion
By definition, acceleration =change in velocity / time
=(final velcity -initial velocity ) / time
=(OC-OD)/OE=DC/OE
a=DC/t
DC=AB=at
From the graph EB=EA+AB
v=u+at ....(1)
This is first equation of motion.
For second equation of motion
From the graph the distance covered by the object during time t is given by the area of quadrangle DOEB
s=area of the quad-rectangel DOEB
s=area of the rectangle DOEA + area of the triangle DAB
=(AE x OE) + (1/2 x AB x DA)
`s=ut+1//2 at^2`. ...(2)
This is second equation of motion.
For Third equation of motion
From the graph the distance covered by the object during time t is given by the area of the quadrangle DOEB . Here DOEB is a trapezium . Then
s=area of trapezium DOEB
=1/2 x sum of length of parallel side x distance between parallel sides
=1/2 x (OD +BE) x OE
s=1/2 x (u+v) x t
since a =(v-u)/t or t=(v-u)/a
Therefore s=1/2 x (v+u) x (v-u)/a
`2as=v^2-u^2`
`v^2=u^2+2as` ....(3)
This is third equation of motion.