Consider curve $y = f (x),$ then if PT = Length of tangent PN= Length of normal TM=Length of subtangent MN=Length of sub normal, then $T M \dot{M} N = f^{'} (x)$ (b) $\frac{P T}{P N} = \frac{1}{f^{'} (x)}$ $C T \dot{P} N = c o n s t a n t$ (d) $P T \dot{T} M = c o n s t a n t$

Video Solution

Answer

Step by step video & image solution for Consider curve y=f(x), then if PT = Length of tangent PN= Length of normal TM=Length of subtangent MN=Length of sub normal, then T MdotM N=f^(prime)(x) (b) (P T)/(P N)=1/(f^(prime)(x)) C TdotP N=con s t a n t (d) P TdotT M=con s t a n t by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Knowledge Check

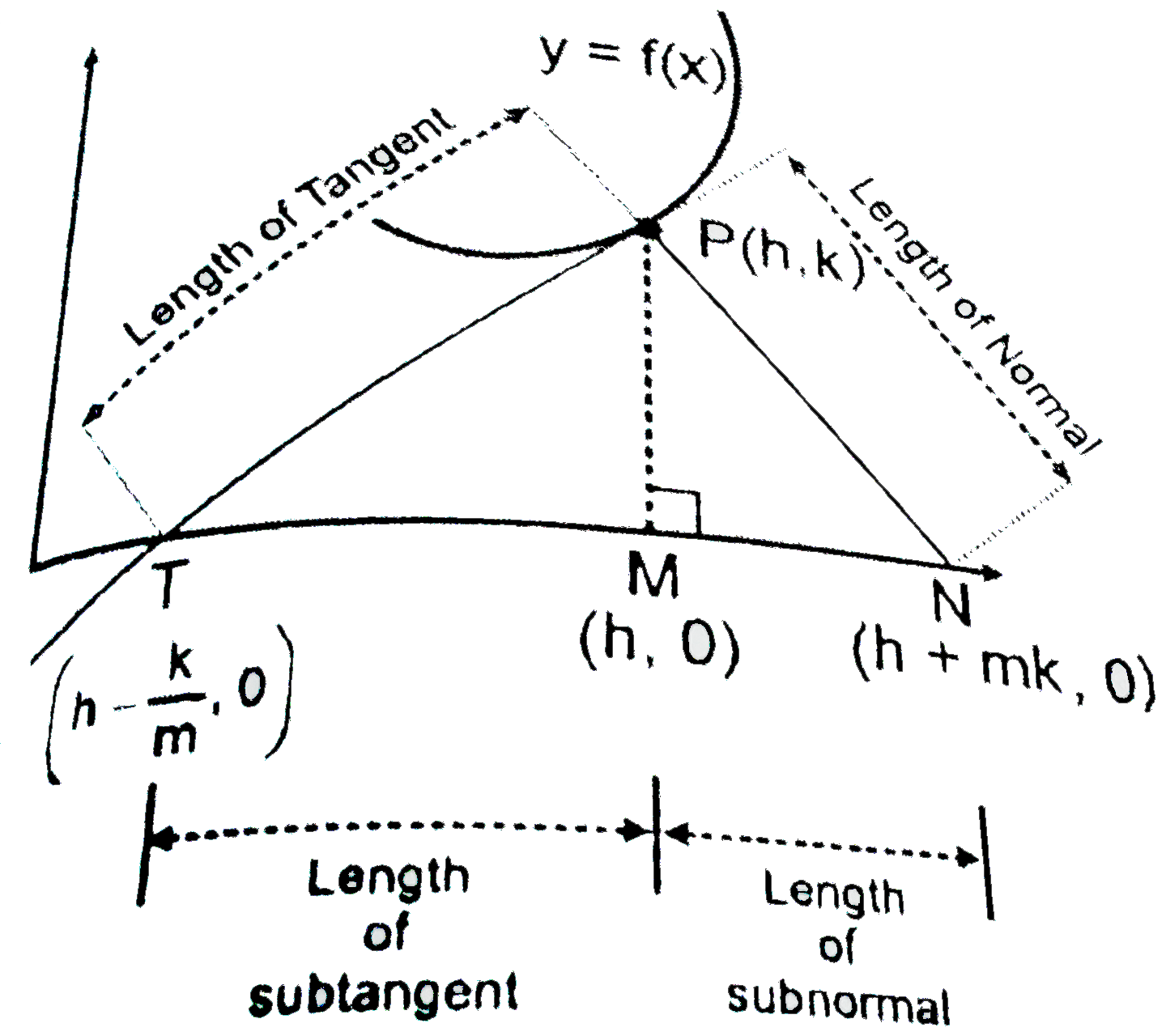
Let P (h,K) be any point on curve $y = f (x) .$ Let tangent drawn at point P meets x-axis at T & normal at point P meets x-axis at N (as shown in figure) and m $= \frac{d y}{d x}] (h, k))$ = shope of tangent.

(i) Length of tangent =PT $= | K | \sqrt{1 + \frac{1}{m^{2}}}$
(ii) Length of Normal =PN + $| K | \sqrt{1 + m^{2}}$
(iii) Length subtangent = Projection of segment PT on x-axis $= T M = ∣ ∣ ∣ \frac{k}{m} ∣ ∣ ∣$
(iv) Length of subnormal =Projection of line segment PN on x-axis =MN $= | K m |$
Find length of subnormal to $x = \sqrt{2} cos t, y = - 3 sin t at t = \frac{- π}{4} .$
A $\frac{2}{9}$
B $1$
C $\frac{7}{2}$
D $\frac{9}{2}$
The length of the normal at t on the curve $x = a (t + sin t), y = a (1 - cos t),$ is
A $a sin t$
B $2 a {sin}^{3} \frac{t}{2} sec \frac{t}{2}$
C $2 a sin \frac{t}{2} tan \frac{t}{2}$
D $2 a sin \frac{t}{2}$
The length of the subtangent to the ellipse $x = a cos t, y = b sin t$ at $t = π / 4$ is
Aa
Bb
C $b / \sqrt{2}$
D $a / \sqrt{2}$

RESONANCE DPP-JEE MAINS-All Questions

Home
Ask Doubt
Profile

Consider curve y = f ( x ) , then if PT = Length of tangent PN= Length of normal TM=Length of subtangent MN=Length of sub normal, then T M M . N = f ′ ( x ) (b) P T P N = 1 f ′ ( x ) C T P . N = c o n s t a n t (d) P T T . M = c o n s t a n t

Topper's Solved these Questions

Similar Questions

Knowledge Check

The length of the normal at t on the curve x=a(t+sint),y=a(1−cost), is

The length of the subtangent to the ellipse x=acost,y=bsint at t=π/4 is

RESONANCE DPP-JEE MAINS-All Questions

Consider curve $y = f (x),$ then if PT = Length of tangent PN= Length of normal TM=Length of subtangent MN=Length of sub normal, then $T M \dot{M} N = f^{'} (x)$ (b) $\frac{P T}{P N} = \frac{1}{f^{'} (x)}$ $C T \dot{P} N = c o n s t a n t$ (d) $P T \dot{T} M = c o n s t a n t$

The length of the normal at t on the curve $x = a (t + sin t), y = a (1 - cos t),$ is

The length of the subtangent to the ellipse $x = a cos t, y = b sin t$ at $t = π / 4$ is