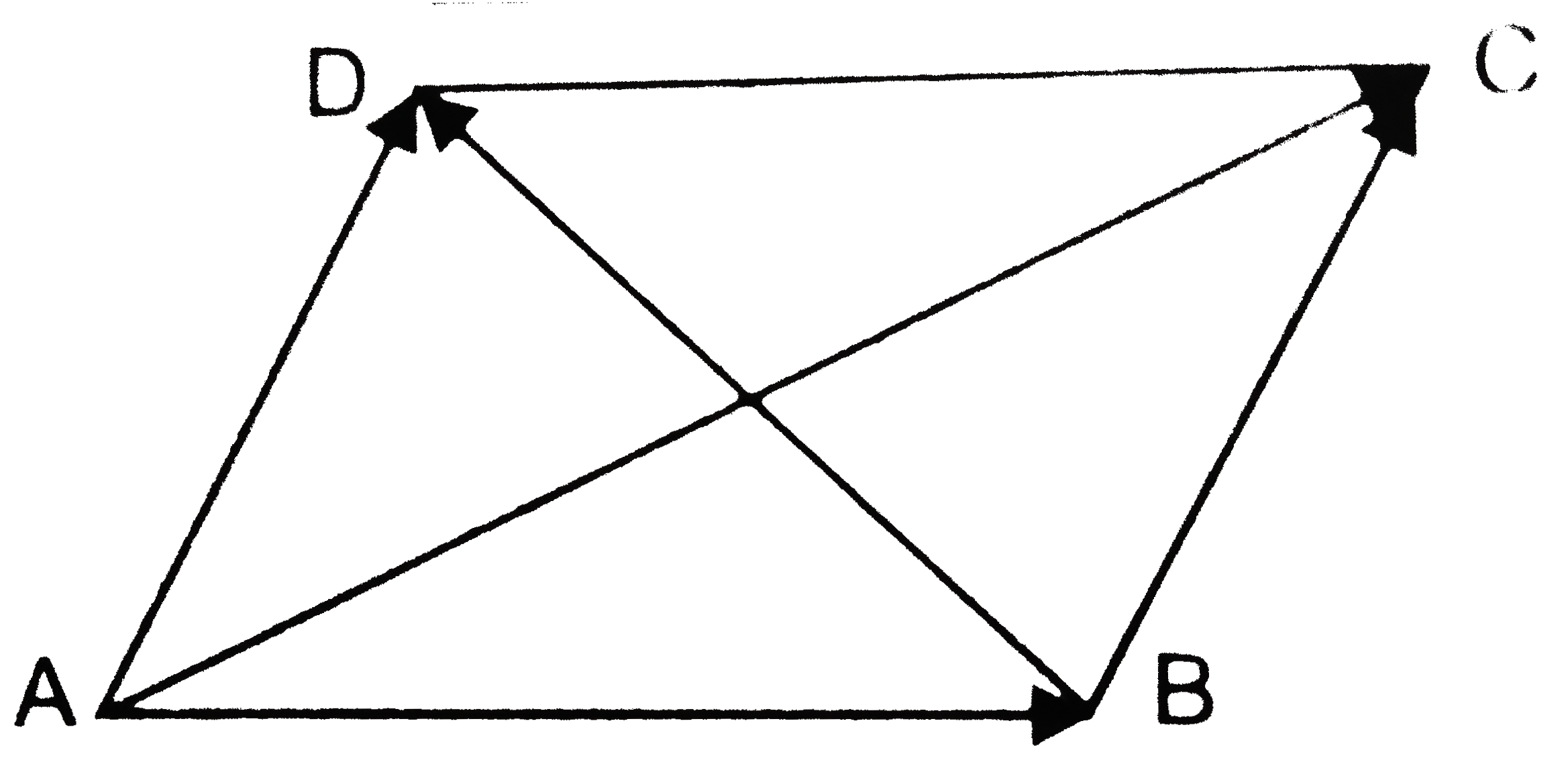
PQRS is a parallelogram and $- - \to Q S$ and $- - \to P R$ are its diagonals .Show that
$- - \to P R + - - \to Q S - 2 - - \to Q R = 0$

Video Solution

Text Solution

Generated By DoubtnutGPT

To prove that $\to P R + \to Q S - 2 \to Q R = 0$ for the parallelogram PQRS, we will follow these steps:
Step 1: Understand the vectors in the parallelogram
In a parallelogram PQRS, we have:
- $\to P Q$ is one side,
- $\to Q R$ is the adjacent side,
- $\to P R$ is the diagonal from point P to point R,
- $\to Q S$ is the diagonal from point Q to point S.
Step 2: Express the diagonals in terms of sides
Using vector addition, we can express the diagonals $\to P R$ and $\to Q S$ in terms of the sides:
- The diagonal $\to P R$ can be expressed as:
$\to P R = \to P Q + \to Q R$
- The diagonal $\to Q S$ can be expressed as:
$\to Q S = \to Q R + \to R S$
Since $\to R S = - \to P Q$ (opposite sides of a parallelogram are equal and opposite), we can rewrite $\to Q S$ as:
$\to Q S = \to Q R - \to P Q$
Step 3: Substitute the expressions into the equation
Now, we substitute the expressions for $\to P R$ and $\to Q S$ into the equation $\to P R + \to Q S - 2 \to Q R$ :
$\to P R + \to Q S - 2 \to Q R = (\to P Q + \to Q R) + (\to Q R - \to P Q) - 2 \to Q R$
Step 4: Simplify the equation
Now, we simplify the expression:
$= \to P Q + \to Q R + \to Q R - \to P Q - 2 \to Q R$
$= \to P Q - \to P Q + \to Q R + \to Q R - 2 \to Q R$
$= 0$
Conclusion
Thus, we have shown that:
$\to P R + \to Q S - 2 \to Q R = 0$

Updated on:18/9/2024