A magnetic field in a region of space is given by →B=2ˆi+3ˆj−ˆk tesla. A straight wire of length Z carrying current I is placed along the Z-axis. Find the angle made by net magnetic force with the positive X-axis.
To solve the problem step by step, we will follow the physics principles related to magnetic forces on current-carrying conductors.
Step 1: Identify the Given Information
We have the magnetic field vector:
→B=2^i+3^j−^k tesla
We also have a straight wire of length L carrying current I placed along the Z-axis. The length vector of the wire can be represented as:
→L=L^k
Step 2: Use the Formula for Magnetic Force
The force →F on a current-carrying conductor in a magnetic field is given by the formula:
→F=I→L×→B
Step 3: Calculate the Cross Product
We need to calculate →L×→B:
→L×→B=(L^k)×(2^i+3^j−^k)
Using the distributive property of the cross product, we can expand this:
→L×→B=L^k×(2^i)+L^k×(3^j)+L^k×(−^k)
Calculating each term:
1. ^k×^i=^j (using the right-hand rule)
2. ^k×^j=−^i
3. ^k×^k=0
Thus:
→L×→B=L(2^j−3^i+0)=−3L^i+2L^j
Step 4: Write the Force Vector
Now substituting back into the force equation:
→F=I→L×→B=I(−3L^i+2L^j)=−3IL^i+2IL^j
Step 5: Determine the Components of the Force
The components of the force vector →F are:
- Fx=−3IL (in the negative x-direction)
- Fy=2IL (in the positive y-direction)
Step 6: Calculate the Angle with the Positive X-axis
To find the angle θ that the force makes with the positive x-axis, we can use the tangent function:
tan(θ)=FyFx=2IL−3IL=−23
Since Fx is negative and Fy is positive, the angle θ is in the second quadrant:
θ=180∘−tan−1(23)
Calculating tan−1(23):
tan−1(23)≈33.69∘
Thus,
θ=180∘−33.69∘≈146.31∘
Final Answer
The angle made by the net magnetic force with the positive x-axis is:
θ≈146.31∘
We have the magnetic field vector:
→B=2^i+3^j−^k tesla
We also have a straight wire of length L carrying current I placed along the Z-axis. The length vector of the wire can be represented as:
→L=L^k
The force →F on a current-carrying conductor in a magnetic field is given by the formula:
→F=I→L×→B
We need to calculate →L×→B:
→L×→B=(L^k)×(2^i+3^j−^k)
Using the distributive property of the cross product, we can expand this:
→L×→B=L^k×(2^i)+L^k×(3^j)+L^k×(−^k)
1. ^k×^i=^j (using the right-hand rule)
2. ^k×^j=−^i
3. ^k×^k=0
→L×→B=L(2^j−3^i+0)=−3L^i+2L^j
Now substituting back into the force equation:
→F=I→L×→B=I(−3L^i+2L^j)=−3IL^i+2IL^j
The components of the force vector →F are:
- Fx=−3IL (in the negative x-direction)
- Fy=2IL (in the positive y-direction)
To find the angle θ that the force makes with the positive x-axis, we can use the tangent function:
tan(θ)=FyFx=2IL−3IL=−23
Since Fx is negative and Fy is positive, the angle θ is in the second quadrant:
θ=180∘−tan−1(23)
tan−1(23)≈33.69∘
Thus,
θ=180∘−33.69∘≈146.31∘
The angle made by the net magnetic force with the positive x-axis is:
θ≈146.31∘
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - PRACTICE PROBLEMS
59 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Conceptual Questions
29 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
Similar Questions
Knowledge Check
A straight wire of finite length carrying current I subtends an angle of 60∘ at point P as shown. The magnetic field at P is
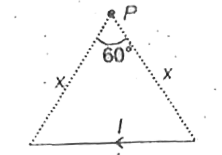 Aμ0I2√3πxBμ0I2πxC√3μ0I2πxDμ0I3√3πx
Aμ0I2√3πxBμ0I2πxC√3μ0I2πxDμ0I3√3πxThe angle made by the vector 3ˆi−4ˆj+5ˆk with the Z-axis is
Aπ4Bcos−1(1√2)C0Dcos−1(35√2)A uniform magnetic field existsin region given by →B=3ˆi+4ˆj+5ˆk. A rod of length 5m is placed along y-axis is moved along x- axis with constant speed 1m/sec. Then the magnitude of induced e.m.f in the rod is :
AzeroB25VC20 VD15 V
A long, straight wire carries a current along the z-axis. One can find two points in the x-y plane such that
View SolutionA magnetic field →B=(ˆi+0.5ˆj+0.25ˆk) T exists in a space. A wire of length →l=(0.25ˆi−0.25ˆj) m carries a current of 1 A and is placed in the magnetic field region.
Find the magnitude of magnetic force on the wire.
View SolutionA cylindrical region of magnetic field is produced by an electromagnet as shown in the figure. A wint carrying a current of0.5 A is placed perpendicularly to the axis of the cylindrical region. If the magnetic field produced by the electromagnets is 2.0 T in strength and the radius of cylindrical region is 5 cm, find the magnetic force acting on the wire due to the magnetic field in the region.

View SolutionA uniform magnetic field existsin region given by →B=3ˆi+4ˆj+5ˆk. A rod of length 5m is placed along y-axis is moved along x- axis with constant speed 1m/sec. Then the magnitude of induced e.m.f in the rod is :
View SolutionThe angle made by the vector 4ˆi−3ˆj+5ˆk with z-axis is :
View Solution

