The density of ice is 0.921 g cm−3. Calculate the mass of a cubic block of ice which is 76 mm on each side.
The correct Answer is:A, B, D
To calculate the mass of a cubic block of ice with a given density, we can follow these steps:
Step 1: Understand the given data
- Density of ice = 0.921 g/cm³
- Each side of the cube = 76 mm
Step 2: Convert the side length from mm to cm
To convert millimeters to centimeters, we divide by 10:
Side length in cm=76 mm10=7.6 cm
Step 3: Calculate the volume of the cube
The volume V of a cube is given by the formula:
V=side3
Substituting the side length:
V=(7.6 cm)3=7.6×7.6×7.6=438.976 cm3
We can round this to:
V≈439 cm3
Step 4: Use the density to find the mass
The mass m can be calculated using the formula:
m=density×volume
Substituting the values:
m=0.921 g/cm3×439 cm3
Calculating the mass:
m≈404.199 g
We can round this to:
m≈404.2 g
Final Answer
The mass of the cubic block of ice is approximately 404.2 grams.
---
- Density of ice = 0.921 g/cm³
- Each side of the cube = 76 mm
To convert millimeters to centimeters, we divide by 10:
Side length in cm=76 mm10=7.6 cm
The volume V of a cube is given by the formula:
V=side3
Substituting the side length:
V=(7.6 cm)3=7.6×7.6×7.6=438.976 cm3
We can round this to:
V≈439 cm3
The mass m can be calculated using the formula:
m=density×volume
Substituting the values:
m=0.921 g/cm3×439 cm3
Calculating the mass:
m≈404.199 g
We can round this to:
m≈404.2 g
The mass of the cubic block of ice is approximately 404.2 grams.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - SOME BASIC CONCEPTS OF CHEMISTRY EXERCISE - CONCEPTUAL QUESTION 112videos
SOME BASIC CONCEPTS OF CHEMISTRY
- BOOK - MODERN PUBLICATIONCHAPTER - SOME BASIC CONCEPTS OF CHEMISTRY EXERCISE - CONCEPTUAL QUESTION 214videos
SOME BASIC CONCEPTS OF CHEMISTRY
- BOOK - MODERN PUBLICATIONCHAPTER - SOME BASIC CONCEPTS OF CHEMISTRY EXERCISE - COMPETITION FILE (INTEGER TYPE AND NUMERICAL VALUE TYPE QUESTIONS)10videos
SOME BASIC CONCEPTS OF CHEMISTRY
- BOOK - MODERN PUBLICATIONCHAPTER - S-BLOCK ELEMENTS ( ALKALI AND ALKALINE EARTH METALS ) EXERCISE - UNIT PRACTICE TEST13videos
S-BLOCK ELEMENTS ( ALKALI AND ALKALINE EARTH METALS )
- BOOK - MODERN PUBLICATIONCHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - UNIT PRACTICE TEST13videos
STATES OF MATTER : GASES AND LIQUIDS
Similar Questions
Gaseous ozone is bubbled through water-ice mixture at 0∘C. As the O3(g) decomposes to form O2(g), the enthalpy of reaction is absorbed by the resulting ice. Given that the heat of fusion of ice is 6.0095 kJmol−1 , calculate the mass of ice that melts for each gram of O3 that decomposes.
2O3(g)→3O2(g),ΔH=−285.kJView SolutionThe density of ice is 0.918gcm−3 and that of water is 1.03gcm−3. An iceberg floats with a portion of 224m3 outside the surface of water. Find the total volume of the iceberg.
View Solution
Knowledge Check
- Question 1 - Select One
A cubical block of side 10cm floats at the interface of an oil and water as shown in the figure. The density of oil is 0.6gcm−3 and the lower face of ice cube is 2cm below the interface. The pressure above that of the atomosphere at the lower face of the block is
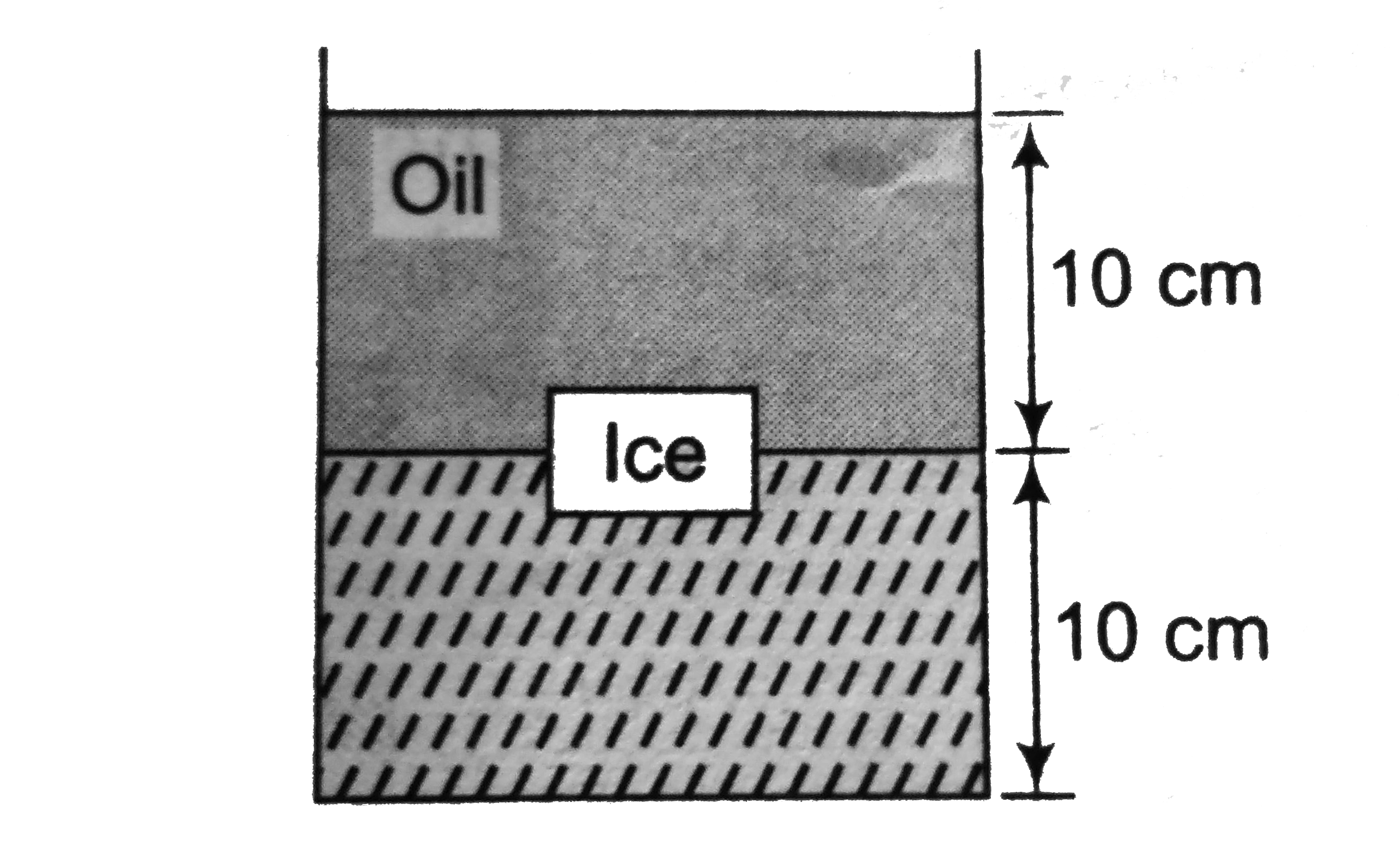 A200PaB620PaC900PaD800Pa
A200PaB620PaC900PaD800Pa - Question 2 - Select One
The density of ice is 0.92 g/cm3 and the density of seawater is 1.03 g/cm3. A large iceberg floats in Arctic waters. What fraction of the volume of the iceberg is exposed?
A0.080 %B11 %C0.89D0.92 - Question 3 - Select One
The density of ice xcm−3 and that of water is ygcm−3. What is the change in volume when mg of ice melts?
Am(y−x)cm3By−xmcm3Cmxy(x−y)cm3Dm((1y−1x)cm3.
Calculate the mass of a body whose volume is 2m3 and density 0.52gcm3.
View SolutionA hollow sphere of glass whose external and internal radii are 11cm and 9cm respec-tively is completely filled with ice at 0∘C and placed in a both of boiling water How long will it take for the ice to melt completely ? Givne that density of ice =0.9g/cm3 latent heat of fusion of ice =80cal/g and thermal conductivity of glass =0.002cal/cm−s∘C .
View SolutionAn ice layer of thickness 3 cm is formed on a lake. How much time will it take to increase the thickness of ice by 2 mm if temperature of air is −30∘C ? Take, Density of ice =1gcm−3 , Latent heat of ice =80calg−1 , Conductivity of ice =0.008cals−1cm−1∘C−1
View SolutionA hollow sphere of glas whose external and internal radii are 11 cm and 9 cm respectively is completely filled with ice at 0∘C and placed in a bath of boiling water. How long will it take for the ice to melt completely? Given that density of ice = 0.9g/cm3, latent heat of fusion of ice =80cal/g and thermal conductivity of glass =0.002cal/cm−s∘C.
View SolutionA cubical block of wood 10cm on a side, floats at the interface of oil and water as shown in figure. The density of oil is 0.6gcm−3 and density of water is 1gcm−3. The mass of the block is
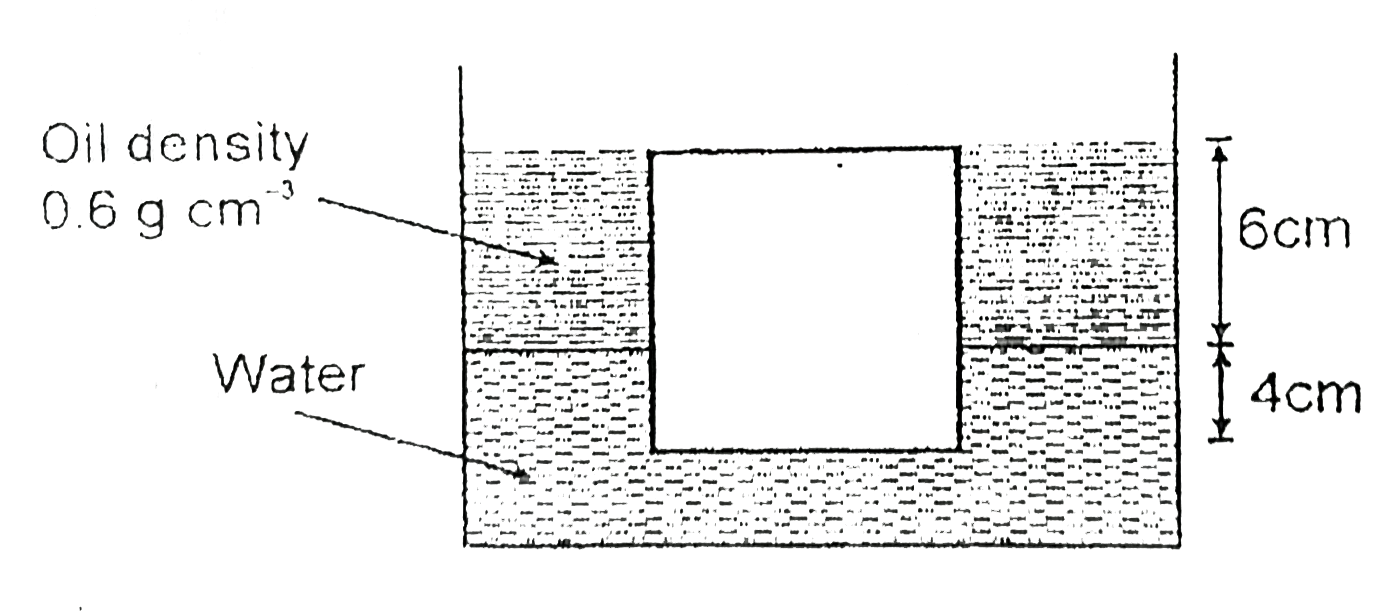 View Solution
View Solution

