In an examination paper of five questions, 5% of the candidates answered all of them and 5% answered none. Of the rest, 25% candidates answered only one question and 20% answered 4 questions. If 396 candidates answered either 2 questions or 3 questions, the number of candidates that appeared for the examination was
800
1000
850
900
The correct Answer is:A
To solve the problem step by step, we will break down the information given and calculate the total number of candidates who appeared for the examination.
Step 1: Define the total number of candidates
Let the total number of candidates who appeared for the examination be A.
Step 2: Calculate the number of candidates who answered all questions
5% of the candidates answered all 5 questions. Therefore, the number of candidates who answered all questions is:
Candidates who answered all=5100×A=A20
Step 3: Calculate the number of candidates who answered none
Similarly, 5% of the candidates answered none of the questions. Therefore, the number of candidates who answered none is also:
Candidates who answered none=5100×A=A20
Step 4: Calculate the remaining candidates
The remaining candidates are those who answered either 1, 2, 3, or 4 questions. We can find this by subtracting the candidates who answered all and none from the total:
Remaining candidates=A−(A20+A20)=A−2A20=A−A10=9A10
Step 5: Calculate the number of candidates who answered only one question
25% of the remaining candidates answered only one question:
Candidates who answered only one=25100×9A10=9A40
Step 6: Calculate the number of candidates who answered four questions
20% of the remaining candidates answered four questions:
Candidates who answered four=20100×9A10=9A50
Step 7: Calculate the number of candidates who answered either 2 or 3 questions
We know that 396 candidates answered either 2 or 3 questions. Thus, we can express this as:
Candidates who answered 2 or 3=9A10−(9A40+9A50)
Step 8: Find a common denominator and simplify
To simplify 9A40+9A50, we find the LCM of 40 and 50, which is 200:
9A40=9A×5200=45A200
9A50=9A×4200=36A200
Thus,
9A40+9A50=45A+36A200=81A200
Step 9: Set up the equation
Now we can set up the equation for the candidates who answered either 2 or 3 questions:
9A10−81A200=396
Converting 9A10 to have a denominator of 200 gives:
9A×20200=180A200
Thus, the equation becomes:
180A200−81A200=396
99A200=396
Step 10: Solve for A
Now, multiply both sides by 200:
99A=396×200
99A=79200
Now, divide by 99:
A=7920099=800
Conclusion
The total number of candidates that appeared for the examination is 800.
Let the total number of candidates who appeared for the examination be A.
5% of the candidates answered all 5 questions. Therefore, the number of candidates who answered all questions is:
Candidates who answered all=5100×A=A20
Similarly, 5% of the candidates answered none of the questions. Therefore, the number of candidates who answered none is also:
Candidates who answered none=5100×A=A20
The remaining candidates are those who answered either 1, 2, 3, or 4 questions. We can find this by subtracting the candidates who answered all and none from the total:
Remaining candidates=A−(A20+A20)=A−2A20=A−A10=9A10
25% of the remaining candidates answered only one question:
Candidates who answered only one=25100×9A10=9A40
20% of the remaining candidates answered four questions:
Candidates who answered four=20100×9A10=9A50
We know that 396 candidates answered either 2 or 3 questions. Thus, we can express this as:
Candidates who answered 2 or 3=9A10−(9A40+9A50)
To simplify 9A40+9A50, we find the LCM of 40 and 50, which is 200:
9A40=9A×5200=45A200
9A50=9A×4200=36A200
Thus,
9A40+9A50=45A+36A200=81A200
Now we can set up the equation for the candidates who answered either 2 or 3 questions:
9A10−81A200=396
Converting 9A10 to have a denominator of 200 gives:
9A×20200=180A200
Thus, the equation becomes:
180A200−81A200=396
99A200=396
Now, multiply both sides by 200:
99A=396×200
99A=79200
Now, divide by 99:
A=7920099=800
The total number of candidates that appeared for the examination is 800.
Topper's Solved these Questions
Similar Questions
Five question were asked in an examination. 5% of the total examinees answered all 5 questions and 5% of total examinees did not answered any question. 25% of the rest of the examinees answered only 1 question and 20% answered 4 questions. 2412% of the total examinees answered only 2 questions. If 200 examinees answered 3 questions, then find the total number of examinees.
View SolutionIn an examination, 92% of the candidates passed and 46 failed. How many candidates appeared?
View Solution
Knowledge Check
- Question 1 - Select One
In an examination, 60% of the candidates passed in English and 70% of the candidates passed in Mathematics, but 20% failed in both of these subjects. If 2500 candidates passed in both the subjects, the number of candidates that appeared at the examination was
A3000B3500C4000D5000 - Question 2 - Select One
In an examination, 60% of the candidates passed in English and 70% of the candidates passed in Mathematics, but 20% failed in both of these subjects. If 2500 candidates passed in both the subjects, the number of candi dates who appeared at the ex amination was
A3000B3500C4000D5000 - Question 3 - Select One
In an examination 74% of the candidates passed and 156 failed. The candidates who appeared in the examination were
A211B400C500D600
In an examination, 94% of the candidates passed and 114 failed. How many candidates appeared?
View SolutionIn an examination 75% candidates mas in English and 60% passed in Mathematics. 25% failed in both and 240 passed the examination. Find the total number of candidates
View Solutionin an examination 96% of the candidate passed and 50 failed. how many candidate appeared?
View SolutionIn an examination 70% of the candidates passed in English, 80% passed in Mathematics. 10% failed in both the subjects if 144 candidates passed in both, the total number of candidates was :
एक परीक्षा में 70% प्रतियोगी अंग्रेजी में, 80% प्रतियोगी गणित में सफल होते है तथा 10% प्रतियोगी दोनों विषयों में असफल होते हैं। यदि 144 प्रतियोगी दोनों विषयों में सफल होते हैं, तो प्रतियोगियों की कुल संख्या क्या थी ?View SolutionStudy the following graph carefully and answer the question given below
Number of candidates appeared (in thousand) and percentage of candidates qualified in a competitive examination over the years.
No. of candidates appeared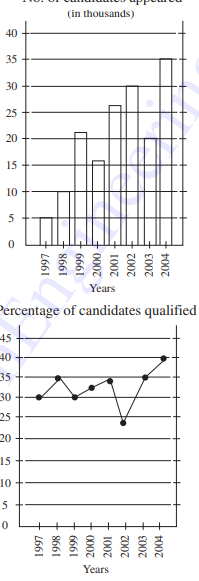
What was the percentage drop in thee number of candidates appeared from the year 2002 to 2003?View Solution

