A rectangular plank √2 metre wide is placed symmetrically on the diagonal of a square of side 8 metres as shown in the figure. The area of the plank is 7√2 sq.m (b) 14 sq.m (c) 98 sq. m (d) (16√2−3)sq.m
To find the area of the rectangular plank placed symmetrically on the diagonal of a square, we can follow these steps:
Step 1: Understand the dimensions of the square and the plank
- The side of the square is given as 8 meters.
- The width of the rectangular plank is given as √2 meters.
Hint: Remember that the diagonal of a square can be calculated using the formula d=a√2, where a is the side length of the square.
Step 2: Calculate the diagonal of the square
- The diagonal AC of the square can be calculated as:
AC=8√2 meters
Hint: Use the formula for the diagonal of a square to find the length.
Step 3: Set up the problem for the plank
- Let AP=AQ=X meters. Since the plank is symmetrical, both segments AP and AQ are equal.
Hint: The symmetry of the plank means that both halves are identical, which simplifies calculations.
Step 4: Apply the Pythagorean theorem
- In triangle PAQ, we can apply the Pythagorean theorem:
PQ2=AP2+AQ2
Given that PQ=√2:
(√2)2=X2+X2⟹2=2X2⟹X2=1⟹X=1 meter
Hint: Remember that the Pythagorean theorem relates the lengths of the sides of a right triangle.
Step 5: Calculate the lengths of segments
- The lengths PT and TQ can be calculated as:
PT=TQ=PQ2=√22=1√2 meters
Hint: Dividing the width of the plank by 2 gives the lengths of the segments on either side of the center.
Step 6: Find the length of the plank
- The length of the plank PS can be calculated as:
PS=AC−2×AT
where AT=1√2:
PS=8√2−2×1√2=8√2−√2=7√2 meters
Hint: Subtracting twice the segment length from the total diagonal gives the length of the plank.
Step 7: Calculate the area of the plank
- The area A of the rectangular plank can be calculated as:
A=length×width=PS×PQ=(7√2)×(√2)=7×2=14 square meters
Hint: The area of a rectangle is found by multiplying its length by its width.
Final Answer:
The area of the plank is 14 square meters.
- The side of the square is given as 8 meters.
- The width of the rectangular plank is given as √2 meters.
- The diagonal AC of the square can be calculated as:
AC=8√2 meters
- Let AP=AQ=X meters. Since the plank is symmetrical, both segments AP and AQ are equal.
- In triangle PAQ, we can apply the Pythagorean theorem:
PQ2=AP2+AQ2
Given that PQ=√2:
(√2)2=X2+X2⟹2=2X2⟹X2=1⟹X=1 meter
- The lengths PT and TQ can be calculated as:
PT=TQ=PQ2=√22=1√2 meters
- The length of the plank PS can be calculated as:
PS=AC−2×AT
where AT=1√2:
PS=8√2−2×1√2=8√2−√2=7√2 meters
- The area A of the rectangular plank can be calculated as:
A=length×width=PS×PQ=(7√2)×(√2)=7×2=14 square meters
The area of the plank is 14 square meters.
Topper's Solved these Questions
Similar Questions
A rectangular plank √2 metre wide is placed symmetrically on the diagonal of a square of side 8 metres as shown in the figure. The area of the plank is 7√2 sq.m (b) 14 sq.m (c) 98 sq. m (d) (16√2−3)sq.m
View SolutionThe ratio of the area of a square of side a and that of an equilateral triangle of side a, is 2:1 (b) 2:√3 4:3 (d) 4:√3
View Solution
Knowledge Check
A rectangular plank √2 m wide is placed symmetrically on the diagonal of a square of side 8 metres as shown. What is the area of the plank ?
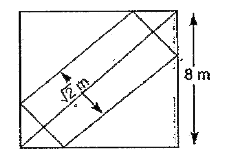 A(16√2−3) sq mB7√2 sq mC98 sq mD14 sq m
A(16√2−3) sq mB7√2 sq mC98 sq mD14 sq m1/3rd the diagonal of square is 3√2 m. What is the measure of the side of the concerned square?
A12 mB9 mC18 mD6 m
If a rectangle is inscribed in an equilateral triangle of side length 2√2 as shown in the figure, then the square of the largest area of such a rectangle is_______.

View SolutionThe diagonal of a rectangle is 10 cms and is twice the length of one of the sides. What is the area of the rectangle in sq. cm? 10√3 (b) 25 (c) 25√3 (d) 100
View SolutionThe diagonal of a square is 4√2 cm. The diagonal of another square whose area is double that of the first square, is (a) 8 cm (b) 8√2cm (c) 4√2cm (d) 16cm
View SolutionAn equilateral triangle is described on the diagonal of a square. What is the ratio of the area of the triangle to that of the square? 2 :√3 (b) 4 :√3 (c) √3:2 (d) √3:4
View SolutionThe diagonal of a rectangle is thrice its smaller side. The ratio of the length to the breadth of the rectangle is (a) 3:1 (b) √3:1 (c) √2:1 (d) 2√2:1
View SolutionIf the extremities of the diagonal fo a square are (1,-2,3) and (2,-3,5), then the length of the side is √6 b. √3 c. √5 d. √7
View Solution
