Two circular wheels of the same radius r have their central hubs at a distance of a from one another. The minimum length of a fan belt which will pass around both the wheels is (FIGURE) 2(a+πr) (b) a+π r2 (c) 2a+πr) (d) a+π r2
To find the minimum length of a fan belt that passes around two circular wheels of the same radius r with their central hubs at a distance a from each other, we can follow these steps:
1. Identify the Components of the Belt:
- The belt will consist of two straight sections connecting the two wheels and two semicircular sections around each wheel.
2. Calculate the Length of the Straight Sections:
- The distance between the centers of the two wheels is a. Since the belt will connect the two wheels directly, there will be a straight section on each side of the wheels. Therefore, the total length of the straight sections is:
Length of straight sections=a+a=2a
3. Calculate the Length of the Semicircular Sections:
- Each wheel has a radius r. The circumference of a full circle is given by 2πr, so the length of a semicircle (half of the circumference) is:
Length of one semicircle=12×2πr=πr
- Since there are two semicircular sections (one around each wheel), the total length of the semicircular sections is:
Length of semicircular sections=πr+πr=2πr
4. Combine the Lengths:
- Now, we can add the lengths of the straight sections and the semicircular sections to find the total length of the belt:
Total length of the belt=2a+2πr
5. Final Expression:
- Thus, the minimum length of the fan belt that will pass around both wheels is:
Total length=2a+πr
Conclusion:
The correct option for the minimum length of the fan belt is:
(c) 2a+πr
- The belt will consist of two straight sections connecting the two wheels and two semicircular sections around each wheel.
- The distance between the centers of the two wheels is a. Since the belt will connect the two wheels directly, there will be a straight section on each side of the wheels. Therefore, the total length of the straight sections is:
Length of straight sections=a+a=2a
- Each wheel has a radius r. The circumference of a full circle is given by 2πr, so the length of a semicircle (half of the circumference) is:
Length of one semicircle=12×2πr=πr
- Since there are two semicircular sections (one around each wheel), the total length of the semicircular sections is:
Length of semicircular sections=πr+πr=2πr
- Now, we can add the lengths of the straight sections and the semicircular sections to find the total length of the belt:
Total length of the belt=2a+2πr
- Thus, the minimum length of the fan belt that will pass around both wheels is:
Total length=2a+πr
The correct option for the minimum length of the fan belt is:
(c) 2a+πr
Topper's Solved these Questions
Similar Questions
Two circular wheels of the same radius r have their central hubs at a distance of a from one another. The minimum length of a fan belt which will pass around both the wheels is (FIGURE) 2(a+πr) (b) a+π r2 (c) 2a+πr) (d) a+π r2
View Solution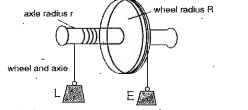
In one revolution of this wheel and axle, the effort is lowered a distance of 2πR cm. What distance is the load raised?View Solution
Knowledge Check
- Question 1 - Select One
The moment of inertia of a circular loop of radius R, at a distance of R/2 around a rotating axis parallel to horizontal diameter of loop is
AMR2B12MR2C2MR2D34MR2
If r is the radius and h is height of the cylinder the volume will be (a) 13π2h (b) πr2h (c) 2πr (h+r) (d) 2πrh the radius of the cylinder=r
height is =h
the volume is =πr2h
so the ans is (b)View SolutionFrom a uniform circular disc of radius R, a circular disc of radius R/6 and having centre at a distance R/2 from the centre of the disc is removed. Determine the centre of mass of remaining portion of the disc.
View Solution1. Circles of radius 2 are centered at the vertices of a triangle with sides lengths 8, 11 and 12. Find the length of a belt that fits tightly around those three circles as shown in the figure (A) 31+4pi (B)31+6pi (C) 31+pi (D) 31+ 2pi .
View SolutionThe area of a circle is given by A=πr2, where r is the radius . Calculate the rate of increases of area w.r.t. radius.
View SolutionTwo identical solid hemisphers of equal base radiu r cm are stuck together along their bases. The total surface area of the combination is 6πr2.
View SolutionA right circular cylinder of radius r and height h (h>2r) just encloses a sphere of diameter (a) h (b) r (c) 2r (d) 2h
View SolutionThe total surface area of a hemisphere of radius r is πr2 (b) 2πr2 (c) 3πr2 (d) 4πr2
View Solution

