ABC is a right triangle with right angle at B , if P is a mid point of AC show that PB=PA=PC=12(AC)
Step by step video & image solution for ABC is a right triangle with right angle at B , if P is a mid point of AC show that PB= PA = PC = 1/2 (AC) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
Knowledge Check
ABC is an equilateral triangle where AB = a and P is any point in its plane such that PA = PB + PC. Then PA2+PB2+PC2a2 is
A3B√34C34D2
In the given figure ABC is a right angled triangle with ∠B=90∘ . D is the mid -point of BC . Show that AC2=AD2+3CD2 .
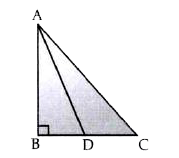
View SolutionIn Fig. 3, ABC is a right triangle, right angled at C and D is the mid-point of BC. Prove that AB2=4AD2−3AC2.
View SolutionABC is a triangle right angled at B. D is a point on AC such that ∠ABD=45∘ If AC=6 and AD=2 then AB=
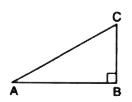
View SolutionThe given figure shows a right triangle right angled at B .
If ∠BCA=2∠BAC, show that AC=2BC
View SolutionIn a right ABC right-angled at C , if D is the mid-point of BC , prove that BC2=4 (AD2−AC2) .
View SolutionIn a right-angled triangle ABC,∠ABC=90∘ and D is mid-point of AC. Prove that BD=12AC.
View Solution1. In a right angled triangle ABC, AB = AC. Thena:b:c is
View Solution