A typist while typing the numbers from 600 to 799 mistakenly he typed 8 every time in place of 6. So the total number of times he has typed 8 is :
A. 300
B. 230
C. 180
D. none of these
The correct Answer is:C
To solve the problem of how many times the typist mistakenly typed the number 8 instead of 6 while typing numbers from 600 to 799, we can break it down step by step.
1. Identify the range of numbers: We need to consider all the numbers from 600 to 799.
2. Count occurrences of 6 in the hundreds place:
- The hundreds digit is 6 for all numbers from 600 to 699.
- There are 100 numbers in this range (600 to 699), so the digit 6 appears 100 times in the hundreds place.
3. Count occurrences of 6 in the tens place:
- The tens digit can be 6 for the numbers 660 to 669.
- There are 10 numbers in this range (660, 661, 662, ..., 669), so the digit 6 appears 10 times in the tens place.
4. Count occurrences of 6 in the units place:
- The units digit can be 6 for the numbers ending in 6, which are: 606, 616, 626, 636, 646, 656, 666, 676, 686, 696.
- There are 10 occurrences of the digit 6 in the units place.
5. Total occurrences of 6:
- Now, we add up all the occurrences of 6:
- Hundreds place: 100
- Tens place: 10
- Units place: 10
- Total = 100 + 10 + 10 = 120.
6. Conclusion:
- Since the typist typed 8 every time he should have typed 6, the total number of times he typed 8 is 120.
Final Answer:
The total number of times the typist has typed 8 is 120.
---
- The hundreds digit is 6 for all numbers from 600 to 699.
- There are 100 numbers in this range (600 to 699), so the digit 6 appears 100 times in the hundreds place.
- The tens digit can be 6 for the numbers 660 to 669.
- There are 10 numbers in this range (660, 661, 662, ..., 669), so the digit 6 appears 10 times in the tens place.
- The units digit can be 6 for the numbers ending in 6, which are: 606, 616, 626, 636, 646, 656, 666, 676, 686, 696.
- There are 10 occurrences of the digit 6 in the units place.
- Now, we add up all the occurrences of 6:
- Hundreds place: 100
- Tens place: 10
- Units place: 10
- Total = 100 + 10 + 10 = 120.
- Since the typist typed 8 every time he should have typed 6, the total number of times he typed 8 is 120.
The total number of times the typist has typed 8 is 120.
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - FINAL ROUND116videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 140videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - LEVEL 1140videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNCTIONS AND GRAPHEXERCISE - Final Round40videos
FUNCTIONS AND GRAPH
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)52videos
GEOMETRY
Similar Questions
A typist has to type a manuscript of 40 pages. She has typed 30 pages of the manuscript. What is the ratio of the number of pages typed to the number of pages left?
View SolutionRohan went to the post-office to buy five-rupee, two-rupee and one-rupee stamps. He paid the clerk Rs 20, and since he had no change, he gave Rohan three more one-rupee stamps. If the number of stamps of each type that he had ordered initially was more than one, what was the total number of stamps that he bought? (a) 8 (b) 9 (c) 10 (d) 12
View Solution
Knowledge Check
- Question 1 - Select One
A typist while typing the numbers from 600 to 799 mistakenly he typed 8 every time in place of 6. So the total number of times he has typed 8 is :
A300B230C180Dnone of these - Question 2 - Select One
A person starts typing the numbers from 1 to 1999. He press the keys total 'n' number of times. The value of n is L
A6889B1000C2888DNone of these - Question 3 - Select One
A person starts typing the numbers from 1 to 1999. He press the keys total 'n' number of times. The value of n is L
A6889B1000C2888Dnone of these
Roshan wanted to type the first 190 whole numbers. Find the number of times he had to press the numbered keys.
View SolutionThe number of gram-atoms in 8 g of He are
View SolutionIn the given bar graph presents the number of different types of vehicles exported by a company during 2014 and 2015?
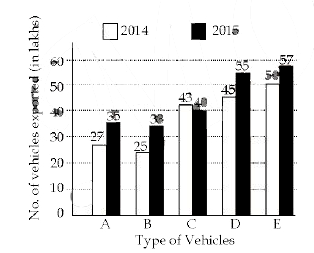
What is the ratio of the total number of type A and C vehicles exported in 2014 to the total number of type B and E vehicles exported in 2015?View SolutionThe given Bar Graph presents Different Type of Vehicles (in lakhs) exported by a company in 2016 and 2017.
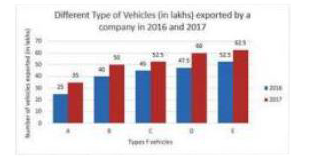
The total number of vehicles of type C and E exported by the company in 2017 is what percentage (correct to one decimal place) more than the total number of vehicles of type A, B and C exported in 2016?View SolutionIn given bar graph presents the number of different types of vehicles exported by a company during 2014?

The total number of type B,D and E vehicles exported in 2014 is what percentage of the total number of type A,C,D and E vehicles exported in 2015View Solution

