Capt.Manoj Panday once decided to distribute 180 bullets among his 36 soldiers. But he gave n bullets to a soldier of nth row and there were same number of soldiers in each row. Thus he distributed all his 180 bullets among his soldiers. The number of soldiers in (n - 1)th row was:
3
8
9
none of these
The correct Answer is:D
To solve the problem, we need to determine the number of soldiers in the (n - 1)th row given that Captain Manoj Panday distributed 180 bullets among 36 soldiers, with each soldier in the nth row receiving n bullets.
1. Understand the total distribution: We know that there are 180 bullets distributed among 36 soldiers. This means that the total number of soldiers is 36.
2. Define the number of rows: Let’s assume there are n rows of soldiers, and each row has the same number of soldiers, which we will denote as x. Therefore, the total number of soldiers can be expressed as:
n⋅x=36
3. Understanding the bullet distribution: According to the problem, the distribution of bullets is such that:
- The 1st row receives 1 bullet per soldier,
- The 2nd row receives 2 bullets per soldier,
- The 3rd row receives 3 bullets per soldier,
- and so on, up to the nth row, which receives n bullets per soldier.
4. Calculate total bullets distributed: The total number of bullets distributed can be expressed as:
Total bullets=1⋅x+2⋅x+3⋅x+…+n⋅x
This can be simplified using the formula for the sum of the first n natural numbers:
Total bullets=x⋅(n(n+1)2)
Setting this equal to the total bullets (180):
x⋅n(n+1)2=180
5. Substituting for x: From the equation n⋅x=36, we can express x as:
x=36n
Substituting this into the bullets equation gives:
36n⋅n(n+1)2=180
6. Simplifying the equation: We can simplify this equation:
36⋅n+12=180
Multiplying both sides by 2:
36(n+1)=360
Dividing both sides by 36:
n+1=10
Therefore:
n=9
7. Finding the number of soldiers in (n - 1)th row: Since n=9, the number of soldiers in each row (x) can be calculated as:
x=36n=369=4
Thus, the number of soldiers in the (n - 1)th row (which is the 8th row) is also:
x=4
Final Answer:
The number of soldiers in the (n - 1)th row is 4.
n⋅x=36
- The 1st row receives 1 bullet per soldier,
- The 2nd row receives 2 bullets per soldier,
- The 3rd row receives 3 bullets per soldier,
- and so on, up to the nth row, which receives n bullets per soldier.
Total bullets=1⋅x+2⋅x+3⋅x+…+n⋅x
This can be simplified using the formula for the sum of the first n natural numbers:
Total bullets=x⋅(n(n+1)2)
Setting this equal to the total bullets (180):
x⋅n(n+1)2=180
x=36n
Substituting this into the bullets equation gives:
36n⋅n(n+1)2=180
36⋅n+12=180
Multiplying both sides by 2:
36(n+1)=360
Dividing both sides by 36:
n+1=10
Therefore:
n=9
x=36n=369=4
Thus, the number of soldiers in the (n - 1)th row (which is the 8th row) is also:
x=4
The number of soldiers in the (n - 1)th row is 4.
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 140videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - TEST OF YOU - LEARNING - 240videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNDAMENTALS EXERCISE - LEVEL 2123videos
FUNDAMENTALS
- BOOK - ARIHANT SSCCHAPTER - FUNCTIONS AND GRAPHEXERCISE - Final Round40videos
FUNCTIONS AND GRAPH
- BOOK - ARIHANT SSCCHAPTER - GEOMETRYEXERCISE - EXERCISE(LEVEL 2)52videos
GEOMETRY
Similar Questions
A general arrangers his soldiers in rows to form a perfect square. He finds that in doing so, 60 soldiers are are left out. If the total number of soldiers be 8160, find the number of soldiers in each row.
View SolutionA rich man is on the verge of dying. He has unlimited amount of money and intends to distribute some of it among his relatives with the following conditions (a) total money that is to be distributed is a positive multiple of four (b) no relative gets more then (4n−1) rupees, then total number of ways in which the rich man can write his will
View Solution
Knowledge Check
- Question 1 - Select One
Capt.Manoj Panday once decided to distribute 180 bullets among his 36 soldiers. But he gave n bullets to a soldier of nth row and there were same number of soldiers in each row. Thus he distributed all his 180 bullets among his soldiers. The number of soldiers in (n - 1)th row was:
A3B8C9Dnone of these - Question 2 - Select One
Lieutenant Kalia when arranged all his 1500 solidiers in such a way that the number of soldiers in a line were the same as there were the number of lines. So he was left with 56 soldiers , who were not a part of this arrangment. The number of lines in this arrangement is :
A44B36C38DNone of these - Question 3 - Select One
A General can draw up his soldiers in the rows of 10, 15 or 18 soldiers and he can also draw them up in the form of a solid square. Find the least number of soldiers with the General.
A100B3600C900D90
A shopkeeper sells three varieties of perfumes and he has a large number of bottles of the same size of each variety in his stock. There are 5 places in a row in his showcase.The number of different ways of displaying the three varieties of perfumes in the show case is
View SolutionA general can draw up his soldiers in the rows of 10, 15 and 18 soldiers and he can also draw them up in the form of a solid square. Find the least number of soldiers with the general
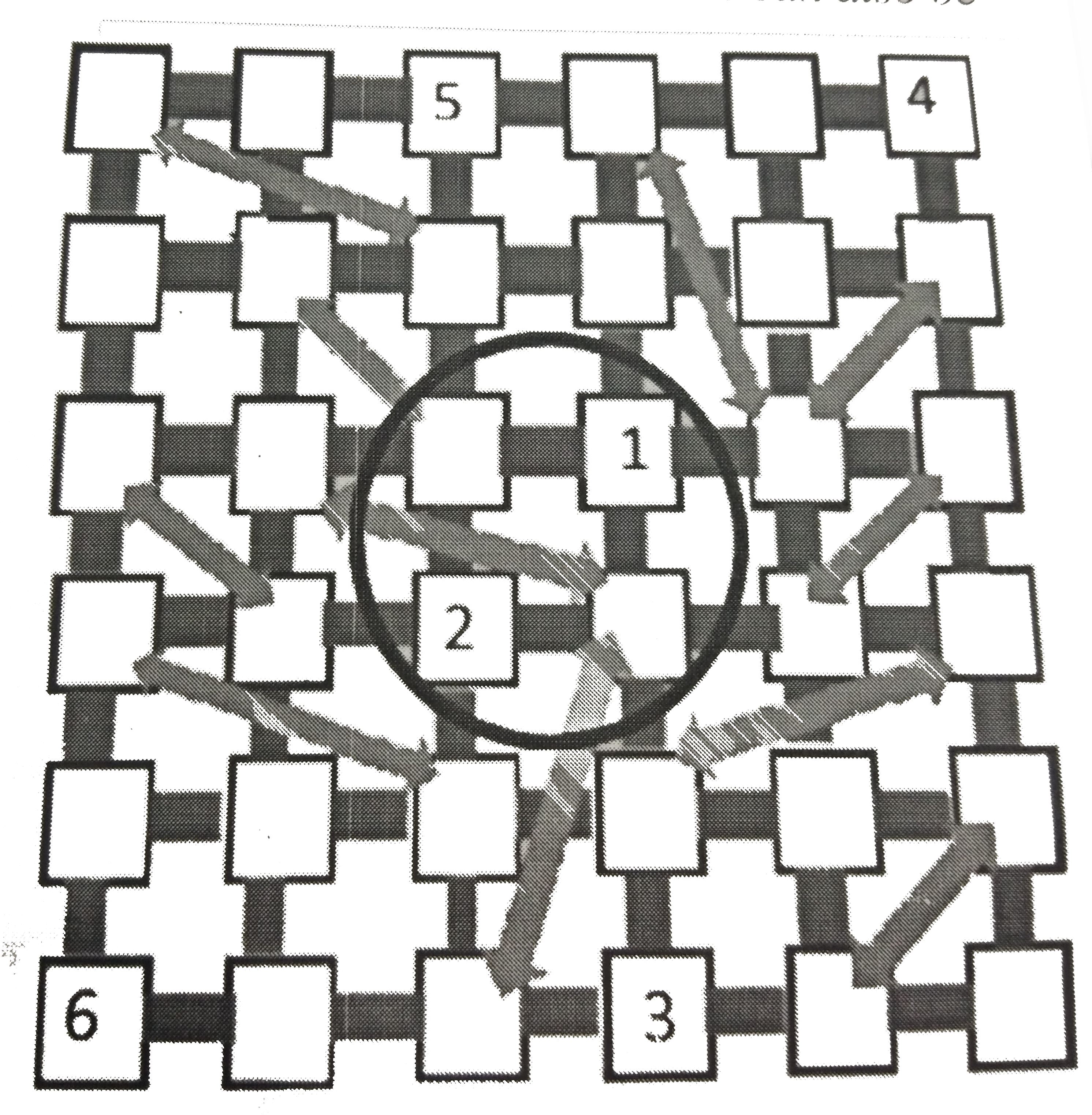
View SolutionAfter having celebrated his brother’s birthday, Max makes his way to Fuchsia city, to take a boat to Cinnabar Island. The sailor, Mr. Hendricks, is however in a dilemma. There is a castle in the middle of the sea on the way to Cinnabar Is land. Owing to issues, he needs to bribe each and every soldier with 10 PokéDollars to pass through. He is not sure of the number of soldiers in the main towers due to the new rules that have been imposed. He seeks Max’s help. In the castle, there are passways as straight row and column (passways are the dark grey strips) at any point when you see left or right or up or down . Also there are some magical tunnels joining one stop (stops are the places where troops of soldiers stand) to the other in a non-straight manner. The magic of the tunnel is clon- ing the soldiers. If you place any number of soldiers in one stop, then all stops joined to it through the tunnel will have same number of cloned soldiers. Cloned soldiers can also be cloned if there is one more. Number of soldiers at any stop in any row or column are more than one and sum of all soldiers at all stops in any row or column is same. Every stop is marked a number from 1 to 6 and stops in any row or column have different numbers. Soldier troop with ‘x’ number of soldier will stand on the stop marked as ‘x’. There are some soldier having all time duty and they are depicted in the figure with their positions. But fearing for safety, the king increased the security and ordered to place a soldier on every stop in the above way of his convenience. The circle in the middle represents the main tower and Max has to find the number of extra soldiers. The answer is ________
 View Solution
View SolutionA shopkeeper sells three varieties of perfumes and he has a large number of bottles of the same size of each variety in his stock. There are 5 places in a row in his showcase. The number of different ways of displaying the three varieties of perfumes in the show case, is
View SolutionA shopkeeper sells three varieties of perfumes and he has a large number of bottles of the same size of each variety in his stock. There are 5 places in a row in his showcase. The number of different ways of displaying the three varieties of perfumes in the show case, is
View Solution

