If the ratio of total surface area to the curved surface area of a cylinder be 4:1, what is the ratio of radius to the height ?
4:1
2:3
3:2
3:1
The correct Answer is:D
To solve the problem, we need to find the ratio of the radius (r) to the height (h) of a cylinder given that the ratio of the total surface area (TSA) to the curved surface area (CSA) is 4:1.
1. Understand the Formulas:
- The total surface area (TSA) of a cylinder is given by the formula:
TSA=2πr(r+h)
- The curved surface area (CSA) of a cylinder is given by the formula:
CSA=2πrh
2. Set Up the Ratio:
- According to the problem, the ratio of TSA to CSA is given as:
TSACSA=41
- Substituting the formulas for TSA and CSA, we have:
2πr(r+h)2πrh=41
3. Simplify the Equation:
- The 2π terms in the numerator and denominator cancel out:
r(r+h)rh=4
- This simplifies to:
r+hh=4
4. Cross-Multiply:
- Cross-multiplying gives:
r+h=4h
5. Rearrange the Equation:
- Rearranging the equation, we get:
r=4h−h
- This simplifies to:
r=3h
6. Find the Ratio:
- To find the ratio of radius to height, we write:
rh=3hh=3
- Therefore, the ratio of radius to height is:
r:h=3:1
Final Answer:
The ratio of the radius to the height of the cylinder is 3:1.
- The total surface area (TSA) of a cylinder is given by the formula:
TSA=2πr(r+h)
- The curved surface area (CSA) of a cylinder is given by the formula:
CSA=2πrh
- According to the problem, the ratio of TSA to CSA is given as:
TSACSA=41
- Substituting the formulas for TSA and CSA, we have:
2πr(r+h)2πrh=41
- The 2π terms in the numerator and denominator cancel out:
r(r+h)rh=4
- This simplifies to:
r+hh=4
- Cross-multiplying gives:
r+h=4h
- Rearranging the equation, we get:
r=4h−h
- This simplifies to:
r=3h
- To find the ratio of radius to height, we write:
rh=3hh=3
- Therefore, the ratio of radius to height is:
r:h=3:1
The ratio of the radius to the height of the cylinder is 3:1.
Topper's Solved these Questions
- MENSURATIONBOOK - ARIHANT SSCCHAPTER - MENSURATIONEXERCISE - INTRODUCTORY EXERCISE- 10.7
12 Videos
- MENSURATIONBOOK - ARIHANT SSCCHAPTER - MENSURATIONEXERCISE - EXERCISE (MISCELLANEOUS)
59 Videos
- MENSURATIONBOOK - ARIHANT SSCCHAPTER - MENSURATIONEXERCISE - INTRODUCTORY EXERCISE- 10.5
32 Videos
- LOGARITHM BOOK - ARIHANT SSCCHAPTER - LOGARITHM EXERCISE - EXERCISE LEVEL 2
19 Videos
- MIXED GRAPHBOOK - ARIHANT SSCCHAPTER - MIXED GRAPHEXERCISE - Higher Skill Level Questions
15 Videos
Similar Questions
Knowledge Check
The ratio of the total surface area to the lateral surface area of a cylineder with base radius 80 cm and height 20 cm is
A2:1B3:1C4:1D5:1The ratio of the total surface area to the lateral surface area of a cylinder whose radius is 20 cm and height 60 cm, is
A2:1B3:2C4:3D5:3The ratio of the total surface area to the lateral surface area of a cylineder with base radius 80 cm and height 20 cm is
A2:1B3:1C4:1D5:1
As shown in the adjacent figure, a sphere is placed in a cylinder. It touches the top, bottom and the curved surface of the cylinder. If radius of the base of the cylinder is 'r',
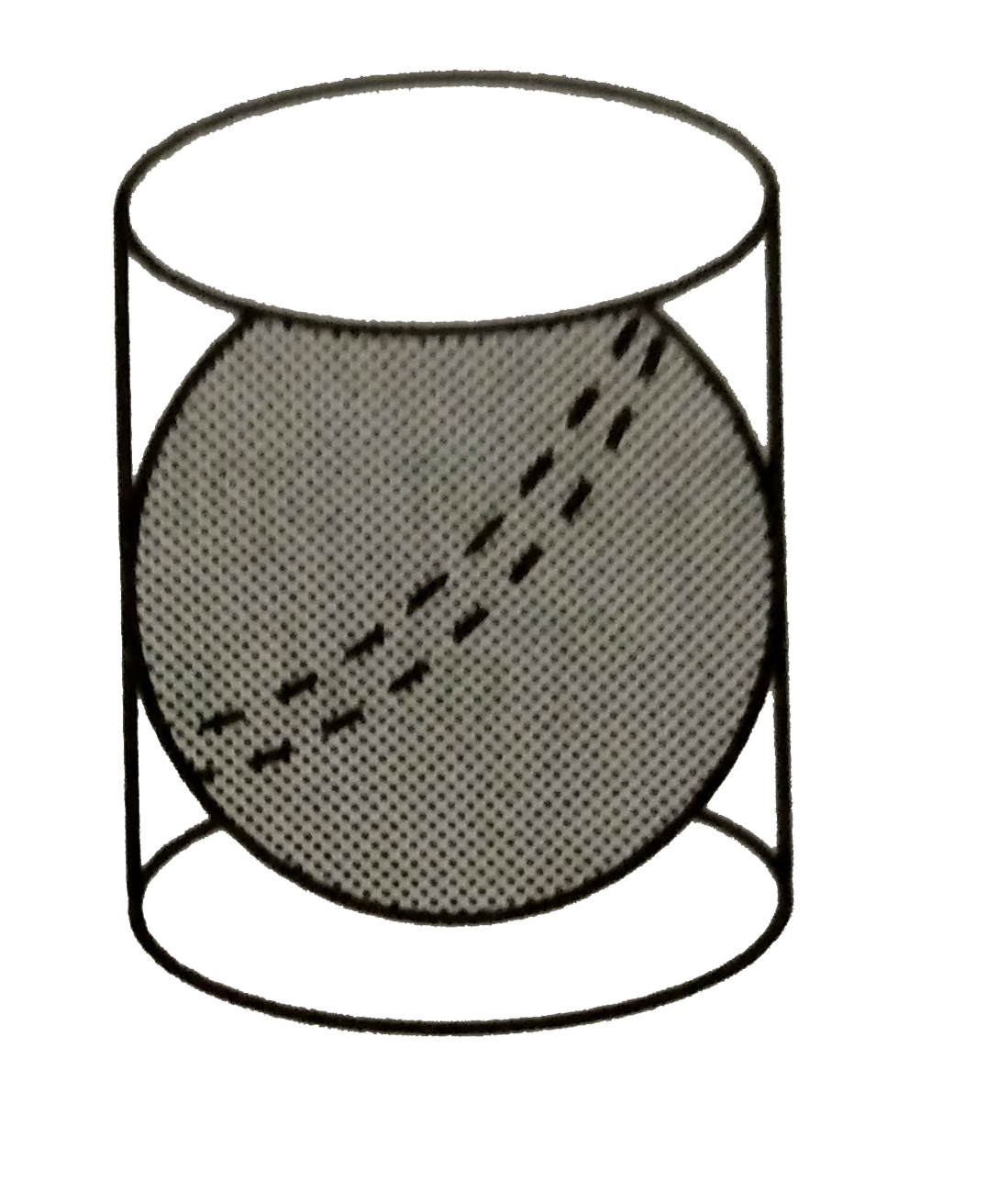
(i) what is ratio of the radii of the sphere and the cylinder?
(ii)what is the ratio of the curved surface area of the cylinder and the surface area of the sphere?
(iii) what is the ratio of the volumes of the cylinder and the sphere?
View SolutionThe ratio of total surface area to lateral surface area of a cylinder whose radius is 20 cm and height 60 cm, is (a) 2 : 1 (b) 3 : 2 (c) 4 : 3 (d) 5 : 3
View SolutionCurved surface area of a cylinder of radius h and height r is _______.
View SolutionThe ratio of the surface area of a sphere and the curved surface area of the cylinder circumscribing the sphere is
View SolutionThe curved surface area of a cylinder with radius 2cm and height 7 cm will be :
View Solution
