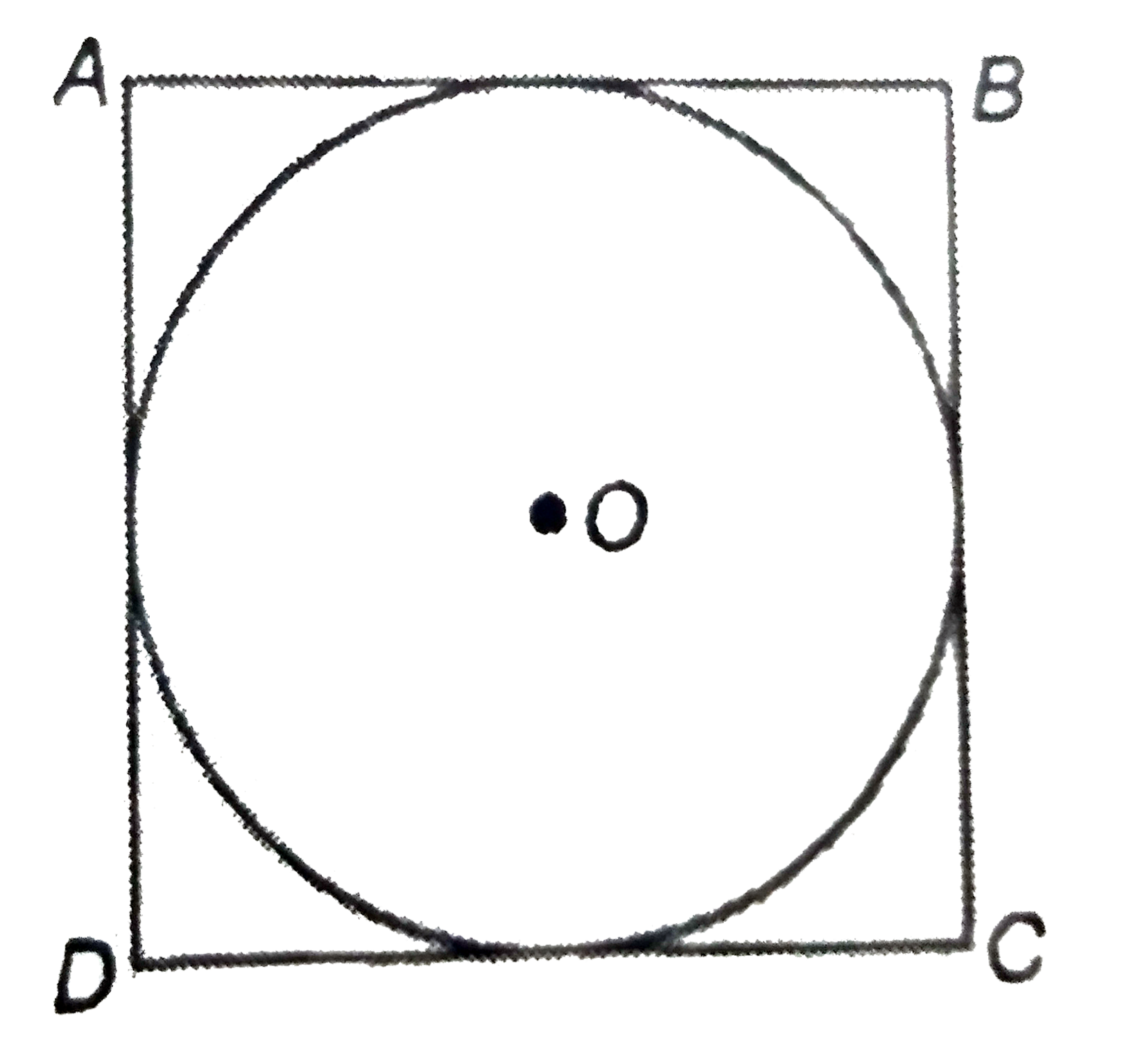
In the adjoining figure ABCD is a square . Four equal semicircles are drawn in such a way that they meet each other at 'O' . Sides AB,BC, CD and AD are the respective diameters of the four semicircles. Each of the side of the square is 8 cm. Find the area of the shaded region :
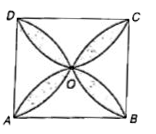
32(π−2)cm2
16(π−2)cm2
(2π−8)cm2
(34π−4)cm2
The correct Answer is:A
Step by step video, text & image solution for In the adjoining figure ABCD is a square . Four equal semicircles are drawn in such a way that they meet each other at 'O' . Sides AB,BC, CD and AD are the respective diameters of the four semicircles. Each of the side of the square is 8 cm. Find the area of the shaded region : by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - MENSURATIONEXERCISE - TEST OF YOUR LEARNING18videos
MENSURATION
- BOOK - ARIHANT SSCCHAPTER - MENSURATIONEXERCISE - EXERCISE (LEVEL 1)55videos
MENSURATION
- BOOK - ARIHANT SSCCHAPTER - LOGARITHM EXERCISE - EXERCISE LEVEL 219videos
LOGARITHM
- BOOK - ARIHANT SSCCHAPTER - MIXED GRAPHEXERCISE - Higher Skill Level Questions15videos
MIXED GRAPH
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the area of shaded portion if each side of the square is 14 cm.
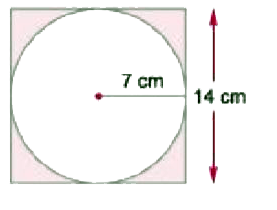 A50 sq cmB45 sq cmC62 sq cmD42 sq cm
A50 sq cmB45 sq cmC62 sq cmD42 sq cm - Question 2 - Select One
In the adjoining figure, ABCD is a square of side 7 cm and two semicircles are drawn inside of it with AB and CD as diameters. Find the area of the shaded region (in cm2).
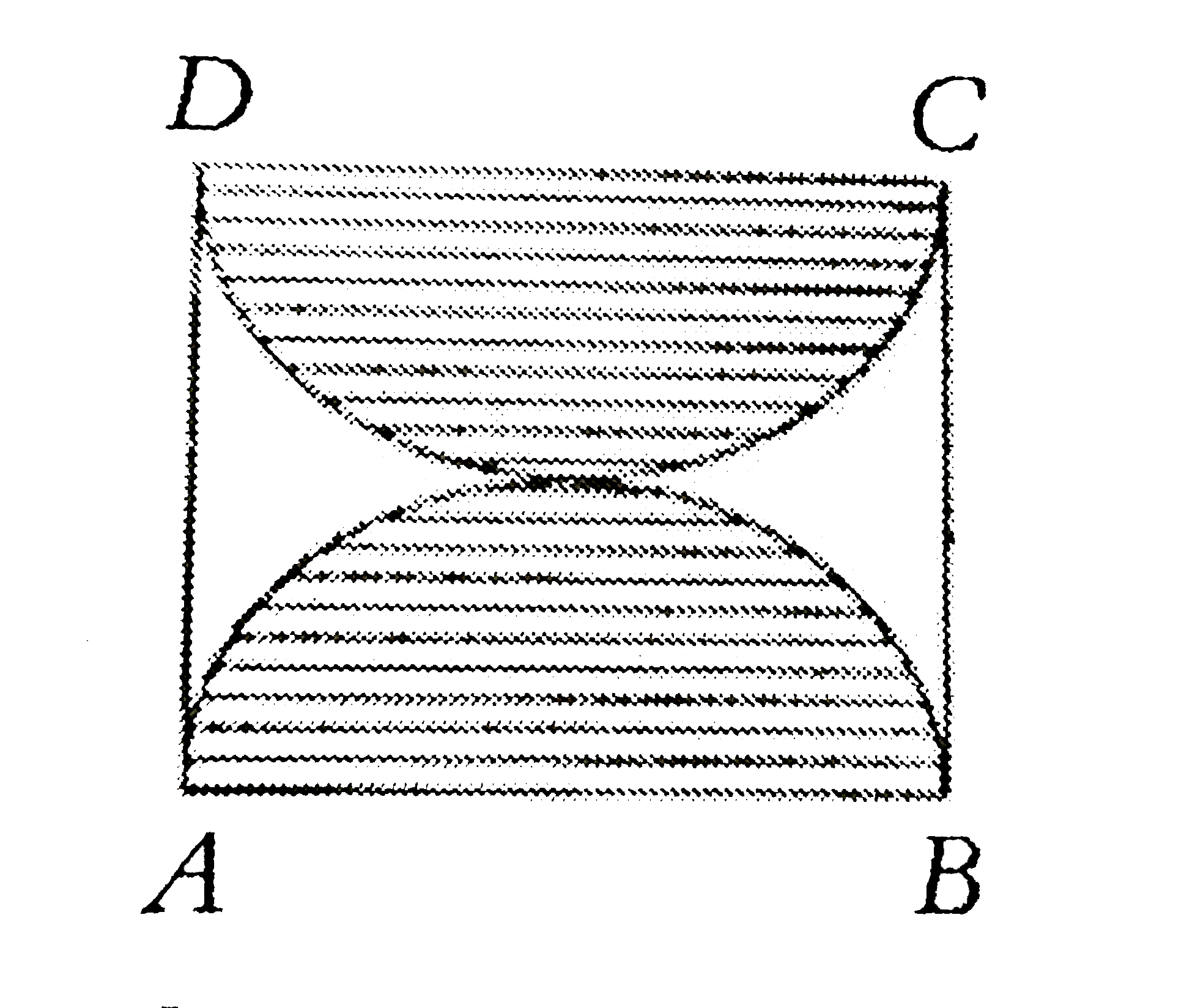 A144 m2B164 m2C184 m2D154 m2
A144 m2B164 m2C184 m2D154 m2 - Question 3 - Select One
In the adjoining figure ABCD is a square . A circle ABCD is passing through all the four vertices of the square. There are two more circles on the sides AD and BC touching each other inside the square , AD and BC are the respective diameters of the two smaller circles. Area of the square is 16cm2.
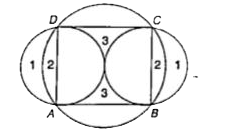
What is the area of region 2 ?A3(π−2)cm2B(π−3)cm2C(2π−3)cm2D4(π−2)cm2
In the given figure, a circle is inscribed in a square ABCD of side 28 cm. Find the area of the shaded region.
View Solutionin the adjoining figure ABCD is a square of side 14 cm. Find the radius of the circle
 View Solution
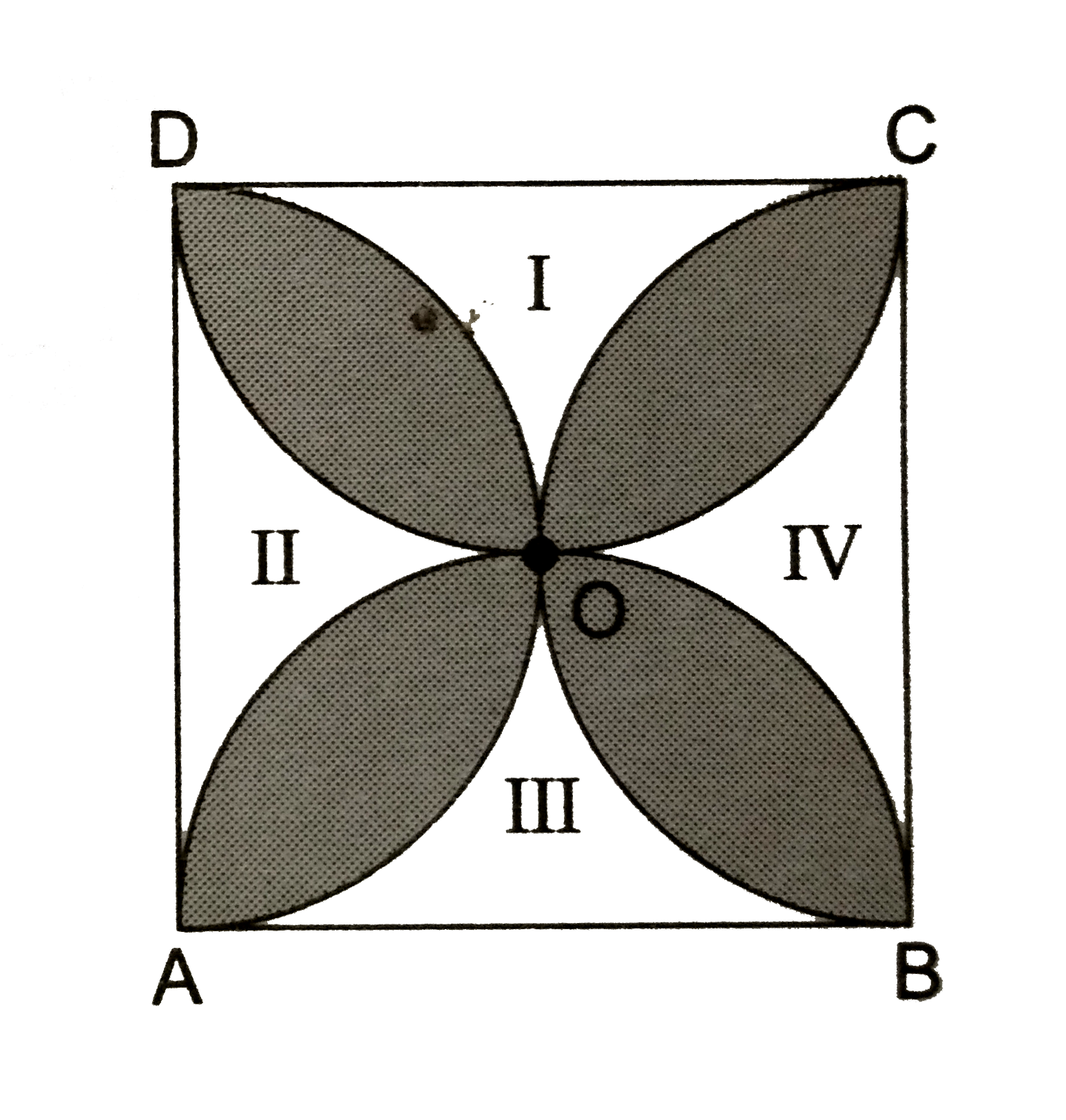
View SolutionIn the given figure, ABCD is a square of side 10 cm and semicircles are drawn with each side of the square as diameter. Find the area of the shaded region.
[ Use π=3.14]
 View Solution
View SolutionIn the figure, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region.
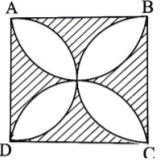 View Solution
View SolutionIn the given figure, ABCD is a square of side 14cm.Semicircles are drawn with each side of square as diameter. Find the area of the shaded region.
View Solution


