Using Ohm's law, calculate the resistance of combination of few resistances joined in series.
To calculate the resistance of a combination of resistances joined in series using Ohm's law, we can follow these steps:
1. Understanding the Circuit:
- Assume we have two resistances, R1 and R2, connected in series with a voltage source V.
2. Applying Ohm's Law:
- According to Ohm's law, the voltage across a resistor is given by V=I×R, where I is the current flowing through the resistor and R is the resistance.
3. Voltage Drops:
- The voltage drop across R1 is V1=I×R1 and across R2 is V2=I×R2.
4. Total Voltage:
- The total voltage supplied by the source is equal to the sum of the voltage drops across the resistors:
V=V1+V2
- Substituting the expressions for V1 and V2:
V=I×R1+I×R2
5. Factoring Out the Current:
- We can factor out the current I:
V=I(R1+R2)
6. Finding the Equivalent Resistance:
- From the equation above, we can identify the equivalent resistance R of the series combination:
R=R1+R2
7. Generalizing for Multiple Resistors:
- If there are more resistors in series, say R3,R4,…,Rn, the equivalent resistance can be generalized as:
Req=R1+R2+R3+…+Rn
8. Special Case for Identical Resistors:
- If all resistances are the same (let's say R), then for n resistors, the equivalent resistance can be calculated as:
Req=n×R
Final Result:
The equivalent resistance Req for resistors in series is given by:
Req=R1+R2+R3+…+Rn
- Assume we have two resistances, R1 and R2, connected in series with a voltage source V.
- According to Ohm's law, the voltage across a resistor is given by V=I×R, where I is the current flowing through the resistor and R is the resistance.
- The voltage drop across R1 is V1=I×R1 and across R2 is V2=I×R2.
- The total voltage supplied by the source is equal to the sum of the voltage drops across the resistors:
V=V1+V2
- Substituting the expressions for V1 and V2:
V=I×R1+I×R2
- We can factor out the current I:
V=I(R1+R2)
- From the equation above, we can identify the equivalent resistance R of the series combination:
R=R1+R2
- If there are more resistors in series, say R3,R4,…,Rn, the equivalent resistance can be generalized as:
Req=R1+R2+R3+…+Rn
- If all resistances are the same (let's say R), then for n resistors, the equivalent resistance can be calculated as:
Req=n×R
The equivalent resistance Req for resistors in series is given by:
Req=R1+R2+R3+…+Rn
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MBD -HARYANA BOARDCHAPTER - CURRENT ELECTRICITYEXERCISE - LONG ANSWER TYPE QUESTIONS
6 Videos
- CURRENT ELECTRICITYBOOK - MBD -HARYANA BOARDCHAPTER - CURRENT ELECTRICITYEXERCISE - OBJECTIVE TYPE QUESTIONS
20 Videos
- CURRENT ELECTRICITYBOOK - MBD -HARYANA BOARDCHAPTER - CURRENT ELECTRICITYEXERCISE - OBJECTIVE TYPE QUESTIONS
20 Videos
- BOARD QUESTION PAPER (SOLVED) - 2019BOOK - MBD -HARYANA BOARDCHAPTER - BOARD QUESTION PAPER (SOLVED) - 2019EXERCISE - LONG ANSWER TYPE QUESTIONS
4 Videos
- DUAL NATURE OF MATTER AND RADIATIONSBOOK - MBD -HARYANA BOARDCHAPTER - DUAL NATURE OF MATTER AND RADIATIONSEXERCISE - OBJECTIVE TYPE QUESTIONS
17 Videos
Similar Questions
Kirchoff's Law, Combination Of Resistance
View SolutionA student while verifying Ohm's law calculated the value of resistance of the resistor for each set of observation .However, the values of resistance were slightly different from the actual value . Is his experiment wrong ? Justify your answer.
View Solution
Knowledge Check
For series combination total resistance R will be:
A1R=1R1+1R2+1R3+....BR=R1R2R3.....CR=1R1+1R2+1R3+.....DR=R1+R2+R3+....The equivalent resistance of a series combination of two resistances is X ohm. If the resistance are of 10 ohm and 40 ohm respectively, the value of X will be
A10 ohmB20 ohmC50 ohmD40 ohmThe resistance of the series combination of two resistances is S. When they are joined in parallel the total resistance is P. If S= nP then the minimum possible value of n is
A2B3C4D1
Establish a relationship to determine the equivalent resistance R of a combination of three resistors having resistances R1,R2andR3 connected in series.
(b) Calculate the equivalent resistance R of a combination of three resistors of 2Ω,3Ωand6Ω joined in parallel.
View SolutionWhat is the ratio of equivalent resistance of series combination of n equal resistance to the equivalent resistance in parallel combination of these n resistances ?
View SolutionCalculate the combined resistance in each case:
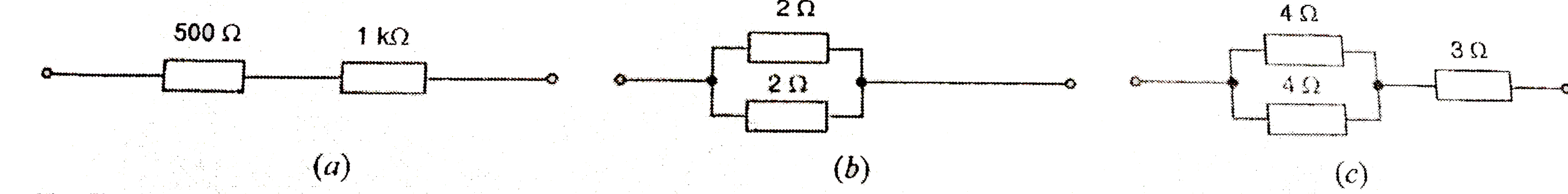
View SolutionDerive expression for equivalent resistance of a parallel combination of resistances.
(b) Calculate the ratio of equivalent resistance for a series combination of n number of identical resistors to the parallel combination of the same type of n number of resistors.
View SolutionThe equivalent resistance in series combination is
View Solution
