If from point 100 m above the ground the angles of depression of two objects due south on the ground are 60∘and45∘, then the distance between the objects is :
50(3−√3)3m
50(3+√3)3m
100(3+√3)3m
100(3−√3)3m
The correct Answer is:D
To solve the problem, we need to find the distance between two objects on the ground based on the angles of depression from a point 100 m above the ground.
1. Understanding the Scenario:
- We have a point (let's call it A) that is 100 m above the ground.
- From point A, the angles of depression to two objects on the ground are 60° and 45°.
2. Drawing the Diagram:
- Draw a vertical line representing the height of 100 m (point A).
- Draw horizontal lines from point A to the two objects on the ground (let's call them O1 and O2).
- The angle of depression to O1 is 60° and to O2 is 45°.
3. Identifying the Triangles:
- From point A to O1, we can form a right triangle (A-O1) where:
- The height (opposite side) = 100 m
- The angle of depression = 60°
- From point A to O2, we can form another right triangle (A-O2) where:
- The height (opposite side) = 100 m
- The angle of depression = 45°
4. Calculating Distances:
- For triangle A-O1 (with angle 60°):
- Using the tangent function:
tan(60°)=oppositeadjacent=100x2
- Rearranging gives:
x2=100tan(60°)=100√3≈100⋅1√3=100√3 m
- For triangle A-O2 (with angle 45°):
- Using the tangent function:
tan(45°)=oppositeadjacent=100x1
- Rearranging gives:
x1=100tan(45°)=100 m
5. Finding the Distance Between the Objects:
- The distance between the two objects O1 and O2 (let's call it d) is:
d=x1−x2=100−100√3
- Simplifying gives:
d=100(1−√3) m
6. Final Answer:
- The distance between the two objects is:
d=100(1−√3) m
- We have a point (let's call it A) that is 100 m above the ground.
- From point A, the angles of depression to two objects on the ground are 60° and 45°.
- Draw a vertical line representing the height of 100 m (point A).
- Draw horizontal lines from point A to the two objects on the ground (let's call them O1 and O2).
- The angle of depression to O1 is 60° and to O2 is 45°.
- From point A to O1, we can form a right triangle (A-O1) where:
- The height (opposite side) = 100 m
- The angle of depression = 60°
- From point A to O2, we can form another right triangle (A-O2) where:
- The height (opposite side) = 100 m
- The angle of depression = 45°
- For triangle A-O1 (with angle 60°):
- Using the tangent function:
tan(60°)=oppositeadjacent=100x2
- Rearranging gives:
x2=100tan(60°)=100√3≈100⋅1√3=100√3 m
- Using the tangent function:
tan(45°)=oppositeadjacent=100x1
- Rearranging gives:
x1=100tan(45°)=100 m
- The distance between the two objects O1 and O2 (let's call it d) is:
d=x1−x2=100−100√3
- Simplifying gives:
d=100(1−√3) m
- The distance between the two objects is:
d=100(1−√3) m
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - TRIGONOMETRYEXERCISE - INTRODUCTORY EXERCISE - 11.610videos
TRIGONOMETRY
- BOOK - ARIHANT SSCCHAPTER - TIME, SPEED AND DISTANCEEXERCISE - SPEED TEST (TSD)10videos
TIME, SPEED AND DISTANCE
- BOOK - ARIHANT SSCCHAPTER - TRUE DISCOUNT AND BANKER'S DISCOUNTEXERCISE - FAST TRACK PRACTICE23videos
TRUE DISCOUNT AND BANKER'S DISCOUNT
Similar Questions
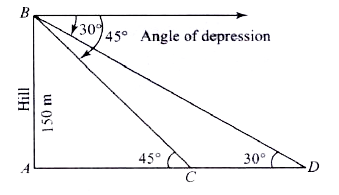
The height of a hill is 150 m. From the top of the hill the angles of depression of two objects lying towards east to the hill are 45∘ and 30∘. Find the distance btween the objects.
View SolutionAn aeroplane flying at a height of 3000 m passes vertically above another aeroplane at an instant when the angles of elevation of the two planes from some point on the ground are 60∘ and 45∘ respectively. Then the vertical distance between the two planes is
View Solution
Knowledge Check
- Question 1 - Select One
From the top of a tower of height 180 m the angles of depression of two objects on either sides of the tower are 30∘and45∘ . Then the distance between the objects are
A180(3+√3)mB180(3−√3)mC180(√3−1)mD180(√3+1)m - Question 2 - Select One
If the angle of depression of an object from a 75 m high tower is 30∘, then the distance of the object from the tower is
A25√3 mB50√3 mC75√3 mD150 m - Question 3 - Select One
From an aeroplane above a straight road the angle of depression of two positions at a distance 20 m apart on the road are observed to be 30∘ and 45∘. The height of the aeroplane above the ground is :
A10√3mB10(√3−1)mC10(√3+1)mD20m
A vertiacal pole is 60 m high, The angle of depression of two points P and Q on the ground are 30∘and45∘ respectively. If the points P and Q lie on either side of the pole, then find the distance PQ.
View SolutionAn aeroplane, when 3000 m high, passes vertically above anthoer aeroplane at an instant when the angles of elevation of the two planes from the same point on the ground are 45∘ and 60∘ respectively. Find the vertical distance between the tow planes.
View SolutionAn aeroplane when flying at a height of 4000m from the ground passes vertically above another aeroplane at an instant when the angles of the elevation of the two planes from the same point on the ground are 60o and 45o respectively. Find the vertical distance between the aeroplanes at that instant.
View SolutionAn aeroplane when flying at a height of 4000m from the ground passes vertically above another aeroplane at an instant when the angles of the elevation of the two planes from the same point on the ground are 600&450 respectively. Find the vertical distance between the aeroplanes at that instant.
View SolutionFrom an aeroplane above a straight road the angles of depression of two positions at a distance 20 m apart on the road are observed to be 30∘and45∘. The height of the aeroplane above the ground is
View Solution