Solve the following expression
(4a+2b−3c)+(3a−2b+4c)
The correct Answer is:7a+c
To solve the expression (4a+2b−3c)+(3a−2b+4c), we will follow these steps:
Step 1: Write down the expression
We start with the expression:
(4a+2b−3c)+(3a−2b+4c)
Step 2: Remove the parentheses
Since we are adding the two expressions, we can simply write them together:
4a+2b−3c+3a−2b+4c
Step 3: Combine like terms
Now we will combine the like terms. We have:
- For a: 4a+3a=7a
- For b: 2b−2b=0 (these cancel each other out)
- For c: −3c+4c=1c or simply c
Putting it all together, we have:
7a+0b+c=7a+c
Final Answer
Thus, the simplified expression is:
7a+c
---
We start with the expression:
(4a+2b−3c)+(3a−2b+4c)
Since we are adding the two expressions, we can simply write them together:
4a+2b−3c+3a−2b+4c
Now we will combine the like terms. We have:
- For a: 4a+3a=7a
- For b: 2b−2b=0 (these cancel each other out)
- For c: −3c+4c=1c or simply c
7a+0b+c=7a+c
Thus, the simplified expression is:
7a+c
Topper's Solved these Questions
- BOOK - ARIHANT SSCCHAPTER - ELEMENTS OF ALGEBRAEXERCISE - INTRODUCTORY EXERCISE - 13.191videos
ELEMENTS OF ALGEBRA
- BOOK - ARIHANT SSCCHAPTER - ELEMENTS OF ALGEBRAEXERCISE - INTRODUCTORY EXERCISE - 13.222videos
ELEMENTS OF ALGEBRA
- BOOK - ARIHANT SSCCHAPTER - DISCOUNTEXERCISE - FAST TRACK PRACTICE47videos
DISCOUNT
- BOOK - ARIHANT SSCCHAPTER - FUNCTIONS AND GRAPHEXERCISE - Final Round40videos
FUNCTIONS AND GRAPH
Similar Questions
Knowledge Check
- Question 1 - Select One
What is the value of the expression?
(a−b)3+(b−c)3+(c−a)33(a−b)(b−c)(c−a)=?
(a)4
(b)1
(c)2
(d)0A4B1C2D0 - Question 2 - Select One
Match the following columns.
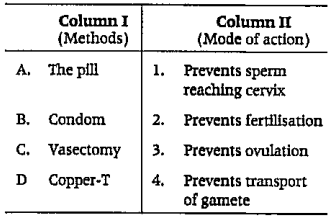
a) A-3, B- 1, C- 4 , D- 2 .
b) A- 4 , B- 1 , C- 2, D- 3.
c) A- 2 , B- 3, C- 1, D- 4.
d) A- 3, B- 4, C-1, D- 2.A B
B C
C D
D
Place the last two terms in each of the following expressions in parentheses preceded by a '−′ sign: 3a−2b+4c−5 (ii) 7a+3b+2c+4
View SolutionSimplify each of the following algebraic expressions by removing grouping symbols. a−[b−{a−(b−1)+3a}] a−[2b−{3a−(2b−3c)}]
View SolutionWrite down the coefficients of a, ab and abc in the term 4a4b2c of the algebraic expression 4a4b2c−3a3b2c+32ab3c2.
View SolutionIf a,b,c are non-zero real numbers, then the minimum value of the expression ((a4+3a2+1)(b4+5b2+1)(c4+7c2+1)a2b2c2) is not divisible by prime number.
View SolutionFactorise the following ∣∣ ∣ ∣∣3a+b+ca3+b3+c3a+b+ca2+b2+c2a4+b4+c4a2+b2+c2a3+b3+c3a5+b5+c5∣∣ ∣ ∣∣
View SolutionLet A=[2432] , B=[13−25] and C=[−2534] . Find each of the following: 2A−3B (ii) B−4C (iii) 3A−C (iv) 3A−2B+3C
View Solution

