A(-3,2),B(3,2) and C(-3,-2) are the vertices of the right trinagle ,right angled at A. Show that the mid point of the hypotenus is equidistant form the vertices.Solution in Tamil
Step by step video & image solution for A(-3,2),B(3,2) and C(-3,-2) are the vertices of the right trinagle ,right angled at A. Show that the mid point of the hypotenus is equidistant form the vertices. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- COORDINATE GEOMETRYBOOK - FULL MARKSCHAPTER - COORDINATE GEOMETRYEXERCISE - EXERCISE -5.4
7 Videos
- COORDINATE GEOMETRYBOOK - FULL MARKSCHAPTER - COORDINATE GEOMETRYEXERCISE - EXERCISE -5.5
7 Videos
- COORDINATE GEOMETRYBOOK - FULL MARKSCHAPTER - COORDINATE GEOMETRYEXERCISE - EXERCISE -5.2
12 Videos
- ALGEBRA BOOK - FULL MARKSCHAPTER - ALGEBRA EXERCISE - Assignment Answer the following questions :
1 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - Assignment (IV. Answer the following questions)
9 Videos
Similar Questions
Find the coordinate of the point of the point which divides the line segment joining the points A(4,-3) and B(9,7) in the ratio 3:2.
View SolutionFind the centroid of the triangle whose vertices are (i) (2,-4), (-3,-7) and (7,2)
View SolutionFind the distance between the following pairs of points. (i) (1,2) and (4,3)
View SolutionSubtract 5x2−4y2+6y−3 from 7x2−4xy+5y2+5x−3y
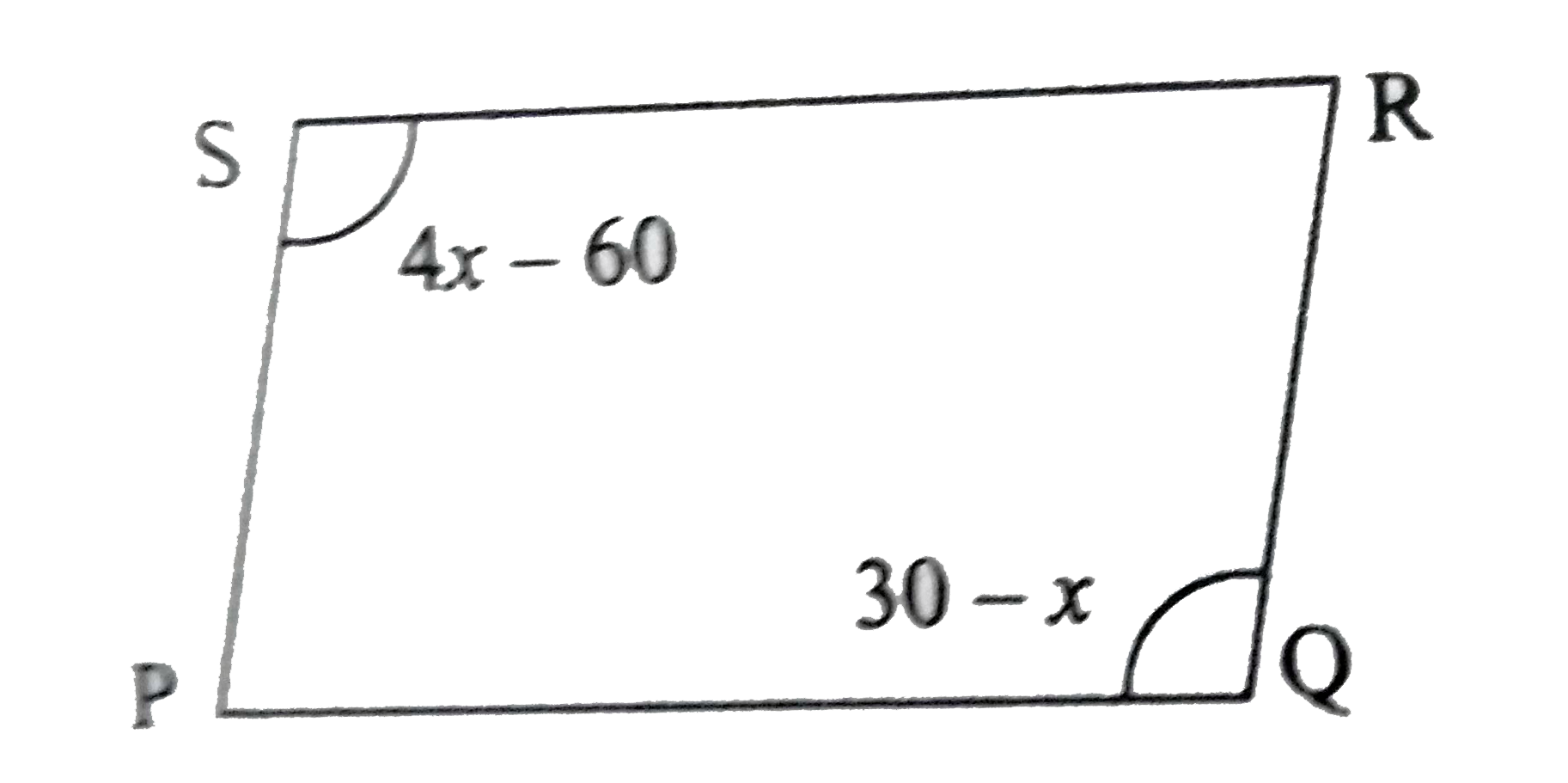
View SolutionIn the given diagram PQRS is a parellelogram.
∠S=4x−60∠Q=30−x. Find the angles of P and R .
View SolutionProve that the mid-point of the hypotenuse of a right triangle is equidistant from its vertices
View SolutionLet A(3,2),B(3,2),B(-4,1),C(3,1) and D(2,-4) be the vertices of a quadrilateral ABCD CD Find area of the quadrilateral formed by the mid-points of the sides of quadrilatdateral
View SolutionProve by the vector method that the mid-point of the hyptenuse of a right angled triangleis equidistant from the vertices of the triangle.OR
View SolutionThe points A(1,1,0),B(0,1,1),C(1,0,1)andD(23,23,23) are (A) coplanar (B) nonn coplanar (C) vertices of a parallelograsm (D) none of these
View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
View SolutionUsing analytical method prove that the mid - point of the hypotenuse of a right angled triangle is equidistant from the three vertices .
View SolutionThe vertices of a Δ ABC are A (-3,2) , B (-1,-4) and C (5,2) . If M and N are the mid - points of AB and AC respectively show that 2 MN = BC .
View SolutionThe points A(-5,4), B(-1,-2) and C(5,2) are the vertices of an isosceles right angled trinagle where the right angle is at B. Find the corrdinates of D so that ABCD is a square .
View SolutionA(-3,2),B(3,2) and C(-3,-2) are the vertices of the right trinagle ,right angled at A. Show that the mid point of the hypotenus is equidistant form the vertices.
View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
View SolutionIn Fig. 14.40, a right triangle BOA is given. C is the mid-point of the hypotenuse AB . Show that it is equidistant from the vertices O, A and B . (FIGURE)
View Solution
