An ideal gas is taken through a process PT3 = constant . The coefficient of thermal expansion of the gas in the given process in n/T. Find n.
The correct Answer is:00004.00
To solve the problem, we need to find the coefficient of thermal expansion of an ideal gas undergoing a process where PT3=constant. We will denote the coefficient of thermal expansion as γ.
1. Understand the Coefficient of Thermal Expansion:
The coefficient of thermal expansion γ is defined as:
γ=1VdVdT
2. Relate Pressure and Volume:
From the given process PT3=constant, we can express pressure P in terms of volume V and temperature T. Using the ideal gas law PV=nRT, we can write:
P=nRTV
Therefore, substituting into the process equation gives:
nRTVT3=C(where C is a constant)
Simplifying this, we get:
nRT4V=C
Rearranging gives:
V=nRT4C
3. Differentiate Volume with Respect to Temperature:
To find dVdT, we differentiate V with respect to T:
V=nRCT4
Thus,
dVdT=nRC⋅4T3
4. Substituting into the Coefficient of Thermal Expansion:
Now, substituting dVdT back into the expression for γ:
γ=1VdVdT=1nRCT4⋅(nRC⋅4T3)
Simplifying this gives:
γ=4T3T4=4T
5. Identifying n:
We are given that γ=nT. From our calculation, we have:
γ=4T
Therefore, comparing both expressions:
nT=4T
This leads us to conclude:
n=4
Final Answer:
n=4
The coefficient of thermal expansion γ is defined as:
γ=1VdVdT
From the given process PT3=constant, we can express pressure P in terms of volume V and temperature T. Using the ideal gas law PV=nRT, we can write:
P=nRTV
Therefore, substituting into the process equation gives:
nRTVT3=C(where C is a constant)
Simplifying this, we get:
nRT4V=C
Rearranging gives:
V=nRT4C
To find dVdT, we differentiate V with respect to T:
V=nRCT4
Thus,
dVdT=nRC⋅4T3
Now, substituting dVdT back into the expression for γ:
γ=1VdVdT=1nRCT4⋅(nRC⋅4T3)
Simplifying this gives:
γ=4T3T4=4T
We are given that γ=nT. From our calculation, we have:
γ=4T
Therefore, comparing both expressions:
nT=4T
This leads us to conclude:
n=4
n=4
Topper's Solved these Questions
Similar Questions
Knowledge Check
An ideal gas is taken through a process PT3= constant. The coefficient of thermal expansion of the gas in the given process is
A1/TB2/TC3/TD4/TAn ideal gas is expanded such that PT2=a constant. The coefficient of volume expansion of the gas is
A1TB2TC3TD4TAn ideal gas is expanding such that PT2=constant. The coefficient of volume expansion of lthe gas is:
A1TB2TC3TD4T
If PT3=Constant then find the coefficient of volume expansion.
View SolutionIdeal gas is taken through process shown in figure
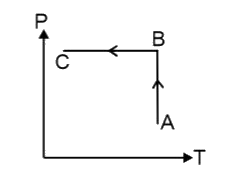
View SolutionAn ideal gas is expanding such that PT∘=constant. The coefficient of colume expansion of the gas is-
View SolutionIdeal gas is taken through process shown in figure :
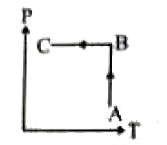
View SolutionIdeal gas is taken through the process shown in the figure :
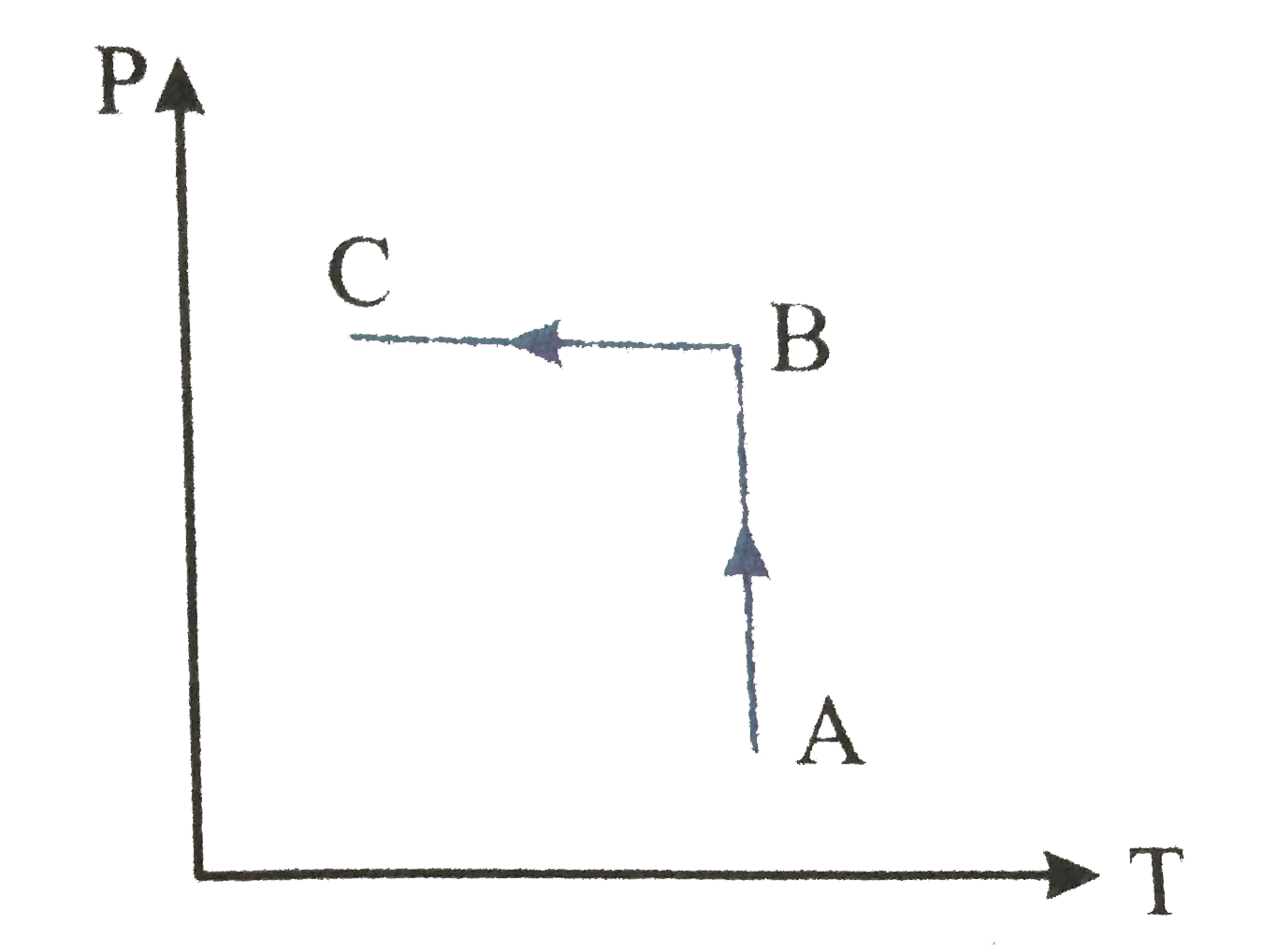
View Solution