There are two nuclei X and Y . |B.E of X | is a and |B.E of Y| is 2a. Also, |B.E per nucleon of X | is 2b and |B.E per nucleon of Y| is b. Then which one of the following is true ?
X is always more stable than Y
X is more stable than Y if mass number of X is greater than that of Y .
Y is always more stable than X
none of the above
The correct Answer is:A
To solve the problem, we need to analyze the given data about the two nuclei, X and Y, in terms of their binding energies and binding energies per nucleon.
1. Identify the Binding Energies:
- The binding energy of nucleus X is given as B.EX=a.
- The binding energy of nucleus Y is given as B.EY=2a.
2. Identify the Binding Energies per Nucleon:
- The binding energy per nucleon of nucleus X is given as B.Epernucleon,X=2b.
- The binding energy per nucleon of nucleus Y is given as B.Epernucleon,Y=b.
3. Understanding Stability:
- The stability of a nucleus is generally determined by its binding energy per nucleon. A higher binding energy per nucleon indicates a more stable nucleus.
4. Compare Binding Energies per Nucleon:
- For nucleus X: B.Epernucleon,X=2b.
- For nucleus Y: B.Epernucleon,Y=b.
- Since 2b>b, this means that nucleus X has a higher binding energy per nucleon than nucleus Y.
5. Conclusion on Stability:
- Since nucleus X has a higher binding energy per nucleon, it is more stable than nucleus Y.
6. Final Answer:
- Therefore, the correct statement is: "X is always more stable than Y."
- The binding energy of nucleus X is given as B.EX=a.
- The binding energy of nucleus Y is given as B.EY=2a.
- The binding energy per nucleon of nucleus X is given as B.Epernucleon,X=2b.
- The binding energy per nucleon of nucleus Y is given as B.Epernucleon,Y=b.
- The stability of a nucleus is generally determined by its binding energy per nucleon. A higher binding energy per nucleon indicates a more stable nucleus.
- For nucleus X: B.Epernucleon,X=2b.
- For nucleus Y: B.Epernucleon,Y=b.
- Since 2b>b, this means that nucleus X has a higher binding energy per nucleon than nucleus Y.
- Since nucleus X has a higher binding energy per nucleon, it is more stable than nucleus Y.
- Therefore, the correct statement is: "X is always more stable than Y."
Topper's Solved these Questions
- PHYSICS PART-IIIBOOK - FIITJEECHAPTER - PHYSICS PART-IIIEXERCISE - MCQ (MULTIPLE CORRECT)
18 Videos
- PHYSICS PART-IIIBOOK - FIITJEECHAPTER - PHYSICS PART-IIIEXERCISE - ASSERTION -REASONING MCQ (SINGLE CORRECT)
12 Videos
- PHYSICS PART-IIIBOOK - FIITJEECHAPTER - PHYSICS PART-IIIEXERCISE - ASSIGNMENT SECTION-II SUBJECTIVE
27 Videos
- OPTICSBOOK - FIITJEECHAPTER - OPTICSEXERCISE - NUMERICAL BASED QUESTIONS
2 Videos
- PHYSICS PART2BOOK - FIITJEECHAPTER - PHYSICS PART2EXERCISE - Numerical Based Question Decimal Type
6 Videos
Similar Questions
Knowledge Check
Assertion: Natural radioactive nuclei are nuclei of high mass number.
Reason: The B.E. per nucleon of heavy nuclei is large as compared to that of the stable nuclei.AAssertion is True, Reason is True, Reason is a correct explanation for Assertion.BAssertion is True, Reason is True, Reason is not a correct explanation for Assertion.CAssertion is True, Reason is false.DAssertion is False, but, Reason is true.The differential equation of y=Ae5x+Be−5x is
Ad2ydx2=25yBd2ydx2=−25yCd2ydx2=5yDyd2ydx2=−5y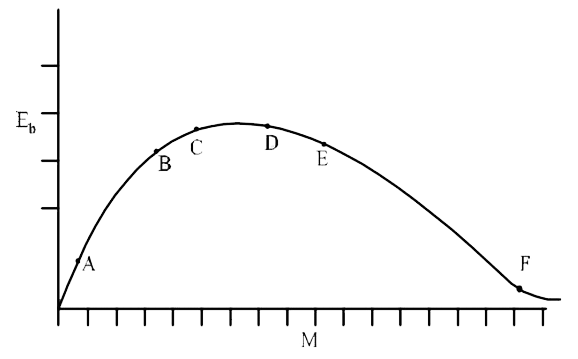
The above is a plot of binding energy per nucleon Eb against the nuclear mass M,A,B,C,D,E,F correspond to different nuclei Consider four reactions:AA+B→C+εBC→A+B+εCD+E→F+εandDF→D+E+ε,
If A=x% of y and B=y% of x,then which of the following is true? a.A is smaller than B b.A is greater than B. c.Relationship between A and B cannot be determined d.If x is smaller than y, then A is greater than B e.None of these
View SolutionLet A and B be two sets such that n(A)=3 and n(B)=2.if (x,1), (y,2), (z,1)are ∈ A×B, find A and B, where x,y,z are distinct elements.
View SolutionXY isa line parallel to side BC of ABC.BEA and CFAB meet XY in E and F respectively.Show that ar(ABE)=ar(ACF).
View SolutionIf y=e−x(Acosx+Bsinx) then y is a situation of
View SolutionTwo sets A and B are are defined as follows
A={(x,y):y =e2x,x∈R} and
B = {(x,y):y=x^3 , x in R}, then
View Solution
