Show that the loss of kinetic energy occurs in an inelastic collision . How will you justify this loss of energy ?
To show that the loss of kinetic energy occurs in an inelastic collision, we can analyze the situation using the principles of conservation of momentum and the definition of kinetic energy.
1. Define the Initial Conditions:
- Let the mass of the first body be m1 and its initial velocity be u1.
- Let the mass of the second body be m2 and its initial velocity be u2=0 (the second body is at rest).
2. Apply Conservation of Momentum:
- The total initial momentum of the system is given by:
pinitial=m1u1+m2u2=m1u1
- After the collision, let the final velocities of the two bodies be v1 and v2. The total final momentum is:
pfinal=m1v1+m2v2
- According to the conservation of momentum:
m1u1=m1v1+m2v2(1)
3. Define the Coefficient of Restitution:
- The coefficient of restitution e for an inelastic collision is defined as:
e=Relative velocity of separationRelative velocity of approach=v2−v1u1−u2
- Since u2=0, this simplifies to:
e=v2−v1u1(2)
4. Express Velocities after Collision:
- Rearranging equation (2) gives:
v2−v1=eu1⇒v2=v1+eu1(3)
5. Substitute Equation (3) into Equation (1):
- Substitute v2 from equation (3) into equation (1):
m1u1=m1v1+m2(v1+eu1)
- Simplifying this gives:
m1u1=(m1+m2)v1+m2eu1
- Rearranging it to solve for v1:
v1=m1u1−m2eu1m1+m2(4)
6. Calculate Initial and Final Kinetic Energies:
- The initial kinetic energy KEi is:
KEi=12m1u21
- The final kinetic energy KEf is:
KEf=12m1v21+12m2v22
- Substitute v1 and v2 from equations (3) and (4) into this expression.
7. Show that KEf<KEi:
- After substituting and simplifying, we find that the final kinetic energy is less than the initial kinetic energy:
KEf<KEi
- This indicates that some kinetic energy has been lost during the inelastic collision.
8. Justification of Energy Loss:
- The loss of kinetic energy in an inelastic collision can be justified by the fact that some of the kinetic energy is converted into other forms of energy, such as heat, sound, and deformation of the colliding bodies. This energy is not recoverable as kinetic energy, which is why the final kinetic energy is less than the initial kinetic energy.
- Let the mass of the first body be m1 and its initial velocity be u1.
- Let the mass of the second body be m2 and its initial velocity be u2=0 (the second body is at rest).
- The total initial momentum of the system is given by:
pinitial=m1u1+m2u2=m1u1
- After the collision, let the final velocities of the two bodies be v1 and v2. The total final momentum is:
pfinal=m1v1+m2v2
- According to the conservation of momentum:
m1u1=m1v1+m2v2(1)
- The coefficient of restitution e for an inelastic collision is defined as:
e=Relative velocity of separationRelative velocity of approach=v2−v1u1−u2
- Since u2=0, this simplifies to:
e=v2−v1u1(2)
- Rearranging equation (2) gives:
v2−v1=eu1⇒v2=v1+eu1(3)
- Substitute v2 from equation (3) into equation (1):
m1u1=m1v1+m2(v1+eu1)
- Simplifying this gives:
m1u1=(m1+m2)v1+m2eu1
- Rearranging it to solve for v1:
v1=m1u1−m2eu1m1+m2(4)
- The initial kinetic energy KEi is:
KEi=12m1u21
- The final kinetic energy KEf is:
KEf=12m1v21+12m2v22
- Substitute v1 and v2 from equations (3) and (4) into this expression.
- After substituting and simplifying, we find that the final kinetic energy is less than the initial kinetic energy:
KEf<KEi
- This indicates that some kinetic energy has been lost during the inelastic collision.
- The loss of kinetic energy in an inelastic collision can be justified by the fact that some of the kinetic energy is converted into other forms of energy, such as heat, sound, and deformation of the colliding bodies. This energy is not recoverable as kinetic energy, which is why the final kinetic energy is less than the initial kinetic energy.
Topper's Solved these Questions
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Revision Exercise (Numerical Problems)
23 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Objective Type Questions (A. Multiple Choice Questions)
29 Videos
- WORK, ENERGY AND POWER BOOK - MODERN PUBLICATIONCHAPTER - WORK, ENERGY AND POWER EXERCISE - Revision Exercise (Short Answer Questions)
16 Videos
- WAVESBOOK - MODERN PUBLICATIONCHAPTER - WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
Knowledge Check
The loss of kinetic energy during the above proces is
AIω22BIω23CIω24DIω26In an inelastic collision, the kinetic energy after collision
Ais same as before collisionBis always less than before collisionCis always greater than before collisionDmay be less or greater than before collision
In what is the loss in kinetic energy of the system due to impact ?
View SolutionExplain the flow and loss of energy in an ecosystem.
View SolutionWhich of the following statements is false for collisions-
(1)Momentum is conserved in elastic collisions but not in inelastic collisions.
(2)Total-kinetic energy is conserved in elastic collisions but momentum is not conserved.
(3)Total kinetic energy and momentum both are conserved in all types of collisions
(4)Total kinetic energy is not conserved in inelastic collisions but momentum is conserved
View SolutionA block moves with a certain speed and collides with another identical block kept at rest . There is maximum possible loss of kinetic energy in this collision . Find the ratio initial kinetic energy of the sustem to that with final kinetic enegy of the system .
View SolutionHow do we control AC without much loss of energy?
View SolutionFig shows that energy levels of a mercury atom. Electrons with kinetic energies of 7.0 eV are introduced into mercury vapor. (a) Convert the energy levels to the system that sets the ground state at 0 eV. (b) What is the kinetic energy of an electron after an elastic collision with a mercury atom? (c ) What are the possible kinetic energies of an electron after an inelastic collision with a mercury atom? (d) What are the energies of the photons coming from the mercury vapor?
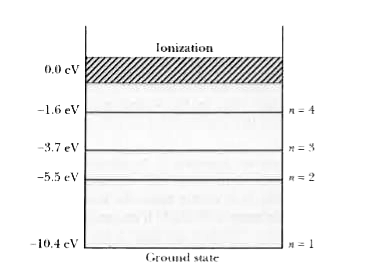
View Solution
