An equilateral glass prism of refractive index 1.5 is immersed in water. Find the angle of deviation produced when a ray of light is incident at 42∘ on one face of the prism.
(Refractive index of water = 1.33)
To solve the problem of finding the angle of deviation produced when a ray of light is incident at 42∘ on one face of an equilateral glass prism immersed in water, we can follow these steps:
Step 1: Identify Given Values
- Refractive index of glass prism (μg) = 1.5
- Refractive index of water (μw) = 1.33
- Angle of the prism (A) = 60° (since it is an equilateral prism)
- Angle of incidence (i) = 42°
Step 2: Apply Snell's Law at the First Face
Using Snell's Law at the first face of the prism:
μw⋅sin(i)=μg⋅sin(r)
Substituting the known values:
1.33⋅sin(42∘)=1.5⋅sin(r)
Step 3: Calculate sin(r)
First, calculate sin(42∘):
sin(42∘)≈0.6691
Now substituting this value into the equation:
1.33⋅0.6691=1.5⋅sin(r)
0.8905=1.5⋅sin(r)
sin(r)=0.89051.5≈0.5937
Step 4: Find Angle of Refraction r
Now, find r using the inverse sine function:
r=sin−1(0.5937)≈36.16∘
Step 5: Calculate Angle of Emergence r′
Using the relation for the angles in the prism:
r+r′=A
So,
r′=A−r=60∘−36.16∘≈23.84∘
Step 6: Apply Snell's Law at the Second Face
Now apply Snell's Law at the second face of the prism:
μg⋅sin(r′)=μw⋅sin(e)
Substituting the known values:
1.5⋅sin(23.84∘)=1.33⋅sin(e)
Step 7: Calculate sin(e)
First, calculate sin(23.84∘):
sin(23.84∘)≈0.4046
Now substituting this value into the equation:
1.5⋅0.4046=1.33⋅sin(e)
0.6069=1.33⋅sin(e)
sin(e)=0.60691.33≈0.456
Step 8: Find Angle of Emergence e
Now, find e using the inverse sine function:
e=sin−1(0.456)≈27.18∘
Step 9: Calculate the Angle of Deviation δ
The angle of deviation δ is given by:
δ=i+e−A
Substituting the known values:
δ=42∘+27.18∘−60∘
δ≈9.18∘
Final Answer
The angle of deviation produced is approximately 9.18∘.
---
- Refractive index of glass prism (μg) = 1.5
- Refractive index of water (μw) = 1.33
- Angle of the prism (A) = 60° (since it is an equilateral prism)
- Angle of incidence (i) = 42°
Using Snell's Law at the first face of the prism:
μw⋅sin(i)=μg⋅sin(r)
Substituting the known values:
1.33⋅sin(42∘)=1.5⋅sin(r)
First, calculate sin(42∘):
sin(42∘)≈0.6691
Now substituting this value into the equation:
1.33⋅0.6691=1.5⋅sin(r)
0.8905=1.5⋅sin(r)
sin(r)=0.89051.5≈0.5937
Now, find r using the inverse sine function:
r=sin−1(0.5937)≈36.16∘
Using the relation for the angles in the prism:
r+r′=A
So,
r′=A−r=60∘−36.16∘≈23.84∘
Now apply Snell's Law at the second face of the prism:
μg⋅sin(r′)=μw⋅sin(e)
Substituting the known values:
1.5⋅sin(23.84∘)=1.33⋅sin(e)
First, calculate sin(23.84∘):
sin(23.84∘)≈0.4046
Now substituting this value into the equation:
1.5⋅0.4046=1.33⋅sin(e)
0.6069=1.33⋅sin(e)
sin(e)=0.60691.33≈0.456
Now, find e using the inverse sine function:
e=sin−1(0.456)≈27.18∘
The angle of deviation δ is given by:
δ=i+e−A
Substituting the known values:
δ=42∘+27.18∘−60∘
δ≈9.18∘
The angle of deviation produced is approximately 9.18∘.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (1)
13 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (2)
9 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
- SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
12 Videos
Similar Questions
An equilateral glass prism (μ−1.6) is immersed in water (μ=1.33). Calculate the angle of deviation produced for a ray of light incident at 40∘ on one face of the prism.
View SolutionAn equilateral glass prism is made of a material of refractive index 1.500. Find its angle of minimum deviation.
View Solution
Knowledge Check
When a ray of light is incident normally on one refracting surface of an equilateral prism of refractive index 1.5, then
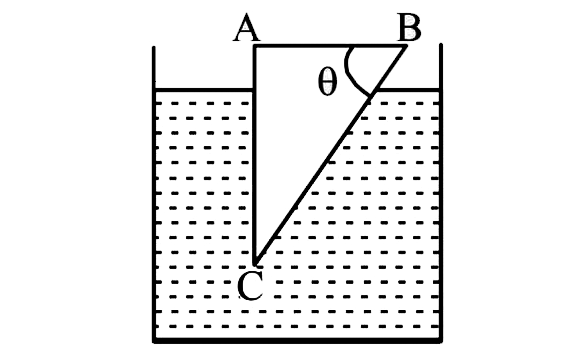
Athe ray undergoes total internal reflection at the second refracting surface of prismBemergent ray is deviated by 30∘Cemergent ray is deviated by 60∘Demergent ray just grazes the second refracting surface of the prismA glass prism of refractive index 1.5 is immersed in water (refractive index 4/3). A light beam incident normally on the face AB is totally reflected to reach on the face BC if
 Asinθ≥89B23<sinθ<89C12<sinθ<89DNone of these
Asinθ≥89B23<sinθ<89C12<sinθ<89DNone of theseA glass prism of refractive index 1.5 is immersed in water (refractive index 4/3). A light beam incident normally on the face AB is totally reflected to reach on the face BC if.
 Asinθ≥89B23<sinθ<89Csinθ≤23DNone of these
Asinθ≥89B23<sinθ<89Csinθ≤23DNone of these
An equilateral glass prism has a refractive index 1.6 in air. Calculate the angle of minimum deviation of the prism, when kept in a medium of refractive index 4√2/5
View SolutionA prism of refractive index √2 has refractive angle 60∘ . Find the angle of incidence so that a ray minimum deviation.
View SolutionWhen a ray of light is incident normally on one refracting surface of an equilateral prism (Refractive index of the material of the prism =1.5
View SolutionChoose the most appropriate option.
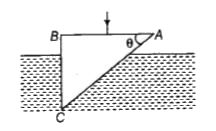
A glass prism ABC (refractive index 1.5), immersed in water (refractive index 4/3). A ray of light is incidentally normally on face AB. If it is totally reflected at face AC, then
View SolutionA glass prism of refractive index 1.5 is immersed in water (refractive index 4/3). A light beam incident normally on the face AB is totally reflected to reach the face BC, Fig. if :
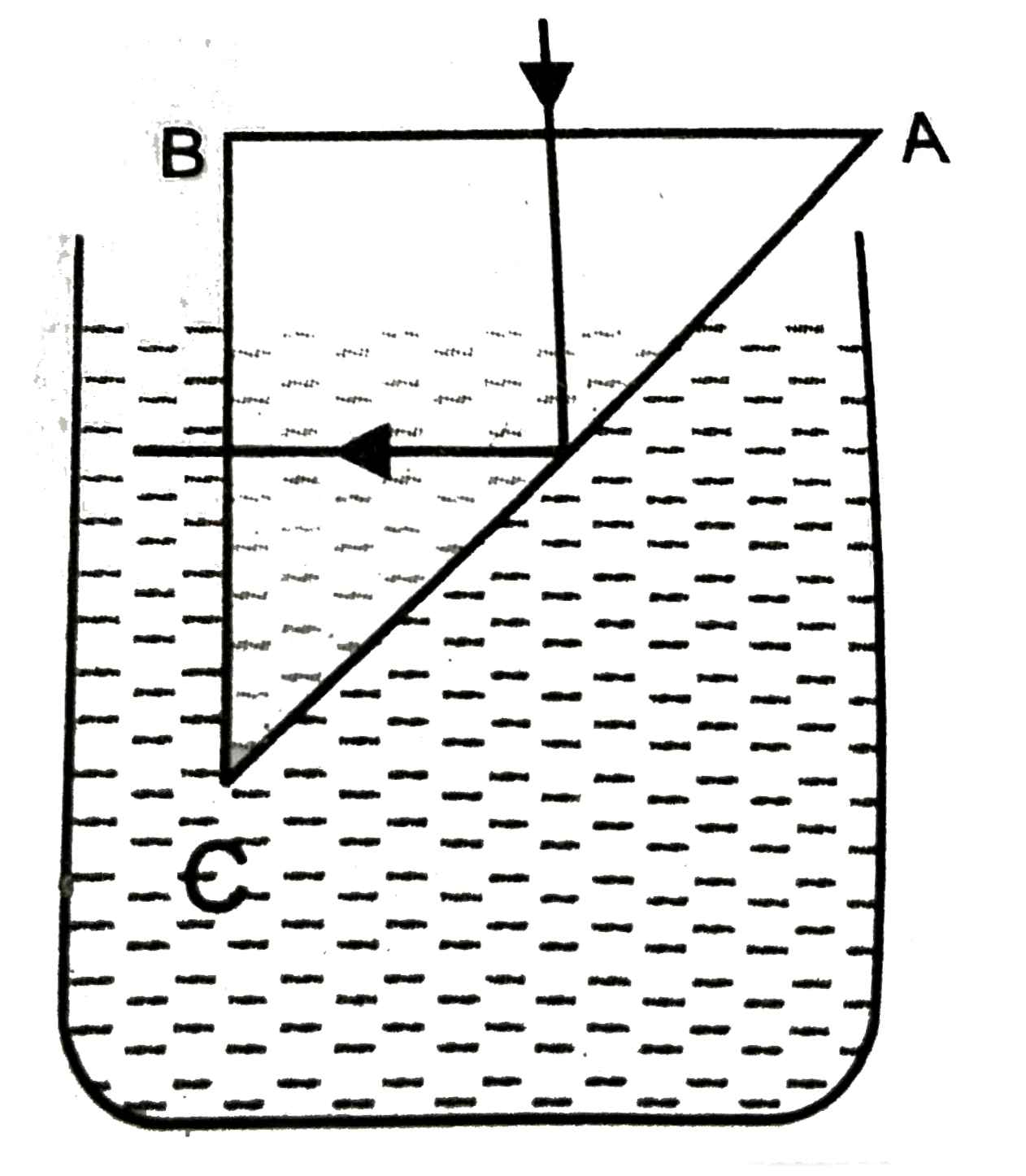 .
.
View Solution
