A convex lens has a focal length of 15 cm. Determine the focal length and nature of the lens which can be used with the convex lens to obtain a combination with effective power 9 diopter.
To solve the problem, we need to determine the focal length and nature of a lens that can be combined with a convex lens of focal length 15 cm to achieve an effective power of 9 diopters.
1. Understand the relationship between power and focal length:
The power P of a lens is given by the formula:
P=1f
where f is the focal length in meters. For a lens in centimeters, the formula becomes:
P=100f
2. Calculate the power of the convex lens:
Given that the focal length of the convex lens f1=15 cm, we can calculate its power:
P1=10015≈6.67 diopters
3. Set up the equation for effective power:
The effective power Peq of a combination of lenses is the sum of their individual powers:
Peq=P1+P2
Given Peq=9 diopters, we can substitute P1:
9=6.67+P2
4. Solve for the power of the second lens:
Rearranging the equation gives:
P2=9−6.67=2.33 diopters
5. Determine the focal length of the second lens:
Using the power formula again, we can find the focal length f2 of the second lens:
P2=100f2
Thus,
2.33=100f2
Rearranging gives:
f2=1002.33≈42.92 cm
6. Determine the nature of the second lens:
Since P2 is positive, the second lens is also a convex lens.
Final Answer:
The focal length of the second lens is approximately 42.92 cm, and it is a convex lens.
---
The power P of a lens is given by the formula:
P=1f
where f is the focal length in meters. For a lens in centimeters, the formula becomes:
P=100f
Given that the focal length of the convex lens f1=15 cm, we can calculate its power:
P1=10015≈6.67 diopters
The effective power Peq of a combination of lenses is the sum of their individual powers:
Peq=P1+P2
Given Peq=9 diopters, we can substitute P1:
9=6.67+P2
Rearranging the equation gives:
P2=9−6.67=2.33 diopters
Using the power formula again, we can find the focal length f2 of the second lens:
P2=100f2
Thus,
2.33=100f2
Rearranging gives:
f2=1002.33≈42.92 cm
Since P2 is positive, the second lens is also a convex lens.
The focal length of the second lens is approximately 42.92 cm, and it is a convex lens.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (8)6videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (9)6videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - PRACTICE PROBLEMS (6)3videos
RAY OPTICS AND OPTICAL INSTRUMENTS
- BOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION15videos
NUCLEI
- BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION12videos
SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS
Similar Questions
Knowledge Check
- Question 1 - Select One
A convex lens is of focal length 10 cm. What is its power?
A+10DB+5DC−10DD+1D - Question 2 - Select One
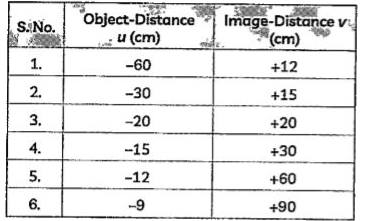
The focal length of the convex lens isA+10 cmB+20cmC−10cmD−20cm - Question 3 - Select One
A convex lens of focal length 0.5 m and concave lens of focal length 1 m are combined. The power of the resulting lens will be
A1 DB−1DC0.5DD−0.5D
The focal length of a convex lens is
View SolutionA thin lens has a focal length of −25cm. What is the power of the lens? It is convex or concave ?
View SolutionTwo convex lenses of focal length 10 cm each are combined. What will be the focal length of the combined lens ?
View SolutionA concave lens of focal length 12 cm and a convex lens of focal length 20 cm are kept in contact with each other .
Find the focal length of the combination .View SolutionA convex lens is coaxially placed with a concave lens. A screen is placed at a distance of 100 cm apart from the combination. The focal length of convex lens is 15 cm. Determine the focal length of the concave lens if image of object placed at a distance of 20 cm, from the combination, is projected on the scrcen.
View Solution

