There is one cylindrical vessel whose height and diameter are both equal to D. An observer's eye is placed in such a manner that bottom edge of the vessel is just visible to eye.
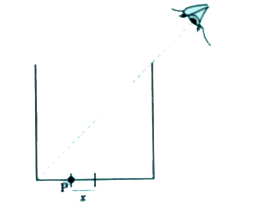
There is a point P at the bottom of container at a distance x from the centre of container as shown in the figure. Up to what height the liquid of refractive index μ should be poured in the vessel so that point P is visible to the eye?
From the figure given below we can understand that he need to put liquid up to a height so that after refraction from liquid up to a height so that after refraction from liquid light ray from point P is refracted along the line of vision . Line of vision of eye is at 45∘ because we know that height and diameter of vessel both are equal to D . Let h be the height of liquid poured.
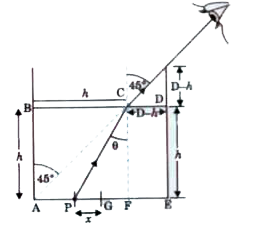
We can see in the figure that the angle of incidence of light ray from point P is equal to θ. Let us apply Snell.s law at point C in the diagram .
μsinθ=sin45∘
sinθ=1μ√2 ...(i)
Further from the figure we can write the following :
PF = PE - FE
⇒PF=(D/2+x)−(D−h)
⇒PF=x+h−D/2...(ii)
Further from the figure we can write the following :
tanθ=PFCF
⇒tanθ=x+h−D2h ...(iii)
Now from equation (i) we can write the following :
tanθ=1√2μ2−1 ...(iv)
From equation (iii) and (iv) we can write the following :
From equation (iii) and (iv) we can write the following :
x+h−D2h=1√2μ2−1
⇒ 2x+2h−D2h=1√2μ2−1
⇒(2x−D)(√2μ2−1)+2h(√2μ2−1)=2h
⇒(2x−D)(√2μ2−1)=2h(1−√2μ2−1)
⇒h=(2x−D)(√2μ2−1)2(1−√2μ2−1)
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - NCERT FILE SOLVED (TEXT BOOK EXERCISES)
38 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - NCERT (EXemplar Problems Obejective Questions) (Multiple Choice Questions (Type-I)
10 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - MODERN PUBLICATIONCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - Conceptual Questions
40 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
- SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
12 Videos
Similar Questions
A rectangular vessel of dimension (l×b×h) and mass M contains a liquid of density ρ. The vessel has an orifice at its bottom at a distance c from the rear wall as shown in the figure.

View SolutionA cylindrical vessel, whose diameter and height both are equal to 30 cm, is placed on a horizontal surface and a small particla P is placed in it at a distance of 5.0 cm from the center. An eye is placed at a position such that the edge of the bottom is just visible. The particl P is in the plane of drawing. Up to what minimum height should water be poured in the vessel to make the particle P visible?
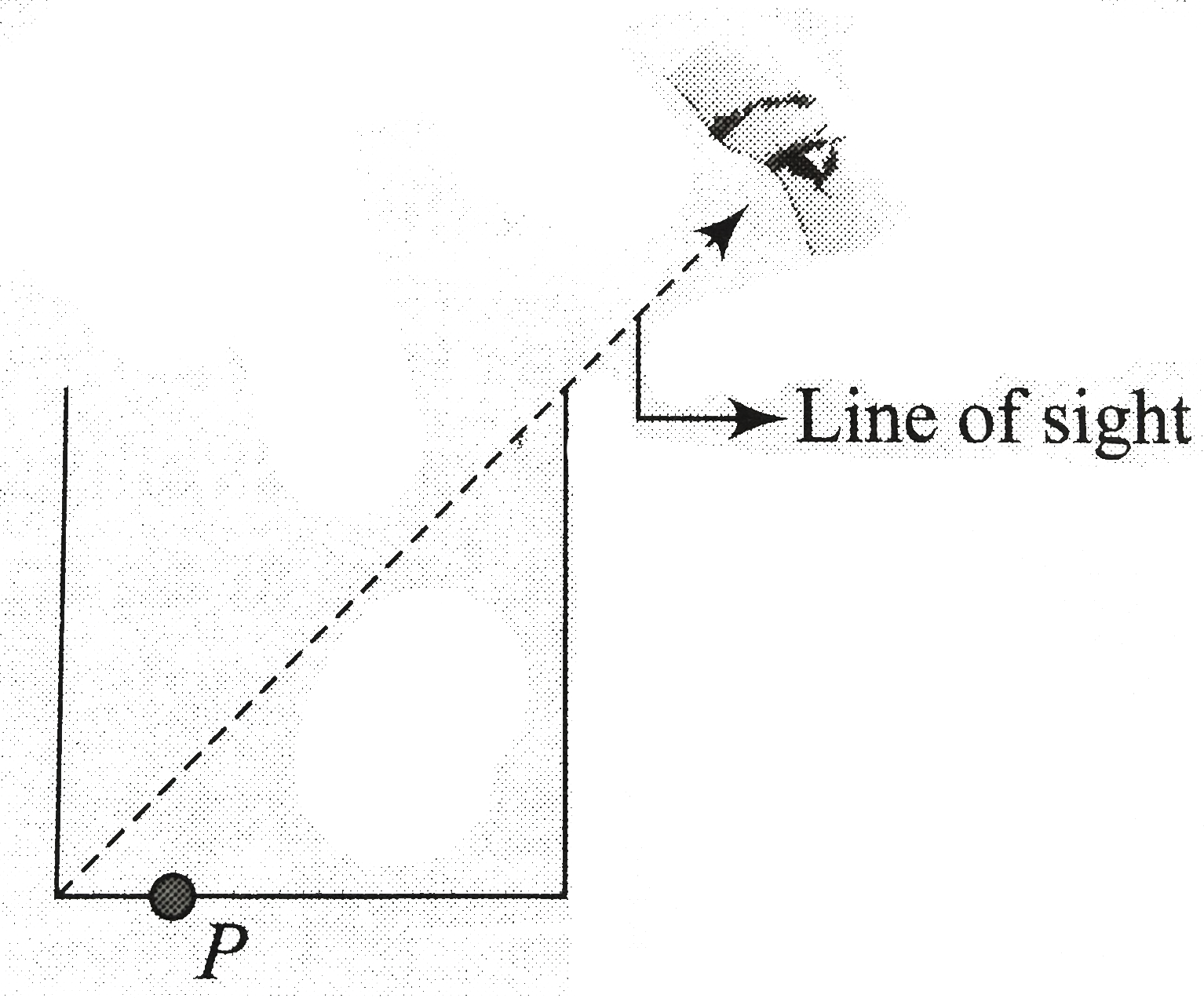
View Solution
Knowledge Check
A rectangular vessel of dimension (l×b×h) and mass M contains a liquid of density ρ. The vessel has an orifice at its bottom at a distance c from the rear wall as shown in the figure.
 AThe maximum volume of the water that can be stored when the vessel is accelerated is hcb/2BThe maximum volume of the water that can be stored when the vessel is accelerated is hlb/2CForce F that must he applied when maximum water stored is [M+hcbρ2]hgcDForce F that must be applied when maximum water stored is [M+hcbρ2]lgc
AThe maximum volume of the water that can be stored when the vessel is accelerated is hcb/2BThe maximum volume of the water that can be stored when the vessel is accelerated is hlb/2CForce F that must he applied when maximum water stored is [M+hcbρ2]hgcDForce F that must be applied when maximum water stored is [M+hcbρ2]lgcThe height to which a cylindrical vessel be filled with a homogenous liquid, to make the average force with which the liquid presses the side of the vessel equal to the force exerted by the liquid on the bottom of the vessel, is equal to.
Ahalf of the radius of the vesselBradius of the vesselCone-fourth of the radius of the vesselDthree-fourth of the radius of the vessel.There is a hole of area 125cm2 in the bottom of a cylindrical vessel containing fluid up to height h. The liquid flows out in time t. If the liquid were filled in the vessel up to height 4h, then it would flow out in time
AtB2tC4tDt2
If an observer sees the bottom of the vessel shown in Figure., at 8cm, find the refractive index of the medium in which the observer is present.

View SolutionIf an observer sees the bottom of the vessel shown in Figure., at 8cm, find the refractive index of the medium in which the observer is present.

View SolutionA cylindrical vessel, whose diameter and height both are equal to 30 cm, is placed on a horizontal surface and a small particle P is placed in it at a distance of 5.0 cm from the centre. An eye is placed at a position such that the edge of the bottom is just visible (see figure 18-E8). The particle P is in the plane of drawing. Up to what minimum height should water be poured in the vessel to make the particle P visible ?

View SolutionA cylindrical vessel, whose diameter and height both are equal to 30 cm, is placed on a horizontal surface and a small particle P is placed in it at a distance of 5.0 cm from the centre. An eye is placed at a position such that the edge of the bottom is just visible (see figure 18-E8). The particle P is in the plane of drawing. Up to what minimum height should water be poured in the vessel to make the particle P visible ?

View SolutionA tank, which is open at the top, contains a liquid up to a height H. A small hole is made in the side of the tank at a distance y below the liquid surface. The liquid emerging from the hole lands at a distance x from the tank :

View Solution
