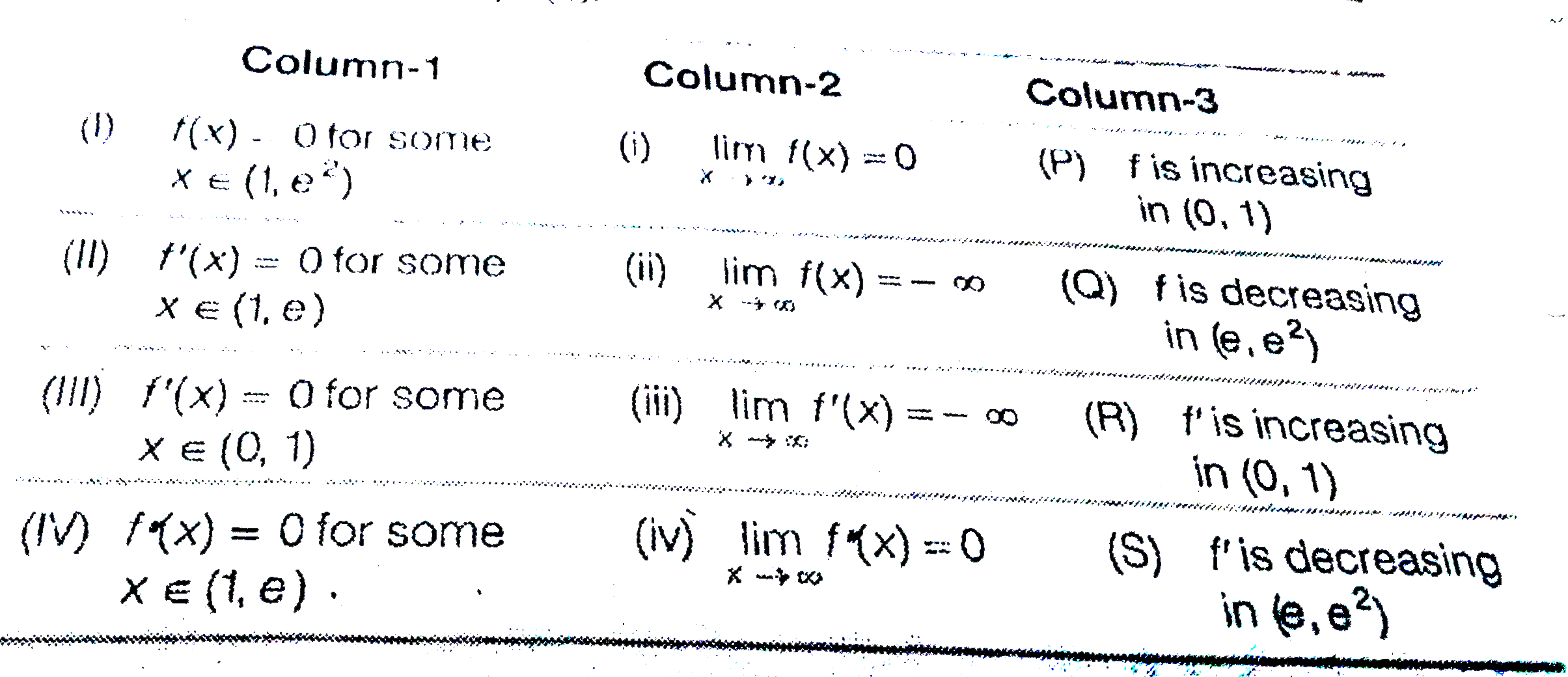
Which of the following options is the only INCORRECT combination ?
Video Solution
Text Solution
The correct Answer is:C
f(x)=x+In x−x In x
f(1)=1>0
f(e2)=e2+2−2e2=2−e2<0
⇒f(x)=0 for some x∈(1,e2)
∴ I is correct
f'(x)=1+1x−Inx−1=1x−Inx
f'(x)>0for (0,1)
f'(x)<0 for (e,∞)
∴PandQ are correct , II is correct, III is incorrect.
f''(x)=−1x2−1x
∴S, is correct, R is incorrect.
IV is incorrect.
limx→∞f(x)=−∞
limx→∞f'(x)=−∞
limx→∞f''(x)=0
∴ ii, iii, iv are correct.
|
Topper's Solved these Questions
- APPLICATION OF DERIVATIVES BOOK - IIT JEE PREVIOUS YEARCHAPTER - APPLICATION OF DERIVATIVES EXERCISE - RATE MEASURE, INCREASING AND DECREASING FUNCTIONS (ANALYTICAL & DESCRIPTIVE QUESTIONS)
7 Videos
- APPLICATION OF DERIVATIVES BOOK - IIT JEE PREVIOUS YEARCHAPTER - APPLICATION OF DERIVATIVES EXERCISE - ROLLES AND LAGRANGES THEOREM (OBJECTIVE QUESTIONS I)
1 Videos
- APPLICATION OF DERIVATIVES BOOK - IIT JEE PREVIOUS YEARCHAPTER - APPLICATION OF DERIVATIVES EXERCISE - RATE MEASURE, INCREASING AND DECREASING FUNCTIONS (FILL IN THE BLANKS )
2 Videos
- 3D GeometryBOOK - IIT JEE PREVIOUS YEARCHAPTER - 3D GeometryEXERCISE - Equation of Plane Objective Question II (Integer Answer Type Question)
1 Videos
- AREABOOK - IIT JEE PREVIOUS YEARCHAPTER - AREAEXERCISE - AREA USING INTEGRATION
55 Videos
Similar Questions
Knowledge Check
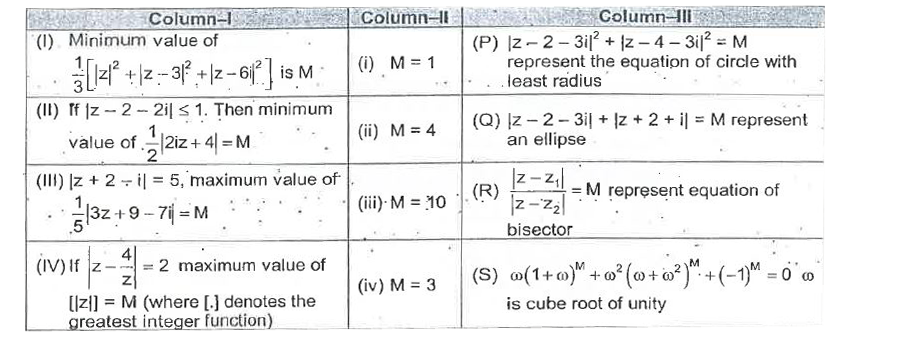
Which of the following options is the only CORRECT combination ?A(I) (ii) (S)B(I) (iii) (P)C(I) (iii) (Q)D(I) (ii) (Q)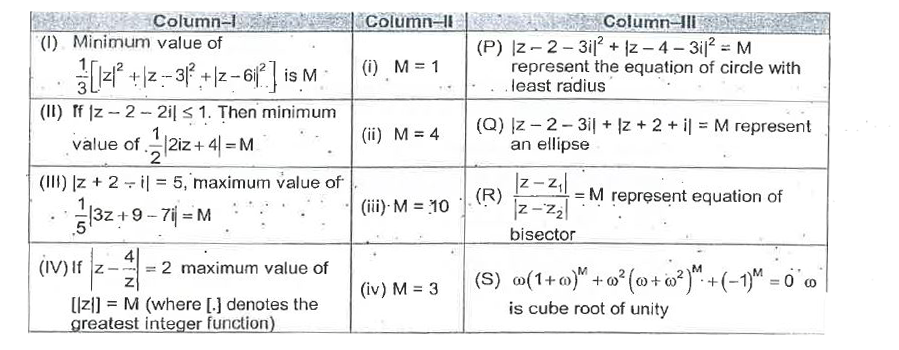
Which of the following options is the only CORRECT combination ?A(II) (ii) (P)B(II) (i) (R)C(II) (i) (S)D(II) (iv) (R)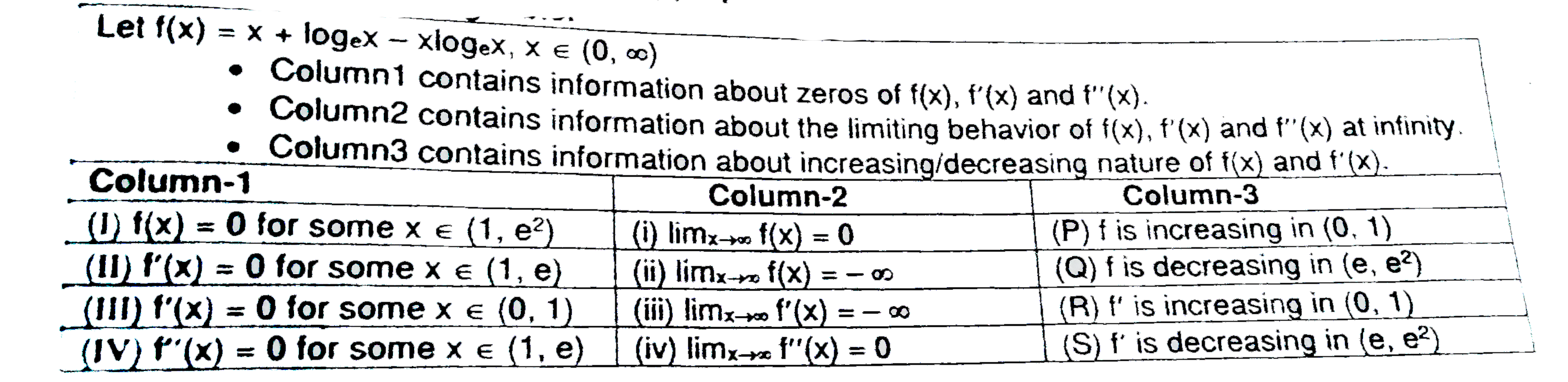
Which of the following options is the only CORRECT combination ?A(III)(iii)(R)B(IV)(iv)(S)C(II)(ii)(Q)D(I)(i)(P)

Which of the following options is the only CORRECT combination ?
View Solution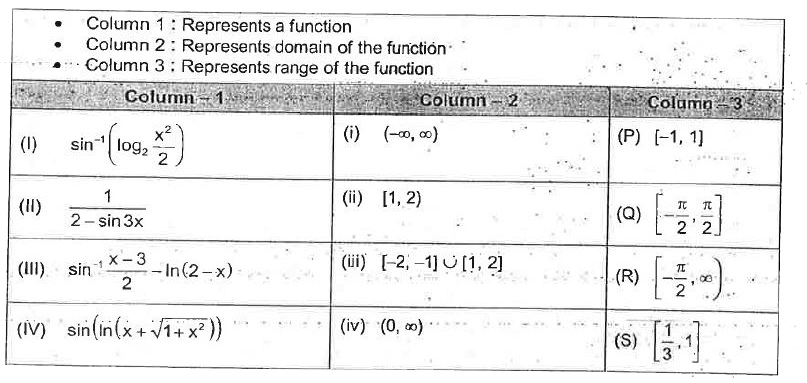
Which of the following options is the only CORRECT combination?
View Solution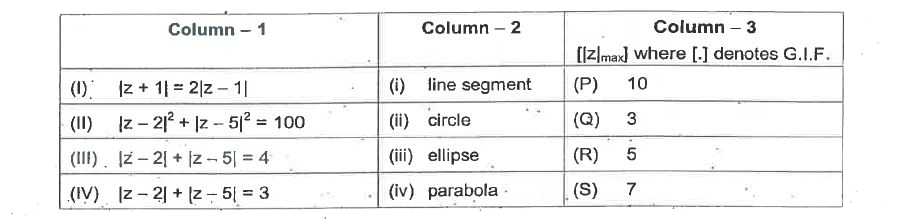
Which of the following options is the only CORRECT combination ?
View Solution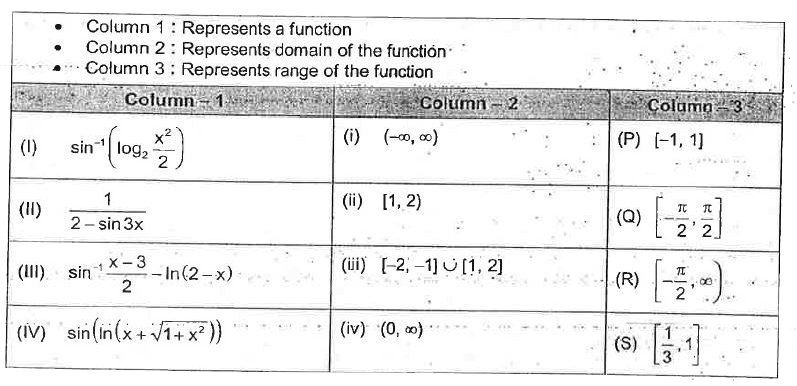
Which of the following options is the only CORRECT combination?
View Solution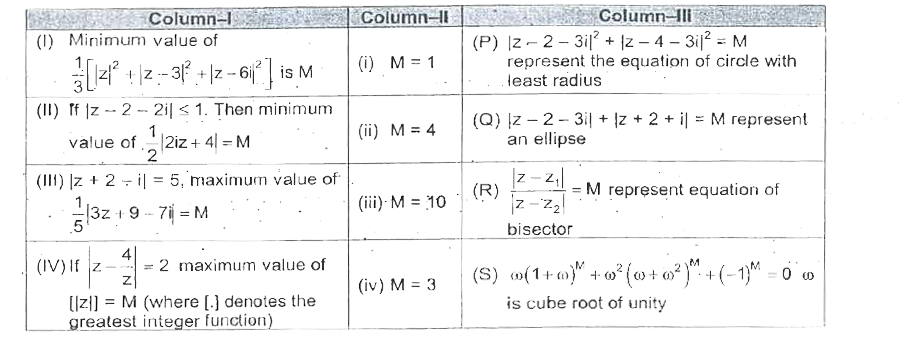
Which of the following options is the only CORRECT combination ?
View Solution


