క్రింది వానిని కనుగొనుము. a1=5, a4=9(12), అయిన a2,a3 లను కనుగొనుము.
Step by step video & image solution for క్రింది వానిని కనుగొనుము. a_1 =5, a_4 = 9(1/2), అయిన a_2, a_3 లను కనుగొనుము. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ఒక టాక్సీ మొదటి కి.మీ. ప్రయాణానికి Rs.20 ల చొప్పున, తరువాత ప్రతి కి,మీ.కు Rs.8 ల చొప్పున చెల్లించవలసిన, 15 కి.మీ. ప్రయాణానికి అయ్యే సొమ్ము Rs.....
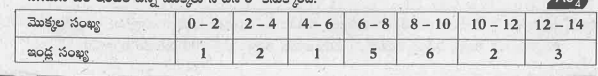
View Solutionఒక' గ్రామంలో కొంతమంది విద్యార్థుల జట్టు “పర్యావరణ పరిరక్షణ - అవగాహని అనే కార్యక్రమంలో భాగంగా, 20 ఇండ్లలో సర్వే నిర్వహించి, ఎన్నెన్ని మొక్కలు నాటినారో సమాచారాన్ని సేకరించి, ఈ క్రింది పట్టికలో నమోదు చేసినారు. సగటున ఒక ఇంటికి ఎన్ని మొక్కలు నాటినారో కనుక్కోండి.
View SolutionFOr an integer nlt2, we have real numbers a_1,a_2,a_3,a_4,..........,a_n such that a_2+a_3+a_4+.......+a_n=a1 and a_1+a_3+a_4+...........+a_n=a_2...............a_1+a_2+a_3+..............+a_(n-1)=a_n what is the value of a_1+a_2+a_3+........+a_n?
View SolutionIf a1,a2,a3,a4 are the coefficients of 2nd, 3rd, 4th and 5th terms of respectively in (1+x)n then a1a1+a2+a3a3+a4=
View SolutionIf a2a3a1a4=a2+a3a1+a4=3(a2−a3a1−a4) then a1,a2,a3,a4 are in :
View SolutionIf a1,a2,a3,a4 are the coefficients of 2nd, 3rd, 4th and 5th terms of (1+x)n respectively then a1a1+a2,a2a2+a3,a3a3+a4 are in
View SolutionIf a1,a2,a3,a4 be the coefficient of four consecutive terms in the expansion of (1+x)n, then prove that: a1a1+a2+a3a3+a4=2a2a2+a3.
View SolutionIf a1,a2,a3,a4 be the coefficient of four consecutive terms in the expansion of (1+x)n, then prove that: a1a1+a2+a3a3+a4=2a2a2+a3.
View SolutionThe average of a1,a2,a3,a4 is 16. Half of the sum of a2,a3,a4 is 23. What is the value of a1
View SolutionIf the coefficient of-four successive termsin the expansion of (1+x)n be a1,a2,a3and a4respectivel. Show thata1a1+a2+a3a3+a4=2a2a2+a3
View SolutionLet A be the set of 4-digit numbers a_1 a_2 a_3 a_4 where a_1 > a_2 > a_3 > a_4 , then n(A) is equal to
View SolutionIf a2a3a1a4=a2+a3a1+a4=3(a2−a3a1−a4) then a1,a2,a3,a4 are in :
View SolutionLet a1,a2,a3anda4 be in AP. If a1+a4=10anda2.a3=24, then the least term of the AP, is
View SolutionIf a1,a2,a3,a4 be the coefficient of four consecutive terms in the expansion of (1+x)n, then prove that: a1a1+a2+a3a3+a4=2a2a2+a3.
View SolutionProve that (a1a2+a3a4+a5a6)(a2a1+a4a3+a6a5)≥9
View SolutionLet A be the set of 4-digit numbers a_1 a_2 a_3 a_4 where a_1 > a_2 > a_3 > a_4 , then n(A) is equal to
View Solution
