ఒక పూలతోటలో మొదటి వరుసలో 23, రెందవ వరుసలో 21 మూడవ వరుసలో 19 మొక్కలు చొప్పున గలవు. ఈ తోటలో మొత్తం 10 వరుసలు గలవు. అయిన చివరి వరుసలో గల మొక్కల సంఖ్యను tn=a+(n−1)d సూత్రంతో కనుక్కోండి.
Step by step video & image solution for ఒక పూలతోటలో మొదటి వరుసలో 23, రెందవ వరుసలో 21 మూడవ వరుసలో 19 మొక్కలు చొప్పున గలవు. ఈ తోటలో మొత్తం 10 వరుసలు గలవు. అయిన చివరి వరుసలో గల మొక్కల సంఖ్యను t_n= a + (n- 1)d సూత్రంతో కనుక్కోండి. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ఒక టాక్సీ మొదటి కి.మీ. ప్రయాణానికి Rs.20 ల చొప్పున, తరువాత ప్రతి కి,మీ.కు Rs.8 ల చొప్పున చెల్లించవలసిన, 15 కి.మీ. ప్రయాణానికి అయ్యే సొమ్ము Rs.....
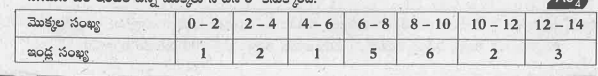
View Solutionఒక' గ్రామంలో కొంతమంది విద్యార్థుల జట్టు “పర్యావరణ పరిరక్షణ - అవగాహని అనే కార్యక్రమంలో భాగంగా, 20 ఇండ్లలో సర్వే నిర్వహించి, ఎన్నెన్ని మొక్కలు నాటినారో సమాచారాన్ని సేకరించి, ఈ క్రింది పట్టికలో నమోదు చేసినారు. సగటున ఒక ఇంటికి ఎన్ని మొక్కలు నాటినారో కనుక్కోండి.
View SolutionThe value of cot∑19n=1(cot−1(1+n∑p=12p)) is equal to (a) 2119 (b) 1921 (c) −1921 (d) −2119
View Solution-56-(-19)-21+23
View Solutiona భుజముగా గల ఘనము యొక్క ఘనపరిమాణమును కనుక్కోండి.
View SolutionS = {x,x అనేది 2n + 3, nవ పదంగా గల అంకథ్రేఢిలోని పదము, మరియు n<6) అయిన S=
View Solution'N between a and b in a GP పదాలు గలవు. అయిన ఆ GP యందు సామాన్య నిష్పత్తి r =........
View SolutionCenter of gravity (6,-1) The tops of the A(3,4), B(-2,5) అయిన మూడవ శీర్షం C
View SolutionLet T_n denote the number of triangles, which can be formed using the vertices of a regular polygon of n sides. It T_(n+1)-T_n=21 ,then n equals a. 5 b. 7 c. 6 d. 4
View SolutionThe value of cot∑19n=1(cot−1(1+n∑p=12p)) is equal to (a) 2119 (b) 1921 (c) −1921 (d) −2119
View SolutionThe median of 21, 17, 13, 33, 19, 23 is......
View Solutionनिम्नलिखित में न्यूनतम बारम्बारता वाले चर का मान लिखें।
17,19,21,17,23,25,23,25,23,23,25,21,25
View SolutionWrite the difference between the frequency of 23 and the frequency of 19 in the following data 19, 21, 23, 35, 37, 15, 27, 39, 23, 27, 35, 19, 23, 19, 38, 40, 42.
View Solutionनिम्नलिखित आँकड़ों में 23 की बारंबारता और 19 की बारंबारता का अंतरफल लिखिए
19, 21, 23, 35, 37, 15, 27, 39, 23, 27, 35, 19, 23, 19, 38, 40, 42.
View SolutionLet Tn denote of the number of triangles which can be formed using the vertices of a regular polygon of n sides If Tn+1−Tn=21 then n is
View Solution
