x, x+2, x+6లు గుణశ్రేఢిలో మూడు వరుస పదాలైన 'x' విలువను కనుగొనుము.
3
4
2
1
Step by step video & image solution for x, x+2, x+6లు గుణశ్రేఢిలో మూడు వరుస పదాలైన 'x' విలువను కనుగొనుము. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ఒక టాక్సీ మొదటి కి.మీ. ప్రయాణానికి Rs.20 ల చొప్పున, తరువాత ప్రతి కి,మీ.కు Rs.8 ల చొప్పున చెల్లించవలసిన, 15 కి.మీ. ప్రయాణానికి అయ్యే సొమ్ము Rs.....
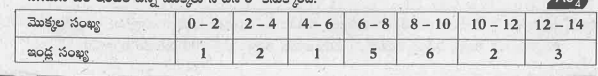
View Solutionఒక' గ్రామంలో కొంతమంది విద్యార్థుల జట్టు “పర్యావరణ పరిరక్షణ - అవగాహని అనే కార్యక్రమంలో భాగంగా, 20 ఇండ్లలో సర్వే నిర్వహించి, ఎన్నెన్ని మొక్కలు నాటినారో సమాచారాన్ని సేకరించి, ఈ క్రింది పట్టికలో నమోదు చేసినారు. సగటున ఒక ఇంటికి ఎన్ని మొక్కలు నాటినారో కనుక్కోండి.
View SolutionSolve the following: |x−2|=(x−2) |x+2|=−x−3 ∣∣x2−x∣∣=x2−x ∣∣x2−x−2∣∣=2+x−x2
View Solutionf(x)={(a|x^(2)-x-2|)/(2+x-x^(2)),x<2 and b,x=2 and (x-[x])/(x-2),x is continuous at x=2 and [] is GLF.then valueof a and b are
View SolutionSolve the following (i) | x-2 | = (x-2) (ii) |x+3 |=-x-3 (iii) ∣∣x2−x∣∣=x2−x (iv) ∣∣x2−x−2∣∣=2+x−x2
View SolutionSolve the following :
(i) |x−2|=(x−2) (ii) |x+3|=−x−3
(iii) ∣∣x2−x∣∣=x2−x (iv) ∣∣x2−x−2∣∣=2+x−x2
View Solution∣∣ ∣∣xaaaxaaax∣∣ ∣∣=(x+2)(x−a)2
View Solutionनीचे लिखे समाधान में दोष निकालें ।
x2−x2=x2−x2
या x(x−x)=(x+x)(x−x)
∴x=x+x=2x,
∴1=2
View SolutionIf f(x) is continuous at x=2, where f(x)=⎧⎨⎩x2−(a+2)x+ax−2, for x≠22, for x=2, then a=
View SolutionIf f(x)=⎧⎨⎩(x−2)2−(1|x−2|+1x−2)x≠20x=2 then f(x) at x=2 is
View Solutionకింది బాహుపదులలో P(0),p(1)మరియుp(2)విలువలుకనుగొనుము.p(x)=x^(2)-x+1
View Solutionకింది బాహుపదులలో P(0),p(1)మరియుp(2)విలువలుకనుగొనుము.p(x)=x^(2)-3x+2
View SolutionQ(6,-8) ల x నిరూపకం, y నిరూపకాలను రాసి ప్రతి బిందువు యొక్క స్థానాన్ని కనుగొనుము.
View Solutionఒక త్రిభుజము XYZ లో, ∠Y లంబకోణము మరియు XZ=17 సెంమీ, YZ=15 సెంమీ, tan X లను కనుగొనుము.
View SolutionIf f(x)=∣∣ ∣ ∣∣x−2(x−1)2x3(x−1)x2(x+1)3x(x+1)2(x+2)3∣∣ ∣ ∣∣ then coefficient of x in f(x) is
View SolutionSolve the following :
(i) |x−2|=(x−2) (ii) |x+3|=−x−3
(iii) ∣∣x2−x∣∣=x2−x (iv) ∣∣x2−x−2∣∣=2+x−x2
View Solution
