ఒక అంకశ్రేఢి a2=6 మరియు a7=−4 అయిన an=0 అయ్యేట్లు n విలువ .....
4
5
6
8
Step by step video & image solution for ఒక అంకశ్రేఢి a_2 = 6 మరియు a_7 = - 4 అయిన a_n= 0 అయ్యేట్లు n విలువ ..... by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ఒక టాక్సీ మొదటి కి.మీ. ప్రయాణానికి Rs.20 ల చొప్పున, తరువాత ప్రతి కి,మీ.కు Rs.8 ల చొప్పున చెల్లించవలసిన, 15 కి.మీ. ప్రయాణానికి అయ్యే సొమ్ము Rs.....
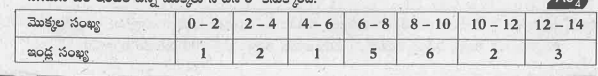
View Solutionఒక' గ్రామంలో కొంతమంది విద్యార్థుల జట్టు “పర్యావరణ పరిరక్షణ - అవగాహని అనే కార్యక్రమంలో భాగంగా, 20 ఇండ్లలో సర్వే నిర్వహించి, ఎన్నెన్ని మొక్కలు నాటినారో సమాచారాన్ని సేకరించి, ఈ క్రింది పట్టికలో నమోదు చేసినారు. సగటున ఒక ఇంటికి ఎన్ని మొక్కలు నాటినారో కనుక్కోండి.
View SolutionLet A_1, A_2........A_7 be a polygon and a_1........a_7 be the complex numbers representing vertices A_1,A_2........A_7. if, |a_1|=|a_2|=.............|a_7|=R, then sum_(1 le j le 7) |a_1+a_2|^2
View SolutionIf a1,a2,….an are in H.P then a1a2+,a3,…,an,a2a1+a3+….+an,…,ana1+a2+….+an−1
are in
View SolutionA sequence an,n∈N be an A.P. such that a7 = 9 and a1a2a7 is least, then the common difference is:
View Solutionp(x)=x^(2)-5x+6, q(x)=x-2 మరియు r(x)=0 అయిన g(x)=
View SolutionPrice of 5 pens and 3 pencils p Price of Rs., 3 pens and 2 pencils q' రూ॥1లు అయిన 2 పెన్నులు మరియు ఒక పెన్సిల్ ధర = ............. రూ॥
View SolutionS = {x,x అనేది 2n + 3, nవ పదంగా గల అంకథ్రేఢిలోని పదము, మరియు n<6) అయిన S=
View Solutionयदि a1,a2,a3…..an सा श्री में है तथा a1=0तब (a3a2+a4a3+….+anan−1)−a2(1a2+1a3+…..+1an−2) का मान निम्न में से किसके बराबर है
View SolutionIf a1,a2,......,an>0, then prove that a1a2+a2a3+a3a4+.....+an−1an+ana1>n
View SolutionIf ai>0,i=1,2,3,...n and (n−1)s=a1+a2+a3+....+an, prove that a1⋅a2⋅a3⋅....an≥(n−1)n⋅(s−a1)(s−a2)...(s−an).
View SolutionIf ai>0,i=1,2,3,...,n then prove that a1a2+a2a3+a3a4+...+an−1an+ana1≥n.
View SolutionIf a1,a2,a3,...,an... are in A.P. such that a4−a7+a10=m, then sum of the first 13 terms of the A.P.is
View SolutionIf a1.a2.......an are positive and (n - 1) s = a1+a2+.....+an then prove that (a1+a2+....+an)n≥(n2−n)n(s−a1)(s−a2)........(s−an)
View SolutionA sequence an,n∈N be an A.P. such that a7 = 9 and a1a2a7 is least, then the common difference is:
View Solution
