The maximum distance of a point on the graph of the function $y = \sqrt{3} sin x + cos x$ from X-axis is

Video Solution

|

Answer

Step by step video & image solution for The maximum distance of a point on the graph of the function y = sqrt 3 sin x + cos x from X-axis is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 MATHS QUESTION BANK

Similar Questions

The maximum distance of a point on the graph of function $y = \sqrt{3} sin x + cos x$ from x -axis is $\dots\dots\dots\dots.$
View Solution
The maximum distance of a point on the graph of function $y = \sqrt{3} sin x + cos x$ from x -axis is $\dots\dots\dots\dots.$
View Solution

Knowledge Check

Question 1 - Select One
The function $y = f (x)$ is graphed in the standard (x, y) coordinate plane below.

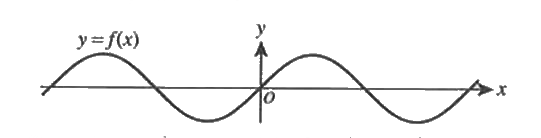
The points on the graph of the function $y = 3 + f (x - 1)$ can be obtained from the points on $y = f (x)$ by a shift of:
A1 unit to the right and 3 unit up.
B1 unit to the right and 3 unit down.
C3 unit to the right and 1 unit up.
D3 unit to the right and 1 unit down.
Question 2 - Select One
$\sqrt{3} sin x + cos x$ is maximum when x is
A $30^{\circ}$
B $90^{\circ}$
C $60^{\circ}$
D $45^{\circ}$

Recommended Questions

Home
Ask Doubt
Profile