AB is focal chord of a parabola. Let D and C be foot of perpendicular from A & B on it's directrix respectively. If CD= 6 and area of trapezium ABCD is 24 square units, then find length of chord AB.
Step by step video & image solution for AB is focal chord of a parabola. Let D and C be foot of perpendicular from A & B on it's directrix respectively. If CD= 6 and area of trapezium ABCD is 24 square units, then find length of chord AB. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Let AB is the focal chord of a parabola and D and C be the foot of the perpendiculars from A and B on its directrix respectively. If CD = 6 units and area of trapezium ABCD is 36 square units, then the length (in units) of the chord AB is
View SolutionAB is a focal chord of the parabola y2=4ax. If A=(4a,4a) then B=
View Solution
Knowledge Check
AB is a chord of the parabola y2=4ax with vertex A ,BC is draw perpendicular to AB meeting the axis at C the projection of BC on the axis of the parabola is
AaB2aC4aD8aIn AB and CD are two equal chords of a circle with center O. OP and OQ are perpendiculars on chords AB and CD respectively. If ∠POQ=150∘ then ∠APQ is equal to
A30∘B75∘C15∘D60∘ABCD is a trapezium with AB || CD. If AB = 10 cm, CD = 7 cm and area of trapezium = 102 cm2, then find the height of trapezium (in cm).
AA)10BB)12CC)14DD)16
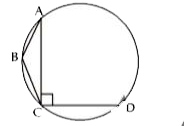
In the following figure, AB = BC, AC = CD and ∠ACD=90∘. If the radius of the circle is 'r' units, then find the length of the chord BC.
View SolutionDivide Rs.6500 among A,B and C so that after spending 90% , 75% and 60% of their respective saving were in the ratio of 3: 5: 6
View SolutionIn a circle of radius 5 cm, AB and AC are two chords such that AB=AC=6cm. Find the length of the chord BC
View SolutionAB and CD are chords of a circle with centre O. AB = 48 cm and its distance from centre O is 10 cm. If the distance of CD from centre O is 24 cm, find the length of CD.
View SolutionIn a trapezium ABCD, AB is a parallel to CD. BD is perpendicular to AD. AC is perpendicular to BC. If AD=BC=15cmandAB=25cm, then the area of the trapezium is :
View Solution
