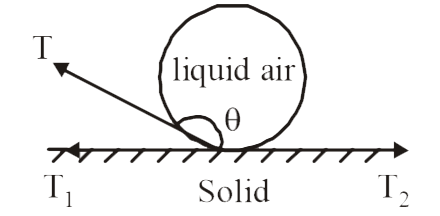
Let $T_{1}$ be surface tension between solid and air, $T_{2}$ be the surface tension between solid and liquid and T be the surface tension between liquid and air. Then in equilibrium, for a drop of liquid on a clean glass plate, the relation is ( $θ$ is angle of contact)

A

$cos θ = \frac{T}{T_{1} + T_{2}}$

B

$cos θ = \frac{T}{T_{1} - T_{2}}$

C

$cos θ = \frac{T_{1} T_{2}}{T}$

D

$cos θ = \frac{T_{1} - T_{2}}{T}$

Text Solution

Verified by Experts

The correct Answer is:A

$T_{1} + T cos (π - θ) = T_{2}$
$∴ cos (π - θ) = \frac{T_{2} - T_{1}}{T}$
$∴ cos θ = \frac{T_{1} - T_{2}}{T}$

|

Updated on:21/07/2023

Class 12 PHYSICS MECHANICAL PROPERTIES OF FLUIDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If the surface tension of a liquid is T , the gain in surface energy for an increase in liquid surface by A is
A $A T^{- 1}$
BAT
C $A^{2} T$
D $A^{2} T^{- 2}$
Question 2 - Select One
Internal pressure inside a liquid drop of radius r and surface tension T is
A $\frac{2 T}{r} - P_{0}$
B $\frac{4 T}{r} + P_{0}$
C $P_{0} + \frac{2 T}{r}$
D $\frac{T}{4 r} - P_{0}$
Question 3 - Select One
If T is the surface tension of a liquid, the energy needed to break a liquid drop of radius R into 64 drops is
A $6 π R^{2} T$
B $π R^{2} T$
C $12 π R^{2} T$
D $8 π R^{2} T$

DISHA PUBLICATION-MECHANICAL PROPERTIES OF FLUIDS -EXERCISE-1 CONCEPT BUILDER

Home
Ask Doubt
Profile