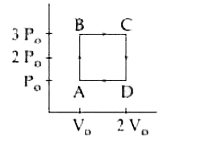
An engine operates by taking n moles of an ideal gas through the cycle ABCDA shown in figure. The thermal efficiency of the engine is: (Take Cv =1.5 R, where R is gas constant)

0.24
0.15
0.32
0.08
The correct Answer is:B
Work-done (W) =P6V6
According to principle of calorimetry
Heat given =QAB=QBC
=32(nRTB−nRTA)+52(nRTC−nRTB)
=32(2P0V0−P0V0)+52(4P0V0−2P0V0)=132P0V0
Thermal efficiency of engine (η)
=WQgiven=213=0.15
Topper's Solved these Questions
- THERMODYNAMICS BOOK - DISHA PUBLICATIONCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE -2 : CONCEPT APPLICATOR
29 Videos
- THERMODYNAMICS BOOK - DISHA PUBLICATIONCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE-1 : CONCEPT BUILDER (Topic-2 SPECIFIC HEAT CAPACITY THERMODYNAMICS PROCESSES)
33 Videos
- THERMAL PROPERTIES OF MATTER BOOK - DISHA PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTER EXERCISE - Exercise-2 (Concept Applicator)
28 Videos
- WAVE OPTICSBOOK - DISHA PUBLICATIONCHAPTER - WAVE OPTICSEXERCISE - EXERCISE-2 : CONCEPT APPLICATOR
30 Videos
Similar Questions
An engine operates by taking n moles of an ideal gas through the cycle ABCDA shown in figure. The thermal efficiency of the engine is -(Take Cv = 15.R, where R is gas constant)
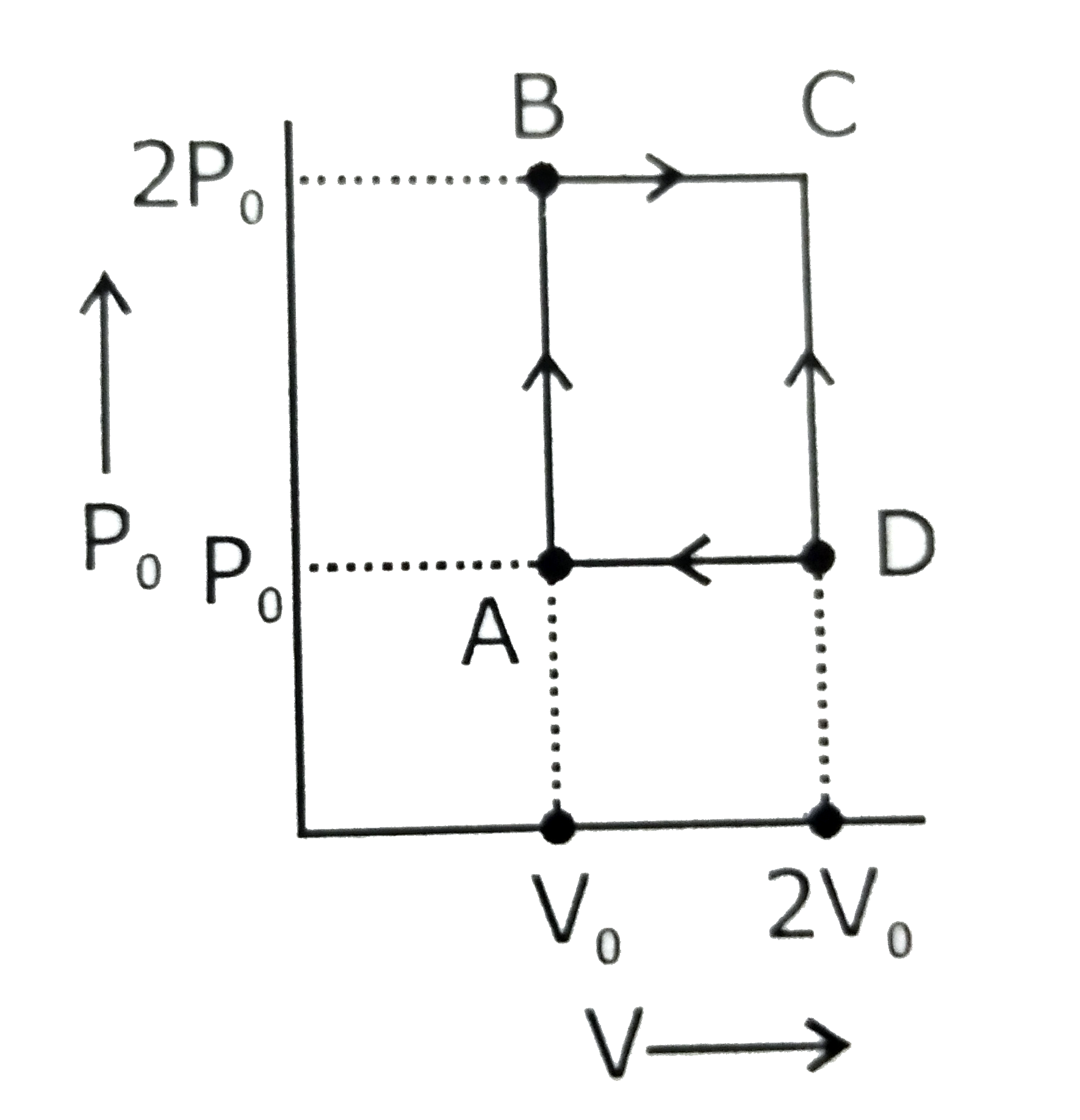
View SolutionAn engine operates by taking n moles of an ideal gas through the cycle ABCDA shown in figure. The thermal efficiency of the engine is -(Take Cv = 15.R, where R is gas constant)

View Solution
Knowledge Check
An ideal monoatomic gas is carried around the cycle ABCDA as shown in the figure. The efficiency of the gas cycle is
 A421B221C431D231
A421B221C431D231An ideal monoatomic gas is carried around the cycle ABCDA as shown in the figure. The efficiency of the cycle is ltBrgt
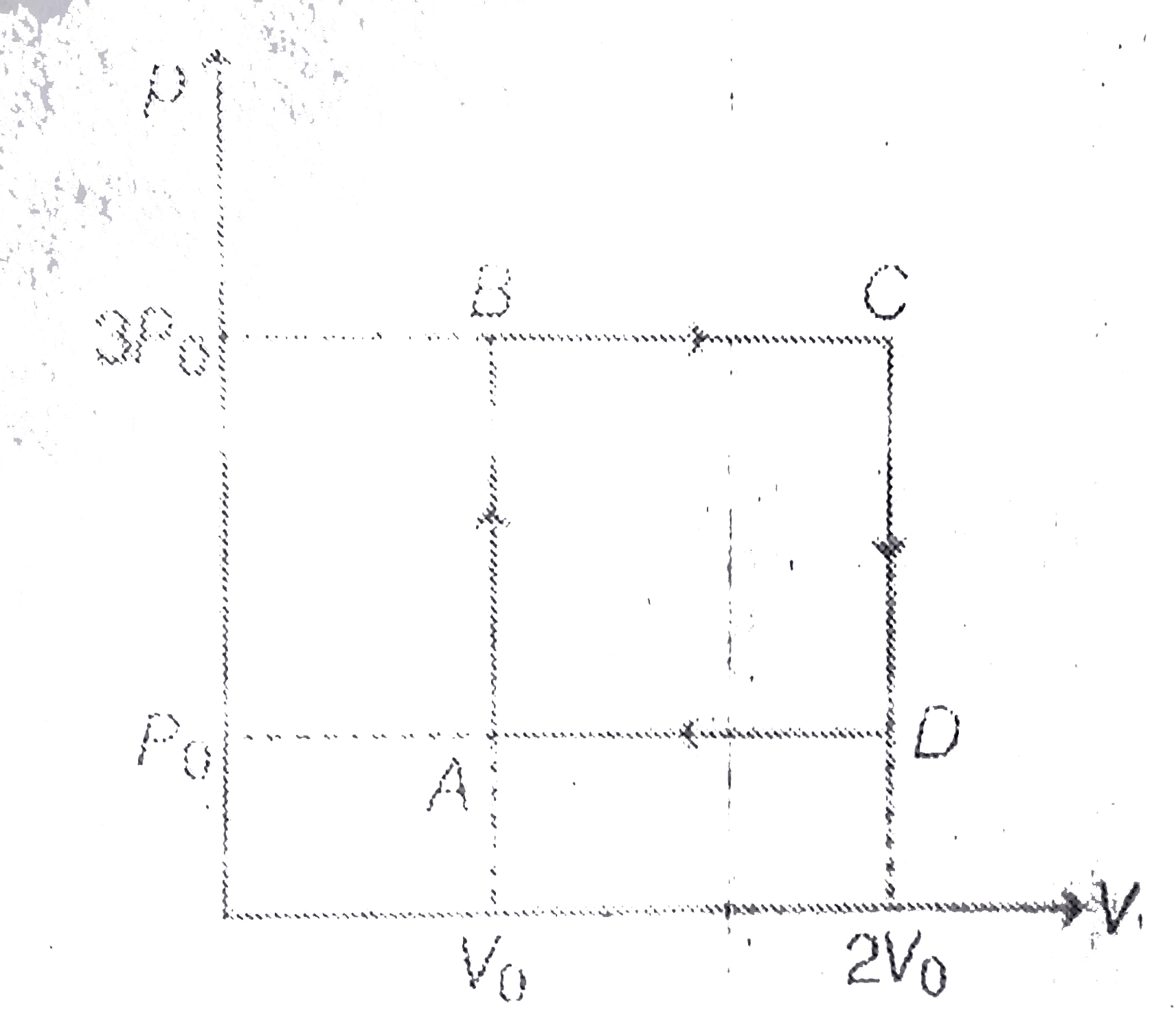 A28.6%B34.2%C46.8%D62.3%
A28.6%B34.2%C46.8%D62.3%One mole of an ideal monoatomic gas undergoes a process as shown in the figure. Find the molar specific heat of the gas in the process.
R is a gas constant.A3R4BR2CR3D2R3
An engine operates by taking n moles of an ideal gas through the cycle ABCDA shown in figure. The thermal efficiency of the engine is -(Take Cv = 15.R, where R is gas constant)

View SolutionAn engine operates by taking a monatomic ideal gas through the cycle shown in the figure. The percentage efficiency of the engine is close to ____________
View SolutionAn engine operates by taking a monoatomic ideal gas through the cycle shown in the figure. The percentage efficiency of the engine is close to ____________

View SolutionAn ideal monoatomic gas is carried around the cycle ABCDA as shown in the figure. The efficiency of the gas cycle is

View SolutionAn ideal monoatomic gas is carried around the cycle ABCDA as shown in the figure. The efficiency of the cycle is ltBrgt

View Solution
