Four charge q1=2×10−8C,q2=−2×10−8C,q3=−3×10−8C, and q4=6×10−8C are palced at four corners of a square of side √2m. What is the potential at the centre of the square?
270V
300V
Zero
100V
The correct Answer is:A
To find the electric potential at the center of a square with four charges placed at its corners, we can follow these steps:
Step 1: Identify the Charges and Their Positions
We have four charges:
- q1=2×10−8C
- q2=−2×10−8C
- q3=−3×10−8C
- q4=6×10−8C
These charges are placed at the corners of a square with a side length of √2m.
Step 2: Calculate the Distance from Each Charge to the Center
The center of the square is equidistant from all four corners. The distance R from each corner to the center can be calculated using the formula for the diagonal of a square:
R=d2=√22+222=√42=1m
where d=√22+22=2.
Step 3: Calculate the Total Charge
The total charge Qtotal is the algebraic sum of all the charges:
Qtotal=q1+q2+q3+q4
Substituting the values:
Qtotal=(2×10−8)+(−2×10−8)+(−3×10−8)+(6×10−8)
Calculating this gives:
Qtotal=2−2−3+6=3×10−8C
Step 4: Calculate the Electric Potential at the Center
The electric potential V at a point due to a point charge is given by:
V=kQR
where k=9×109N⋅m2/C2 is Coulomb's constant.
Since all charges are at the same distance R=1m, the total potential at the center can be calculated as:
V=kQtotalR
Substituting the values:
V=9×1093×10−81
Calculating this gives:
V=9×3×101=27×101=270V
Final Answer
The potential at the center of the square is 270V.
---
We have four charges:
- q1=2×10−8C
- q2=−2×10−8C
- q3=−3×10−8C
- q4=6×10−8C
The center of the square is equidistant from all four corners. The distance R from each corner to the center can be calculated using the formula for the diagonal of a square:
R=d2=√22+222=√42=1m
where d=√22+22=2.
The total charge Qtotal is the algebraic sum of all the charges:
Qtotal=q1+q2+q3+q4
Substituting the values:
Qtotal=(2×10−8)+(−2×10−8)+(−3×10−8)+(6×10−8)
Calculating this gives:
Qtotal=2−2−3+6=3×10−8C
The electric potential V at a point due to a point charge is given by:
V=kQR
where k=9×109N⋅m2/C2 is Coulomb's constant.
V=kQtotalR
Substituting the values:
V=9×1093×10−81
Calculating this gives:
V=9×3×101=27×101=270V
The potential at the center of the square is 270V.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - DISHA PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE 2: CONCEPT APPLICATOR
24 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCEBOOK - DISHA PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - EXERCISE 2: CONCEPT APPLICATOR
24 Videos
- ELECTROMAGNETIC WAVESBOOK - DISHA PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise - 2 : Concept Applicator
30 Videos
- GRAVITATIONBOOK - DISHA PUBLICATIONCHAPTER - GRAVITATIONEXERCISE - EXERCISE-2
30 Videos
Similar Questions
Four charges +q,+2q,+3q and +4q are placed at the four corners ABCD of a square of side a. Calculate the field and potential at the centre of the square.
View SolutionFour charges 10−5,−2×10−5,+3×10−5 and 2×10−5 coulomb are placed at the four corners of a square of side 1m the potential at the centre of the square is...
View Solution
Knowledge Check
Four charges q1=2×10−8C,q2=−2×10−8C,q3=−3×10−8C and q4=6×10−5C are placed at four corners of a square of side √2m. What is the potential at the centre of the square ?
A270 VB300 VCZeroD100 VFour charges q1=2×10−8C,q2=−2×10−8C,q3=−3×10−8C and q4=6×10−5C are placed at four corners of a square of side √2m. What is the potential at the centre of the square ?
A270 VB300 VCZeroD100 VFour charges 10−8,−2×108,+3×10−8and2×10−8 coulomb are placed at the four corners of a square of side 1m the potential at the centre of the square is
AzeroB360 voltC180 voltD360√2 volt
[" Four charges "10^(-3):-2times10^(-5);+3times10^(-5)" and "],[2times10^(-5)" coulomb are placed at the four "],[" corners of a square of side "1m" the potential "],[" at the centre of the square is "]
View SolutionFour point charges q1,q2,q3 and q4 are placed at the corners of the squares of side a,as shown in figure.Calculate the potential at the centre of the square.
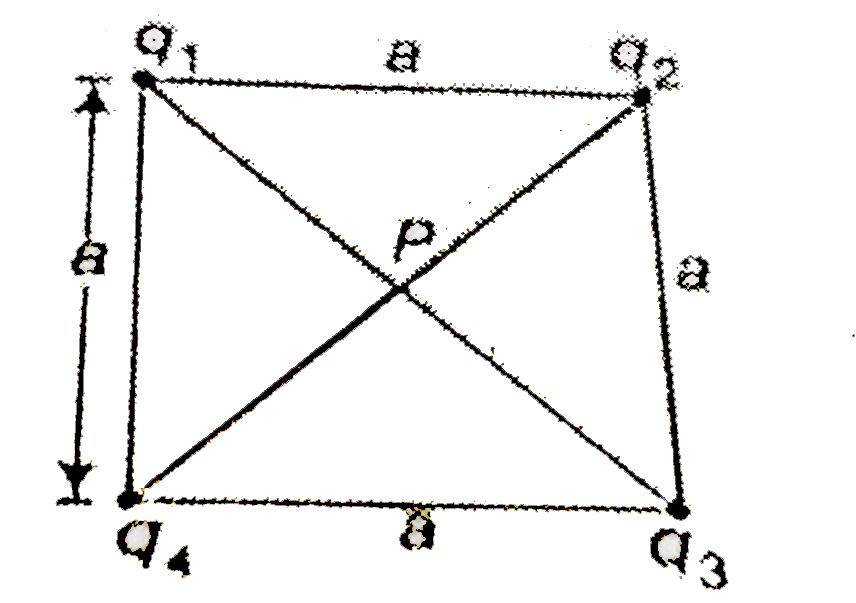
(Given, q1=1×10−8C,q2=−2×10−8C,q3=3×10−8C,q4=2×10−8C and a=1m)
View SolutionElectric charges of +10μC,+5μC,−3μCand+8μC are placed at the corners of a square of side √2 m, the potential at the centre of the square is
View SolutionCharges of +103×10−9C are placed at each of the four corners of a square of side 8cm. The potential at the intersection of the diagonals is
View SolutionElectric charges of +10μC,+5μC,−3μCand+8μC are placed at the corners of a square of side √2m. The potential at the centre of the square is
View Solution
