If particle is excuting simple harmonic motion with time period T, then the time period of its total mechanical energy is :-
Zero
T/2
2T
Infinite
The correct Answer is:D
To solve the problem, we need to analyze the total mechanical energy of a particle executing simple harmonic motion (SHM) and determine its time period.
1. Understanding Simple Harmonic Motion (SHM):
- In SHM, a particle oscillates back and forth around an equilibrium position. The total mechanical energy (E) of a particle in SHM is given by the sum of its kinetic energy (K.E) and potential energy (P.E).
2. Expression for Total Mechanical Energy:
- The total mechanical energy in SHM can be expressed as:
E=12kA2
where k is the spring constant and A is the amplitude of the motion.
3. Relating Angular Frequency and Time Period:
- The angular frequency ω is related to the time period T by the formula:
ω=2πT
4. Substituting Angular Frequency:
- The total mechanical energy can also be expressed in terms of angular frequency:
E=12mA2ω2
- Substituting ω=2πT:
E=12mA2(2πT)2
E=12mA2⋅4π2T2
E=2mA2π2T2
5. Observing the Nature of Total Mechanical Energy:
- The expression for total mechanical energy E is a constant value because it does not depend on time. It remains constant as the particle oscillates.
6. Determining the Time Period of Total Mechanical Energy:
- Since the total mechanical energy does not change with time, the time period of the total mechanical energy is effectively infinite. This means that the energy does not oscillate or vary with time.
Conclusion:
The time period of the total mechanical energy of a particle executing simple harmonic motion is infinite.
Final Answer:
The time period of the total mechanical energy is infinite (Option D).
- In SHM, a particle oscillates back and forth around an equilibrium position. The total mechanical energy (E) of a particle in SHM is given by the sum of its kinetic energy (K.E) and potential energy (P.E).
- The total mechanical energy in SHM can be expressed as:
E=12kA2
where k is the spring constant and A is the amplitude of the motion.
- The angular frequency ω is related to the time period T by the formula:
ω=2πT
- The total mechanical energy can also be expressed in terms of angular frequency:
E=12mA2ω2
- Substituting ω=2πT:
E=12mA2(2πT)2
E=12mA2⋅4π2T2
E=2mA2π2T2
- The expression for total mechanical energy E is a constant value because it does not depend on time. It remains constant as the particle oscillates.
- Since the total mechanical energy does not change with time, the time period of the total mechanical energy is effectively infinite. This means that the energy does not oscillate or vary with time.
The time period of the total mechanical energy of a particle executing simple harmonic motion is infinite.
The time period of the total mechanical energy is infinite (Option D).
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Oscillations) (Simple pendulum and types of SHM)17videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Fundamental)23videos
RACE
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Dscillations) (Kinematics of SHM)20videos
RACE
- BOOK - ALLENCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - EXERCISE-III28videos
NEWTONS LAWS OF MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example1videos
SIMPLE HARMONIC MOTION
Similar Questions
Knowledge Check
- Question 1 - Select One
A particle executing simple harmonic motion with time period T. the time period with which its kinetic energy oscillates is
ATB2TC4TDT2 - Question 2 - Select One
A particle is executing SHM with time period T. If time period of its total mechanical energy isT' then T'T is
A2B12CZeroDInfinite - Question 3 - Select One
A particle is executing simple harmonic motion with a time period T. At time t=0, it is at its position of equilibium. The kinetice energy -time graph of the particle will look like
A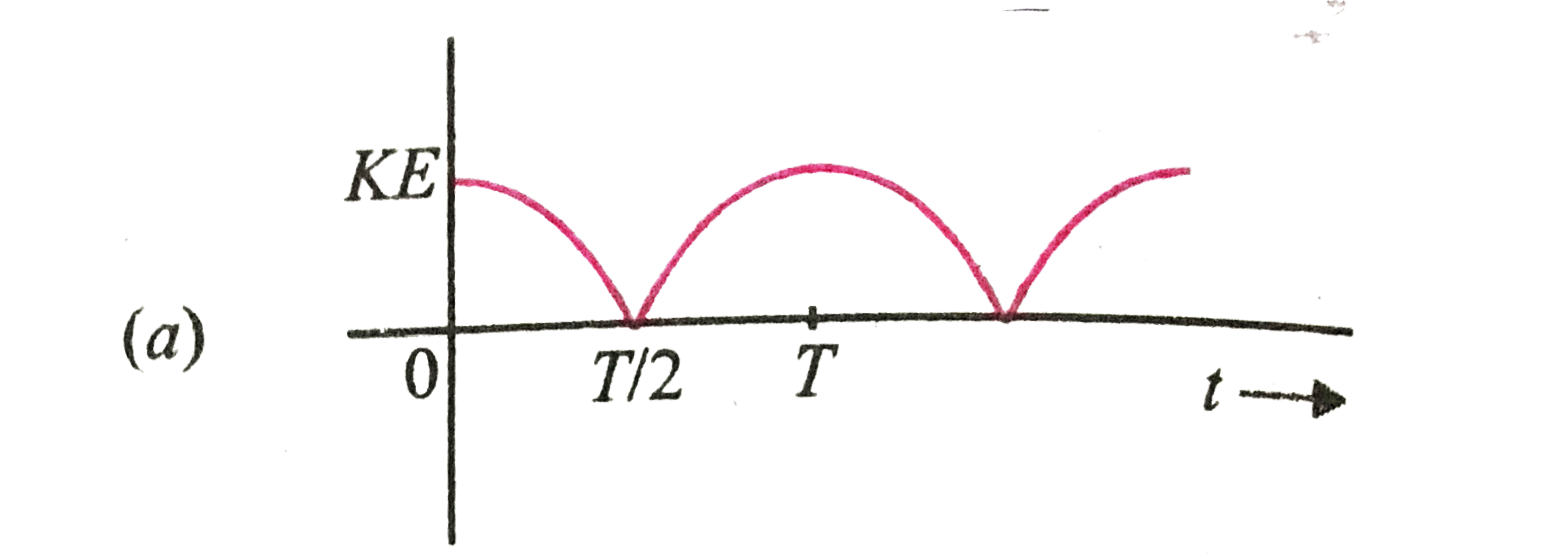 B
B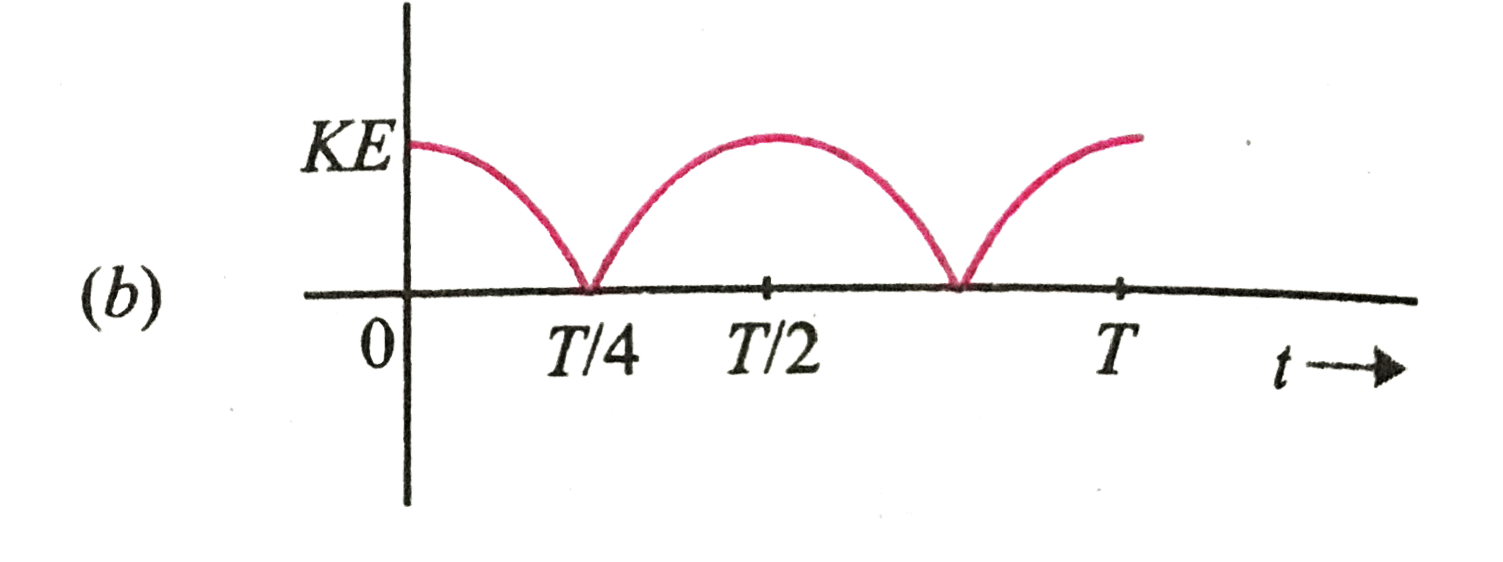 C
C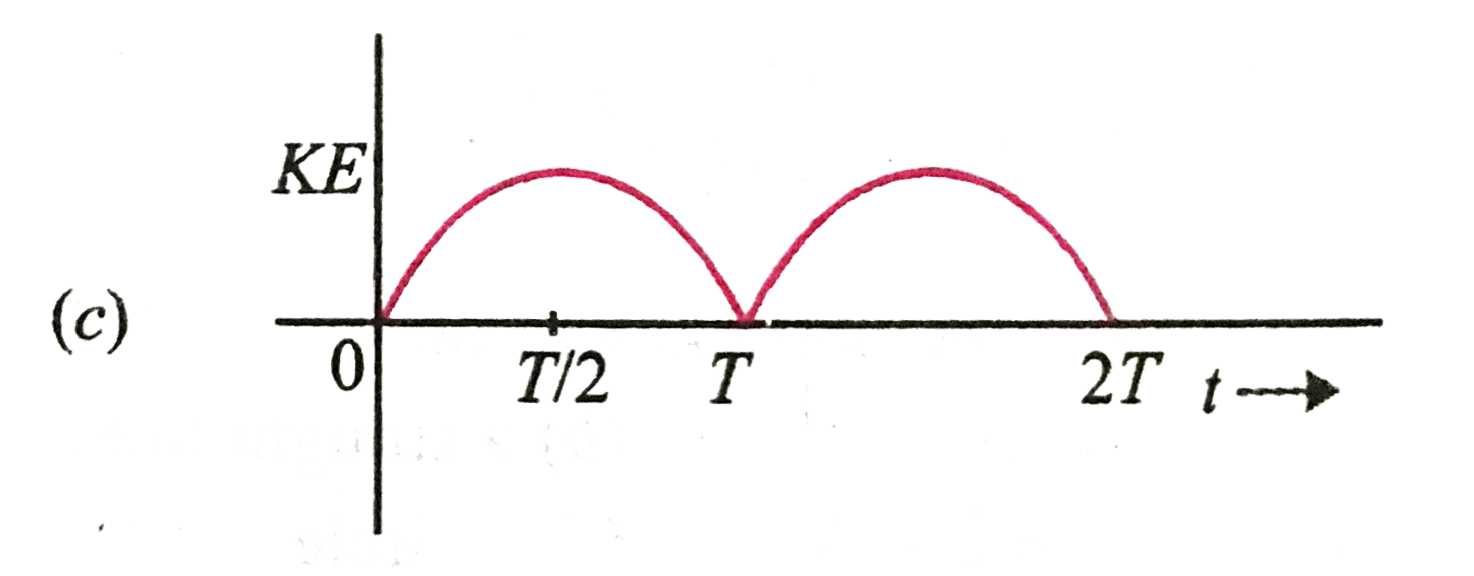 D
D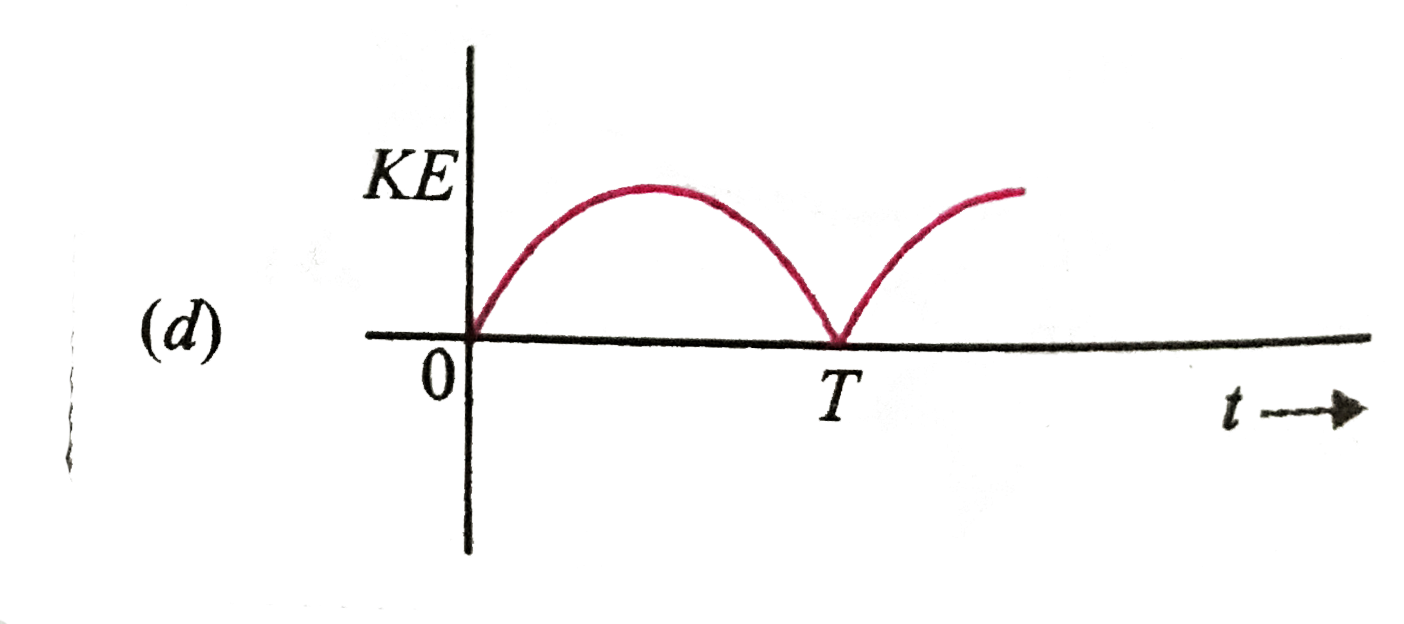
The acceleration displacement graph of a particle executing simple harmonic motion is shown in figure. The time period of simple harmonic motion is
View SolutionIf x, and a denote the displacement, the velocity and the acceler of a particle executing simple harmonic motion of time period T, then, which of the following does not change with time?
View SolutionA particle is executing simple harmonic motion .Its total energy is proportional to its -
View SolutionIf x,v and a denote the displacement, the velocity and the acceleration of a particle executing simple harmonic motion of time period T, then, which of the following does not change with time ?
View SolutionA particle is executing simple harmonic motion with an amplitude A and time period T. The displacement of the particles after 2T period from its initial position is
View Solution


