In the figure , the intensity of waves arriving at D from two coherent soucrces $s_{1} and s_{2} i s I_{0}$ . The wavelength of the wave is $λ = 4 m$ . Resultant intensity at D will be

A

$4 I_{0}$

B

$I_{0}$

C

$2 I_{0}$

D

zero

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

|

Answer

Step by step video & image solution for In the figure , the intensity of waves arriving at D from two coherent soucrces s_(1) and s_(2) is I_(0). The wavelength of the wave is lambda = 4 m . Resultant intensity at D will be by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS RACE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One

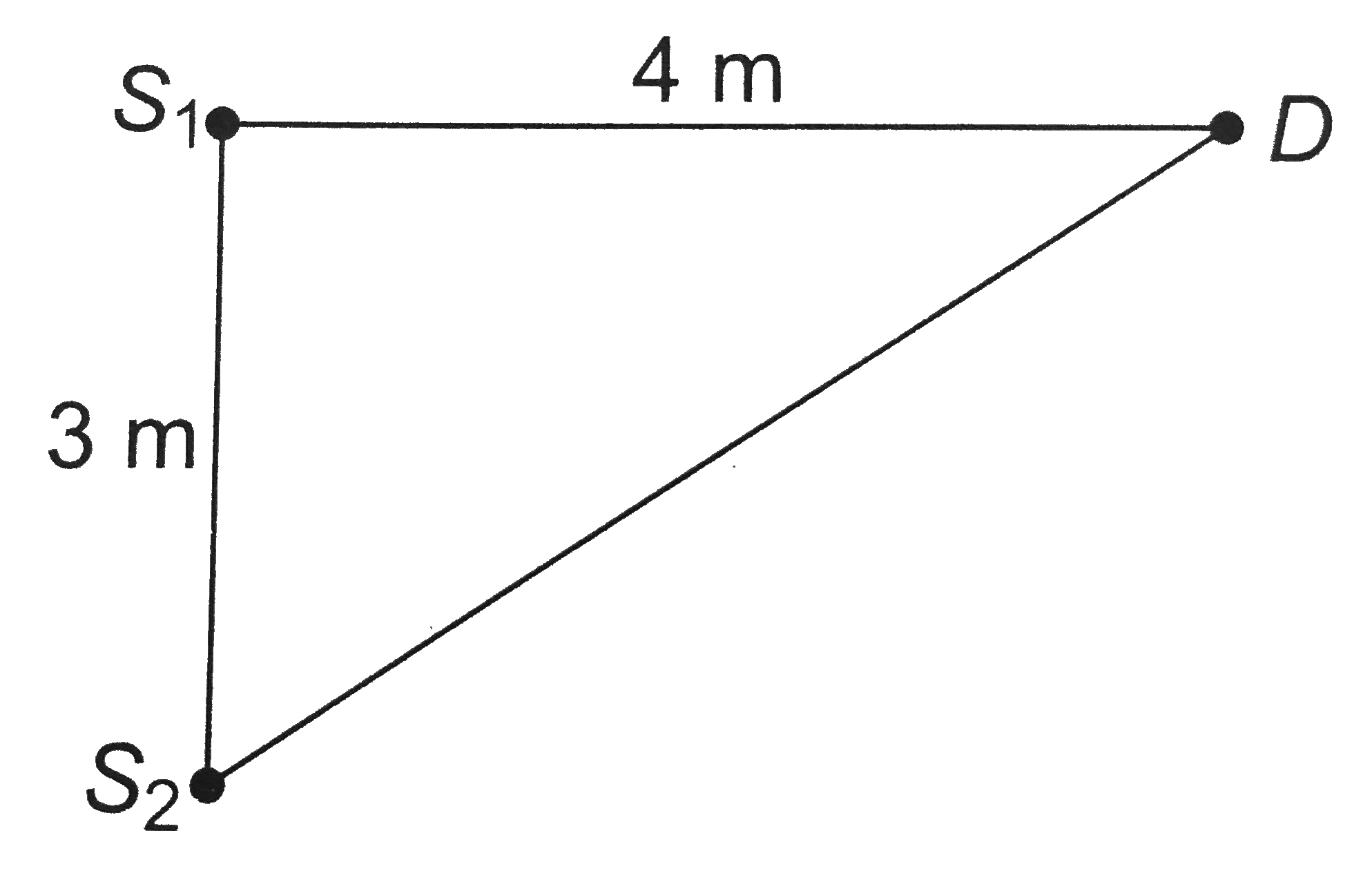
In the figure the intensity of waves arriving at D from two coherent sources $S_{1}$ and $S_{2}$ is $I_{0}$ The wavelength of the wave is $λ = 4 m$ . Resultant intensity at D will be:
A $4 I_{0}$
B $I_{0}$
C $2 I_{0}$
Dzero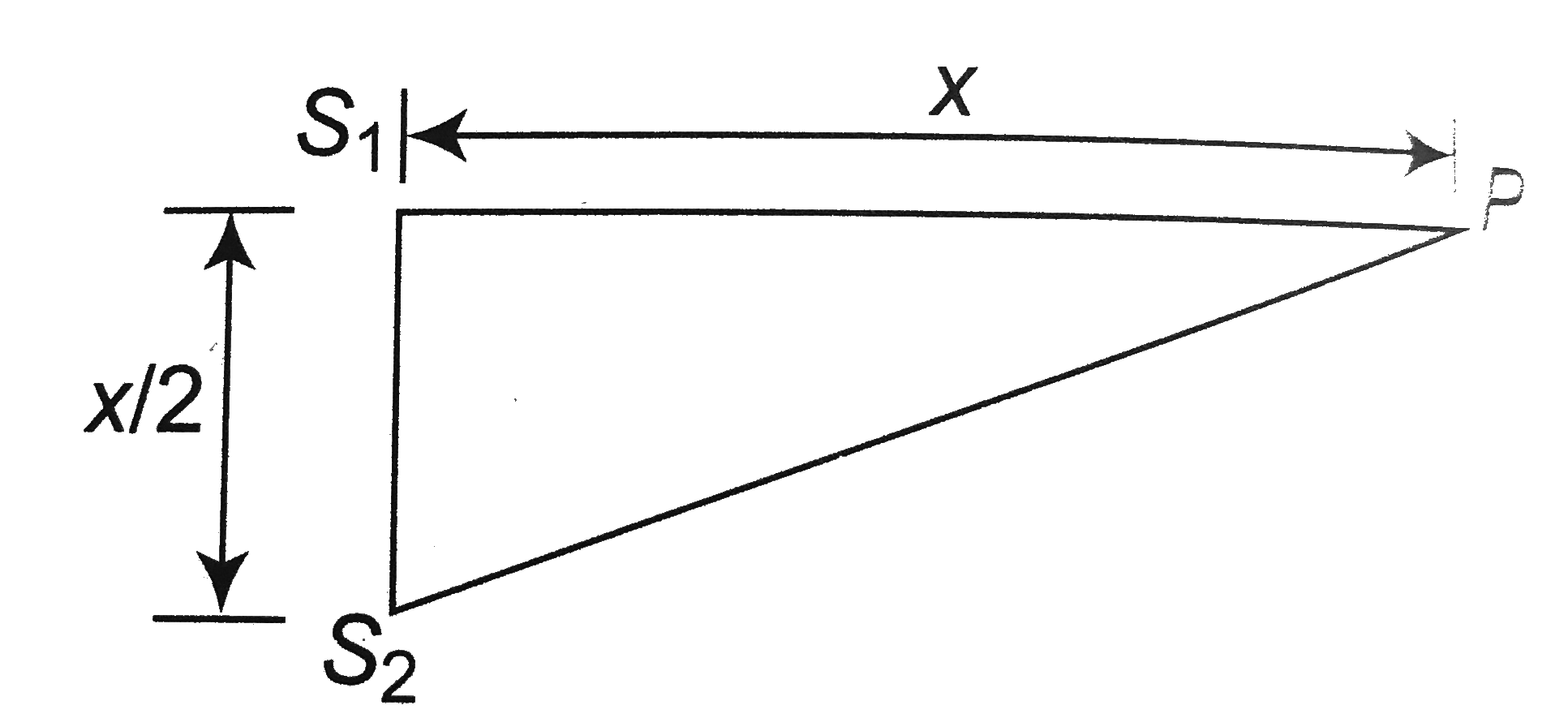
Question 2 - Select One

The wavelength of the waves arriving at P from two coherent sources $S_{1}$ and $S_{2}$ is 4m, while intensity of each wave is $I_{0}$ . The resultant intensity at $P$ is $2 I_{0}$ . Find the minimum value of $S_{2} P$
A $\frac{x \sqrt{5}}{2}$
B $2 x$
C $(x + 1)$
D $(x + \sqrt{2})$
Question 3 - Select One
Two waves of equal amplitude a from two coherent sources $(S_{1} & S_{2})$ interfere at a point R such that $S_{2} R - S_{1} R = 1.5 λ$ The intensity at point R Would be
AZero
B $2 a^{2}$
C $5 a^{2}$
D $6 a^{2}$

ALLEN-RACE-Basic Maths (Wave Motion & Dopplers Effect) (Superposition of waves interfarence, beats)

Home
Ask Doubt
Profile