From a point P on a level ground, the angle of elevation of the top of a tower is 30o . If the tower is 100 m high, the distance of point P from the foot of the tower is (a) 149 m (b) 156 m (c) 173 m (d) 200 m
To solve the problem, we will use trigonometric ratios, specifically the tangent function, which relates the angle of elevation to the height of the tower and the distance from the point to the base of the tower.
1. Identify the Triangle:
We have a right triangle formed by the tower (AB), the ground (AP), and the line of sight from point P to the top of the tower (BP). Here, AB is the height of the tower, AP is the distance from point P to the foot of the tower, and BP is the line of sight.
2. Given Values:
- Height of the tower (AB) = 100 m
- Angle of elevation (∠BAP) = 30°
3. Use the Tangent Function:
The tangent of an angle in a right triangle is defined as the ratio of the opposite side to the adjacent side. Therefore, we can write:
tan(θ)=OppositeAdjacent=ABAP
Substituting the known values:
tan(30°)=100AP
4. Calculate tan(30°:
We know that:
tan(30°)=1√3ortan(30°)=√33
5. Set up the Equation:
Now we can substitute tan(30° into our equation:
1√3=100AP
6. Cross Multiply to Solve for AP:
Cross multiplying gives us:
AP=100⋅√3
7. Calculate the Value of AP:
Using the approximate value of √3≈1.732:
AP≈100⋅1.732=173.2m
8. Final Answer:
Rounding to the nearest whole number, the distance from point P to the foot of the tower is approximately 173 m.
Conclusion:
The distance of point P from the foot of the tower is 173 m, which corresponds to option (c).
We have a right triangle formed by the tower (AB), the ground (AP), and the line of sight from point P to the top of the tower (BP). Here, AB is the height of the tower, AP is the distance from point P to the foot of the tower, and BP is the line of sight.
- Height of the tower (AB) = 100 m
- Angle of elevation (∠BAP) = 30°
The tangent of an angle in a right triangle is defined as the ratio of the opposite side to the adjacent side. Therefore, we can write:
We know that:
Now we can substitute tan(30° into our equation:
Cross multiplying gives us:
Using the approximate value of √3≈1.732:
Rounding to the nearest whole number, the distance from point P to the foot of the tower is approximately 173 m.
The distance of point P from the foot of the tower is 173 m, which corresponds to option (c).
Topper's Solved these Questions
Similar Questions
From a point P on a level ground, the angle of elevation of the top of a tower is 30o . If the tower is 100 m high, the distance of point P from the foot of the tower is (a) 149 m (b) 156 m (c) 173 m (d) 200 m
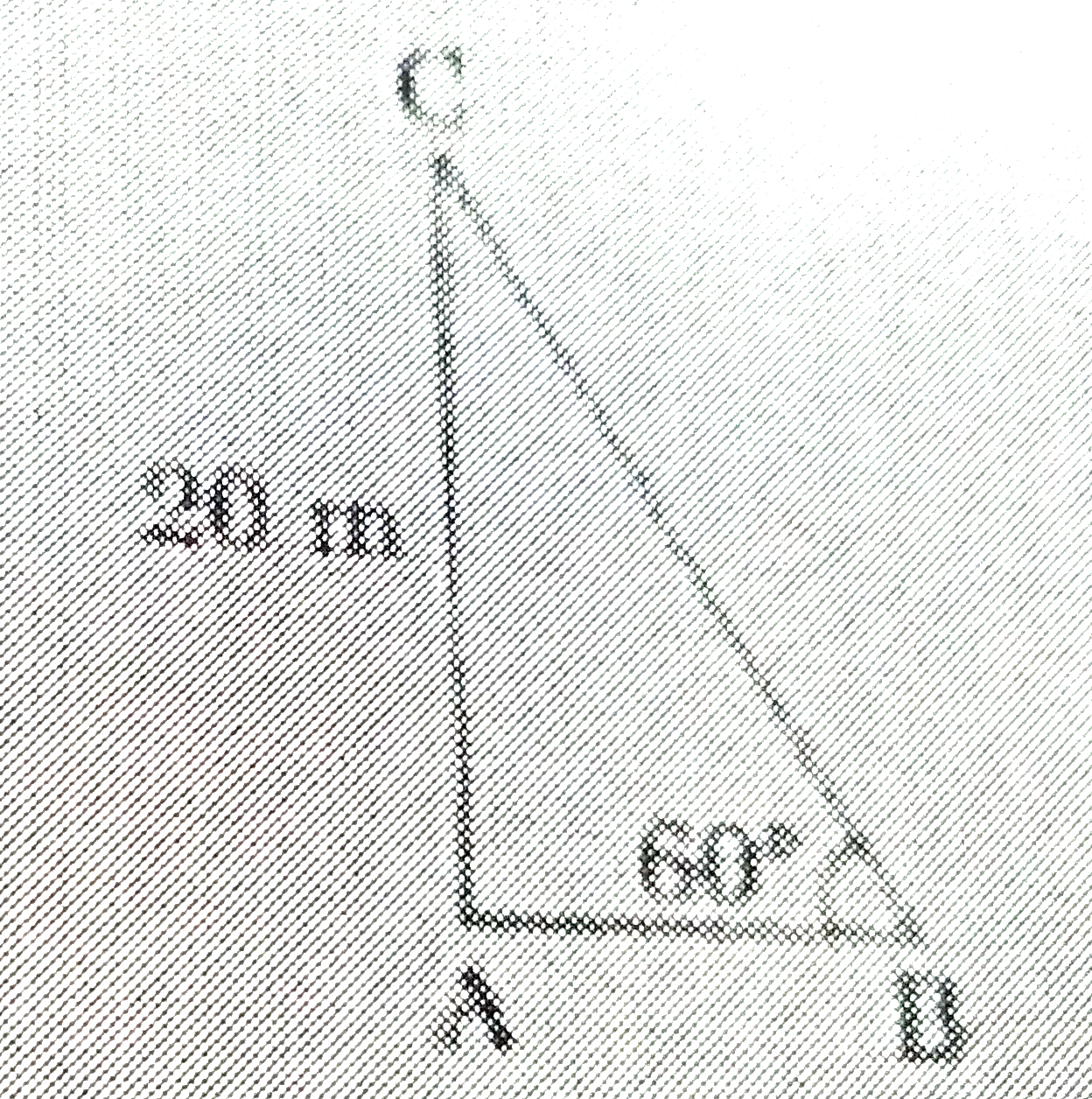
View SolutionIn figure, the angle of elevation of the top of a tower AC from a point B on the ground is 60∘. If the height of the tower is 20m , find the distance of the point from the foot of the tower.
View Solution
Knowledge Check
From a point P on a level ground the angle of elevation to the top of the tower is 30∘ .If the tower is 100 metre high , the distance of point P from the foot of the tower is (Take √3=1.73)
A149 metreB156 metreC173 metreD188 metreThe angle of elevation of the top of a tower of height 100√3m from a point at a distance of 100 m from the foot of the tower on a horizontal plane is
A60∘B90∘C120∘D180∘The angle of elevation of the top of a tower of height 100√3m from a point at a distance of 100 m from the foot of the tower on a horizontal plane is
A60∘B90∘C120∘D180∘
In the figure-2, the angle of elevation of the top of tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m. find the distance of the point from the foot of the tower.
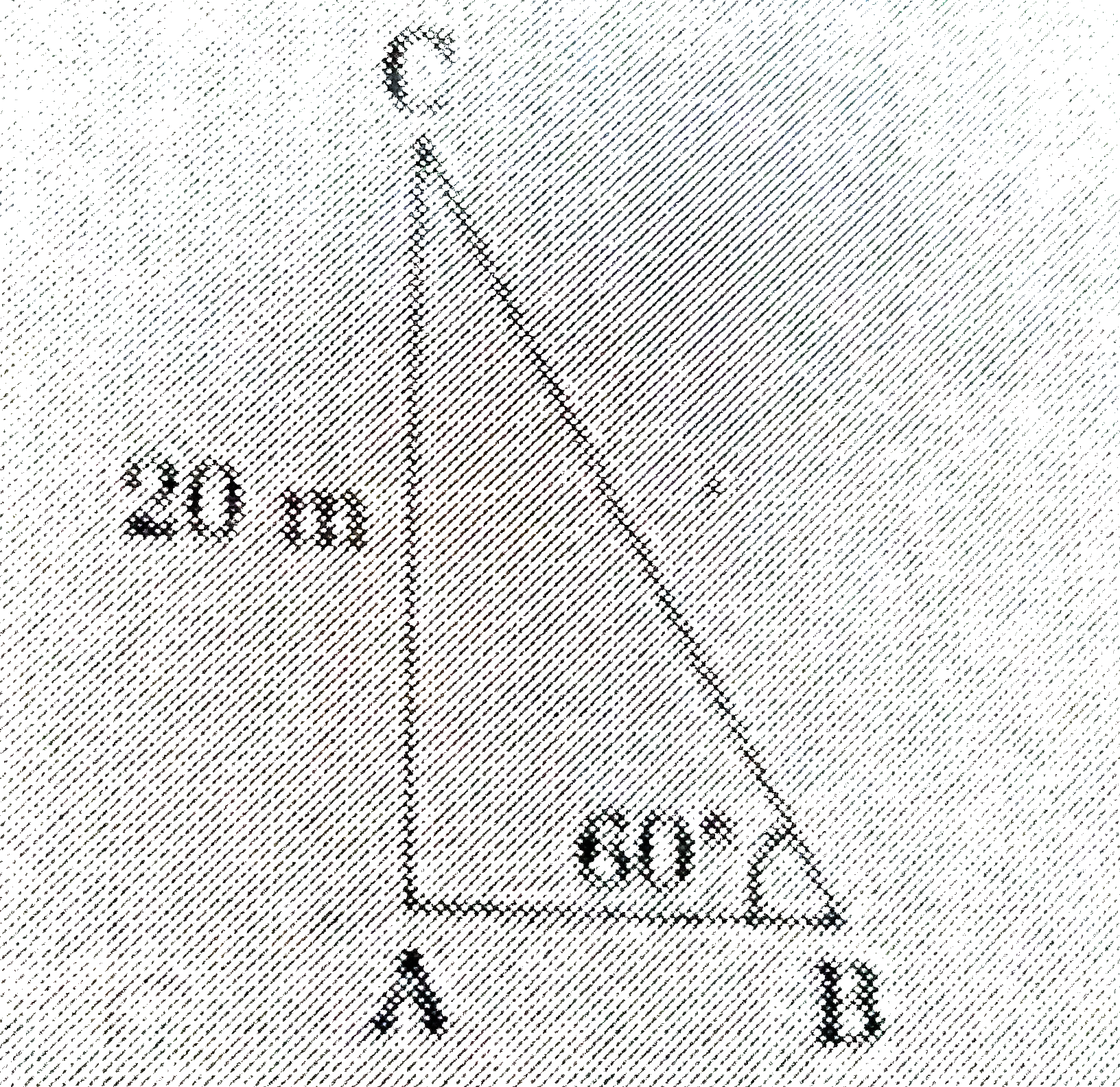
View SolutionIn figure , the angle of elevation of the top of a tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m, Find the distance of the point from the foot of the power .
View SolutionFrom a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ . The height of the tower is
View SolutionThe angle of depression of a point from the top of a 200 m high tower is 45∘. The distance of the point from the tower is
View SolutionAt a point 20 m away from the foot of a tower, the angle of elevation of the top of the tower is 30∘ The height of the tower is
View Solution
