Two point charges and are placed on the x-axis at x = 1 m and x = 4 m respectively. The electric field (in V/m) at a point y =3 m on y-axis is,
To find the electric field at the point (0, 3) m on the y-axis due to the two point charges q1 and q2, we will follow these steps:
Step 1: Identify the Charges and Their Positions
- Charge q1=√10μC is located at (1,0) m.
- Charge q2=−25μC is located at (4,0) m.
Step 2: Calculate the Distances from the Charges to the Point
- For q1:
r1=√(1−0)2+(0−3)2=√12+32=√1+9=√10m
- For q2:
r2=√(4−0)2+(0−3)2=√42+32=√16+9=√25=5m
Step 3: Calculate the Electric Fields due to Each Charge
Using the formula for electric field:
E=14πϵ0⋅Qr2
Given 14πϵ0=9×109N m2/C2.
Electric Field due to q1:
E1=9×109⋅√10×10−6(√10)2=9×109⋅√10×10−610=9×109⋅√10102=9×109⋅√10100
E1=9√10×107N/m2
Electric Field due to q2:
E2=9×109⋅−25×10−6(5)2=9×109⋅−25×10−625=9×109⋅(−10−6)=−9×103N/m2
Step 4: Determine the Direction of Each Electric Field
- The electric field E1 due to q1 (positive charge) points away from the charge, directed upwards and to the left (in the positive y-direction).
- The electric field E2 due to q2 (negative charge) points towards the charge, directed downwards and to the right (in the negative y-direction).
Step 5: Resolve the Electric Fields into Components
- For E1:
- E1x=−9√10×107⋅1√10=−9×107N/m2
- E1y=9√10×107⋅3√10=27×107N/m2
- For E2:
- E2x=0 (no x-component since it acts vertically)
- E2y=−9×103N/m2
Step 6: Sum the Electric Field Components
- Total Ex=E1x+E2x=−9×107+0=−9×107N/m2
- Total Ey=E1y+E2y=27×107−9×103=27×107−0.009×107=26.991×107N/m2
Step 7: Final Result
The total electric field at the point (0,3) m is given by:
→E=(−9×107^i+26.991×107^j)N/m2
- Charge q1=√10μC is located at (1,0) m.
- Charge q2=−25μC is located at (4,0) m.
- For q1:
r1=√(1−0)2+(0−3)2=√12+32=√1+9=√10m
- For q2:
r2=√(4−0)2+(0−3)2=√42+32=√16+9=√25=5m
Using the formula for electric field:
E=14πϵ0⋅Qr2
Given 14πϵ0=9×109N m2/C2.
E1=9×109⋅√10×10−6(√10)2=9×109⋅√10×10−610=9×109⋅√10102=9×109⋅√10100
E1=9√10×107N/m2
E2=9×109⋅−25×10−6(5)2=9×109⋅−25×10−625=9×109⋅(−10−6)=−9×103N/m2
- The electric field E1 due to q1 (positive charge) points away from the charge, directed upwards and to the left (in the positive y-direction).
- The electric field E2 due to q2 (negative charge) points towards the charge, directed downwards and to the right (in the negative y-direction).
- For E1:
- E1x=−9√10×107⋅1√10=−9×107N/m2
- E1y=9√10×107⋅3√10=27×107N/m2
- E2x=0 (no x-component since it acts vertically)
- E2y=−9×103N/m2
- Total Ex=E1x+E2x=−9×107+0=−9×107N/m2
- Total Ey=E1y+E2y=27×107−9×103=27×107−0.009×107=26.991×107N/m2
The total electric field at the point (0,3) m is given by:
→E=(−9×107^i+26.991×107^j)N/m2
Topper's Solved these Questions
Similar Questions
Point charges q1=4 μC and q2 = -2 μC are kept at point x = 0 and x = 4 respectively , electrical potential will be zero at points (where x is in cm)
View SolutionTwo point charges of, 1 μC and -1 μC are kept at (-2 m, 0) and (2 m, 0) respectively. Calculate the electric field at point (0, 5 m).
View Solution
Knowledge Check
Point charge q1=2μC and q2=−1μC are kept at points x=0 and x=6 respectively. Electrical potential will be zero at points
Ax=2andx=9Bx=1andx=5Cx=4andx=12Dx=−2andx=2Two point charges q1=4μC and q2=9μC are placed 20cm apart. The electric field due to them will be zero on the line joining them at distamce of
A8cm from q1B8cm from q2C8013cm from q1D8013cm from q2Two point charges q1andq2, of magnitude +10–8Cand–10–8C, respectively, are placed 0.1 m apart. Calculate the electric fields at points A, B and C shown in Fig. 1.14.
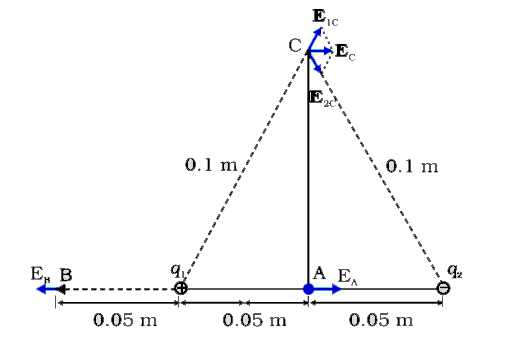 AEA=7.2×104NC−1 EB=3.2×104NC−1
AEA=7.2×104NC−1 EB=3.2×104NC−1
EB is directed towards the RIGHT.BEA=7.2×10−4NC−1 EB=3.2×104NC−1
EB is directed towards the left.CEA=7.2×104NC−1 EB=3.2×104NC−1
EB is directed towards the left.DEA=7.2×104NC−1 EB=3.2×10−4NC−1
EB is directed towards the left.
Ten charges having charge 1 μ C 8 μ C 27 μ C ....1000 μ C are placed on the x-axis with co-ordinates x=1m 2m 3m 4m....10m .The net electric field intensity at the origin is
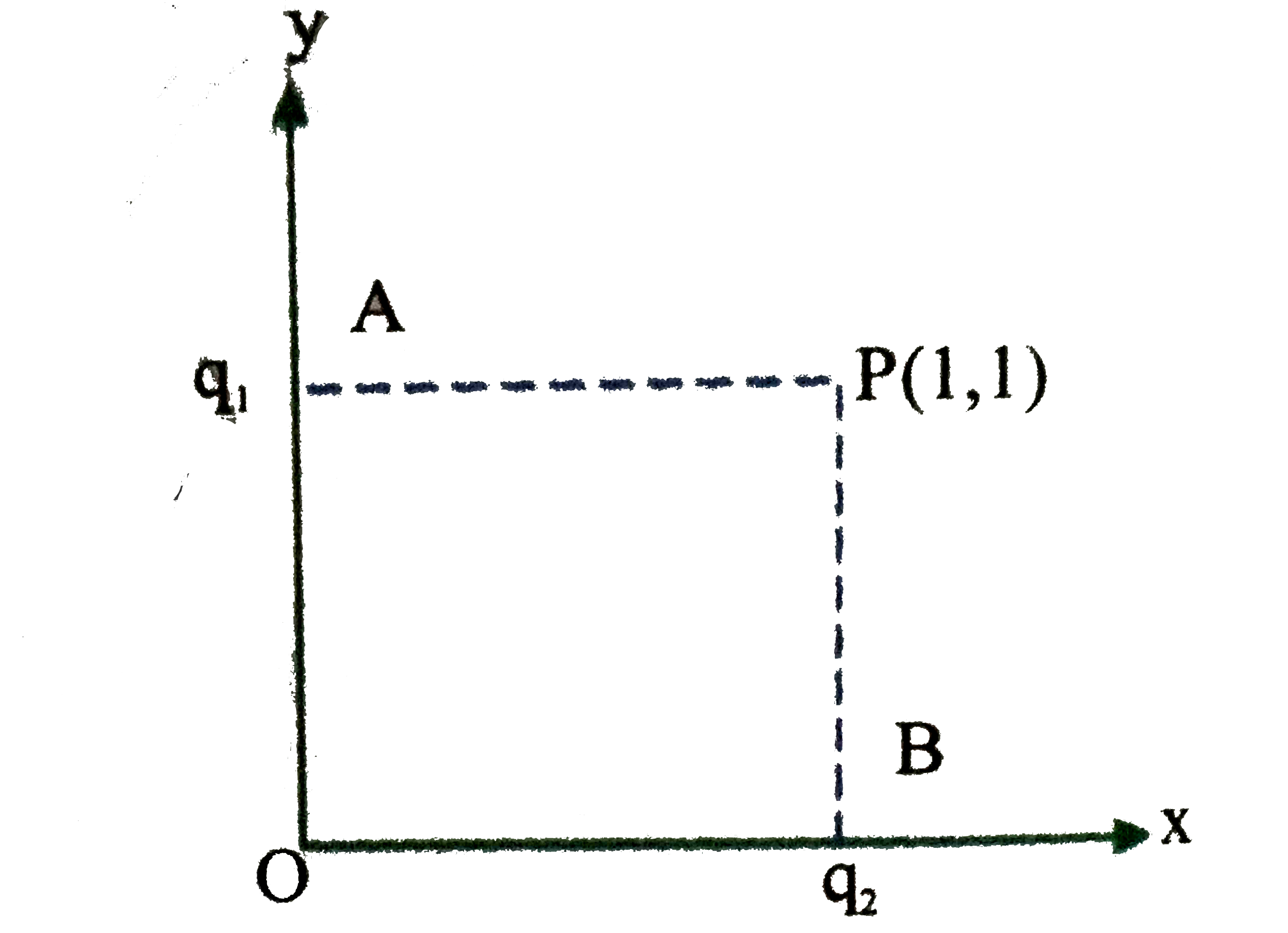
View SolutionTwo points charge q1 and q2(=q1/2) are placed at points A(0,1) and B(1,0) as shown in the figure.The electric field vector at point P(1,1) makes an angle q with the x-axis ,then the angle q is
View SolutionTwo charges of 4μC each are placed at the corners A and B of an equilaternal triangle of side length 0.2m in air. The
electric potential at C is [14πε0=9×109N−m2C2]
View SolutionWhat is the magnitude of a point charge due to which the electric field 30 cm away has the magnitude 2 ? [1/4πε0=9×109N−m2/c2]
View SolutionThe electric field due to a charge at a distance of 3 m from it is 500NC−1. The magnitude of the charge is
[14πε0=9×109N−m2/C2]
View Solution