A heat source at T = 103 K is connected to another heat reservoir at T = 102 K by a copper slab which is 1 m thick. Given that the thermal conductivity of copper is 0.1 WK−1m−1, the energy flux through it in steady state is :
90 W m−2
120 W m−2
65 W m−2
200 W m−2
To solve the problem, we need to calculate the energy flux through the copper slab in steady state using the formula for heat conduction. The formula we will use is derived from Fourier's law of heat conduction:
q=k⋅ΔTL
where:
- q is the energy flux (heat flow per unit area per unit time),
- k is the thermal conductivity of the material (in this case, copper),
- ΔT is the temperature difference across the slab,
- L is the thickness of the slab.
1. Identify the Given Values:
- Thermal conductivity of copper, k=0.1W K−1m−1
- Temperature of the heat source, T1=103K=1000K
- Temperature of the heat reservoir, T2=102K=100K
- Thickness of the copper slab, L=1m
2. Calculate the Temperature Difference:
ΔT=T1−T2=1000K−100K=900K
3. Substitute the Values into the Formula:
q=k⋅ΔTL=0.1W K−1m−1⋅900K1m
4. Calculate the Energy Flux:
q=0.1⋅900=90W/m2
Thus, the energy flux through the copper slab in steady state is 90 W/m².
- q is the energy flux (heat flow per unit area per unit time),
- k is the thermal conductivity of the material (in this case, copper),
- ΔT is the temperature difference across the slab,
- L is the thickness of the slab.
- Thermal conductivity of copper, k=0.1W K−1m−1
- Temperature of the heat source, T1=103K=1000K
- Temperature of the heat reservoir, T2=102K=100K
- Thickness of the copper slab, L=1m
ΔT=T1−T2=1000K−100K=900K
q=k⋅ΔTL=0.1W K−1m−1⋅900K1m
q=0.1⋅900=90W/m2
Topper's Solved these Questions
Similar Questions
A hollow spherical conducting sheel of inner radius R1=0.25m and outer radius R2=0.50m is placed inside a heat reservoir of temperature T0=1000∘C. The shell is initially filled with water at 0∘C. The thermal conductivity of the material is k=1024πW/m−K and its heat capacity is negligible. The time required to raise the temperature of water to 100∘Cis1100ln.109sec. Find K. Take specific heat of water s=4.2kJ/kg.∘C, density of water dw=1000kg/m3π=227

View SolutionSteam at 373 K is passed through a tube of radius 10cm and length10cm and length 2m. The thickness of the tube is 5mm and thermal conductivity of the material is390Wm−1K−1, calculate the heat lost per second. The outside temperature is 0∘C.
View Solution
Knowledge Check
A heat source at T = 500 k is connected to another heat reservoir at t = 100 k by a copper slab which is 1 m long. Given that the thermal conductivity of copper is 0.1Wk−1m−1 , the energy flux through it in the steady state is:
A4000Wm−2B400Wm−2C40Wm−2D4Wm−2A rod of 1m length and area of cross-section 1cm2 is connected across two heat reservoirs at temperature 100∘C and 0∘C as shown. The heat flow per second through the rod in steady state will be [Thermal conductivity of material of rod =0.09kilocalm−1s−1('∘C)]
 A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/sec
A9×10−4kilocal/secB9kilocal/secC0.09kilocal/secD9×10−6kilocal/sec1.56×105J of heat is conducted through is 2m2 wall of 12 cm thick in one hour. Temperature difference between the two sides of the wall is 20∘C. The thermal conductivity of the material of the wall is (in Wm−1K−1)
A0.11B0.13C0.15D1.2
A heater of resistance 20 Ω is used to heat a room at 10∘C and is connected to 220 V mains. The temperature is uniform inside the room and heat is transmitted outside the room through a glass window of area 1.2m2 and thickness 0.1 cm. If T∘C is the temperature outside and thermal conductivity of glass is0.2cals−1m−1∘C−1 , then find the value of 10T - 75 close to nearest integer.
View SolutionThe thermal power of density 100J/m^(3) is generated at a constant rate inside a uniform sphere of radius 10m and thermal conductivity 0.25wm−1k−1 .The temperature inside the sphere at a distance "5m" from center will be, if steady state temperature at surface is 600k
View SolutionA bar of copper of length 75 cm and a bar of steel of length 125 cm are joined together end to end . Both are of circular cross - section with diameter 2 cm . The free ends of the copper and steel bars are maintained at 100∘C and 0∘C respectively . The surface of the bars are thermally insulted . What is the temperature of the copper - steel junction ? What is the heat transmitted per unit time across the junction ? Thermal conductivty of copper 9.2×10−2kcalm−1∘C−1s−1 and that of steel is 1.1×10−2kcalm−1s−1(∘)C−1.
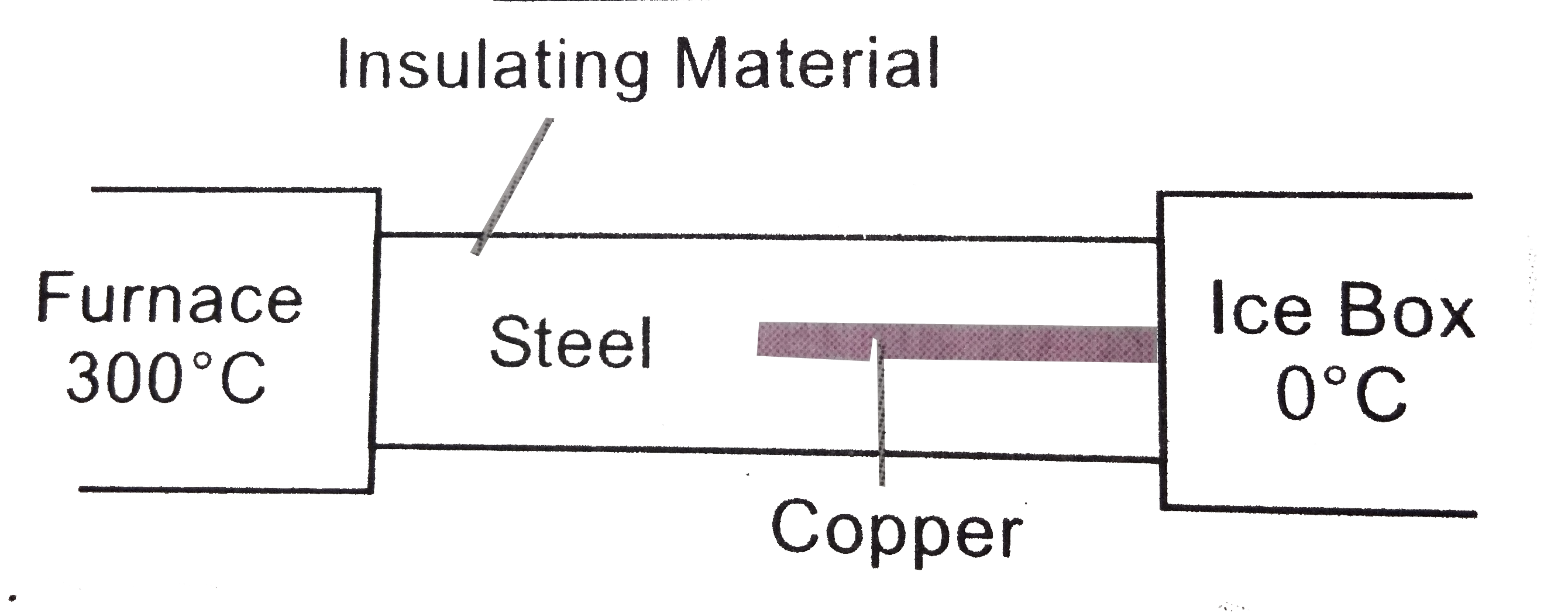
View SolutionWhat is the temperature of the steel-copper junction in the steady state system show in Fig. 7(e).17? Length of steel rod = 15.0 cm, length of the copper rod = 10.0 cm, temperature of the furnace =300∘C, temperature of other end 0∘C. The are of cross-section of the steel rod is twice that of the copper rod. (Thermal conductivity of steel =50.2js−1m−1K−1 and of copper =3895js−1m−1K−1).
View Solution(a) Calculate the rate at which body heat is conducted through the clothing of a skier in a steady-state process, given the following data: the body surface area is 1.8m2, and the clothing is 1.0 cm thick , the skin surface temperature is 33∘C and the outer surface of the clothing is at 1.0∘C, the thermal conductivity of the clothing is 0.040W/m.K. (b) If , after a fall, the skier's clothes became soaked with water of thermal conductivity 0.60W/m.K, by how much is the rate of conduction multiplied ?
View Solution
