A person standing on an open ground hears the sound of a jet aeroplane , coming from north at an angle $60^{\circ}$ with ground level ,But he finds the aeroplane right vertically above his position , if V is the speed of sound , speed of the plane is :

$\frac{\sqrt{3}}{2} v$

$\frac{2 v}{\sqrt{3}}$

$\frac{v}{2}$

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem step by step, we can follow these instructions:
Step 1: Understand the scenario
A person hears the sound of a jet airplane coming from the north at an angle of 60 degrees with the ground. However, when he looks up, he sees the airplane directly above him.
Step 2: Set up the diagram
We can visualize the situation:
- Let point P be the position of the airplane when it emits the sound.
- Let point A be the position of the observer on the ground.
- Let point B be the point directly below the airplane when the observer hears the sound.
- The angle of elevation from the observer to the airplane is 60 degrees.
Step 3: Analyze the distances
- The distance AB represents the distance the sound travels to reach the observer.
- The distance AC represents the distance the airplane travels during the time the sound travels from point P to point A.
Step 4: Use trigonometry
From the triangle formed by points A, B, and C:
- We know that cos(60°) = adjacent/hypotenuse = AC/AB.
- Therefore, we can write:
$cos (60 °) = \frac{A C}{A B}$
Since $cos (60 °) = \frac{1}{2}$ , we have:
$\frac{A C}{A B} = \frac{1}{2}$
Step 5: Relate the distances to speeds
Let:
- $V$ be the speed of sound.
- $V_{p}$ be the speed of the airplane.
The time taken for the sound to travel from P to A is:
$t = \frac{A B}{V}$
The time taken for the airplane to travel from P to C is:
$t = \frac{A C}{V_{p}}$
Since both times are equal:
$\frac{A B}{V} = \frac{A C}{V_{p}}$
Step 6: Substitute the relationship from step 4
From the relationship $A C = \frac{1}{2} A B$ , we can substitute:
$\frac{A B}{V} = \frac{\frac{1}{2} A B}{V_{p}}$
Step 7: Simplify the equation
Cancel $A B$ from both sides (assuming $A B \neq 0$ ):
$\frac{1}{V} = \frac{1 / 2}{V_{p}}$
Step 8: Solve for $V_{p}$
Cross-multiplying gives:
$V_{p} = \frac{1}{2} V$
Conclusion
The speed of the airplane is:
$V_{p} = \frac{V}{2}$
Final Answer
The speed of the plane is $\frac{V}{2}$ .
---

Updated on:7/8/2024

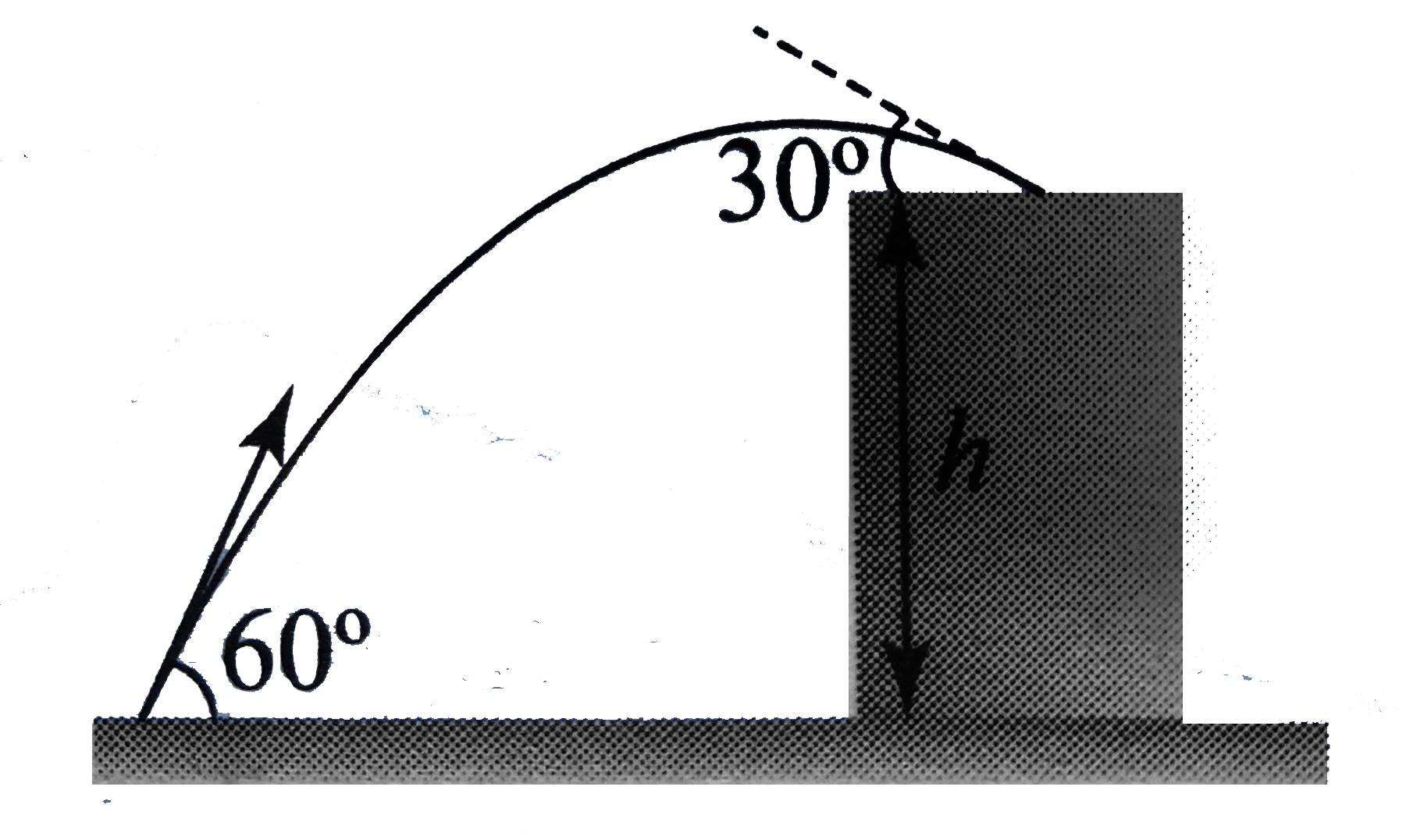
A stone projected at an angle of $60^{\circ}$ from the ground level strikes at an angle of $30^{\circ}$ on the roof of a building of height $h$ . Then the speed of projection of the stone is:
A $\sqrt{2 g h}$
B $\sqrt{6 g h}$
C $\sqrt{3 g h}$
D $\sqrt{g h}$
The angle of elevation of an aeroplane from a point on the ground is $60^{\circ}$ .After 15 seconds flight ,the elevation changes to $30^{\circ}$ .If the aeroplane is flying at a height of $1500 \sqrt{3}$ m , find the speed of the plane
A300 m / sec
B200 m /sec
C100 m /sec
D150 m/sec
The angle of elevation of an aeroplane from a point on the ground is $60^{\circ}$ . After flying for 30 seconds, the angle of elevation charges to $30^{\circ}$ . If the aeroplane is flying at a height of 4500 m, then what is the speed (in m/s) of aeroplane
A $50 \sqrt{} 3$
B $100 \sqrt{} 3$
C $200 \sqrt{} 3$
D $300 \sqrt{} 3$