This problem is quite challenging in setting up but takes only a few of algebra to solve. We deal with not only uniformly circular motion but also a ramp. However we will not need a tilted coordinate system as with other ramps. Insttead we can take a freeze frame of the motion and work with simply horizontaly and vertical axes. As always in this chapter, the starting point will be to apply Newton's second law, but that will required us to identify the force component that is responsible for the uniform circular motion.
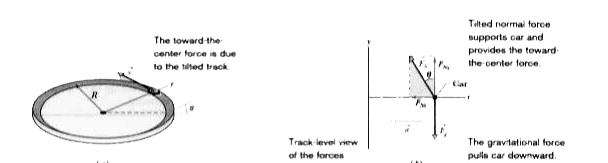
Curved portions of highways. are always banked (tilted) to prevent cars from sliding off the highway. When a highway is dry, the frictional force between the tires and the road surface may be enough to prevent sliding. When the highway is wet, however, the frictional force may be neglifible, and baking is then essential. fig represents a car of mass m as it moves at a constant speed v to 20m/s around a banked circular track of radius R=190m. (It is a normal car, rather than a race car, which meas that any vertical force from the passing air is negligible). If the frictional force from the track is negligible, what bank angle θ prevents sliding?
Text Solution
Here the track is banked so as to tilt the normal force →FN on the car toward the center of the circle.
Thus →FN, now has a centripetal component of magnitude FNr, directed inward along a radiua axis r. We want to find the value off the bank angle θ such that this centripetal component keeps the car onthe circular track witout need of friction.
Radial calculation: As fig shows (and as you should verity), the angle that force →FN makes with the verical is equal to the bank angle θ of the track. Thus, the radial component FNr is equal to FNsinθ. We can now write Newton.s second law for components along the r axis (Fnet,r=mar) is
−FN−sinθm(−v2R)
We cannot solve this equation for the value of θ because it also contains the unknowns FN and m.
Vertical calculations: We next consider the forces and acceleration along the y axis in fig. The vertical component of the normal force is FNy=FNcosθ, the gravitational force →Fg on the car has the magnitude mg and the acceleration of the car along the y axis is zero. Thus we ca write Newton.s second law for components along the y axis (Fnet,y=may) as
FNcosθ−mg=m(0)
From whcih
FNcosθ=mg
Combining results: Equation also contains the unknowns FN and m but note that dividing eq. by eq neatly eliminates both those unknowns. Doing se replacing (sinθ)/(cosθ) with tanθ, and solving for θ then yield
θ=tan−1(v2)gR
=tan−1(20m/s)2(9.8m/s2)(190m)=122
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PROBLEMS56videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCULAR MOTIONEXERCISE - PRACTICE QUESTIONS(SINGLE CORRECT CHOICE TYPE)40videos
CIRCULAR MOTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Integer Type)3videos
CIRCUITS
- BOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Integer Type)3videos
CURRENT AND RESISTANCE
Similar Questions
Knowledge Check
- Question 1 - Select One
The kinematical equation of motion are applied to solve the problems of circular motion , because of
Athe acceleration is non uniformBthe acceleration is uniformCthe acceleration and velocity are uniformDthe motion is circular - Question 2 - Select One
If we need to protect our crops from mosquitoes then we can use
ALadyibird beetleBDragonfliesCBacillus thuringiensisDBoth A and B - Question 3 - Select One
Assertion uniform circular motion is uniformly accelerated motion.
Reason acceleration in uniform circular motion is always towards centre.AIf both Assertion and Reason are correct and Reason is the correct explanation of Assertion.BIf both Assertion and Reason are correct but Reason is not the correct explanation of Assertion.CIf Assertion is true but Reason is false.DIf Assertion is false but Reason is true.
We need __________ coordinates for representing a point on the graph sheet.
View SolutionAssertion : A uniform circular motion is an accelerated motion.
Reason : Direction of acceleration is parallel to be velocity vector.View SolutionWhen can we say that a particle in motion is moving with uniform speed?
View SolutionWhen can we say that a particle in motion is moving with uniform speed?
View SolutionIn order to apply Newton's law of motion in an accelerated (non-inertial ) frame of reference, we make the use fo
View Solution

