Figure 21-38 shows a reversible cycle through which 1.00 mol of a monatomic ideal gas is taken. Volume VC = 8.00 Vb Process Adiabatic bc is an adiabatic expansion, with pb=5.00 atm and Vb=1.00×10−3m3. For the cycle, find (a) the energy added to the gas as heat, (b) the energy leaving the gas as Volume heat, (c) the network done by the gas, and (d) the efficiency of the cycle.
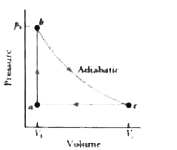
(a)734J,(b)277J,(c)457j:(d)62.3%
Topper's Solved these Questions
- THE FIRST LAW OF THERMODYNAMICSBOOK - RESNICK AND HALLIDAYCHAPTER - THE FIRST LAW OF THERMODYNAMICSEXERCISE - PRACTICE QUESTIONS ( SINGLE CORRECT CHOICE TYPE )
48 Videos
- THE FIRST LAW OF THERMODYNAMICSBOOK - RESNICK AND HALLIDAYCHAPTER - THE FIRST LAW OF THERMODYNAMICSEXERCISE - PRACTICE QUESTIONS ( MORE THAN ONE CORRECT CHOICE TYPE )
9 Videos
- THE FIRST LAW OF THERMODYNAMICSBOOK - RESNICK AND HALLIDAYCHAPTER - THE FIRST LAW OF THERMODYNAMICSEXERCISE - CHECK POINT
8 Videos
- TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION BOOK - RESNICK AND HALLIDAYCHAPTER - TEMPERATURE, ZEROTH LAW OF THERMODYNAMICS AND THERMAL EXPANSION EXERCISE - PRACTICE QUETIONS (Integer Type)
4 Videos
- THE KINETIC THEORY OF GASESBOOK - RESNICK AND HALLIDAYCHAPTER - THE KINETIC THEORY OF GASESEXERCISE - PRACTICE QUESTIONS
72 Videos
Similar Questions
Suppose 1.80 mol of an ideal gas is taken from a volume of 3,00 m3 to a volume of 5.50m3 via an isothermal expansion at 30∘C. (a) How much energy is transferred as heat during the process, and (b) is the transfer to or from the gas?
View SolutionA 2.00 mol sample of helium gas initially at 300 K and 0.400 atm is compressed isothermally to 1.20 atm. Noting that the helium behaves as an ideal gas, find (a) the final volume of the gas, (b) the work done on the gas and (c ) the energy transferred by heat.
View SolutionA 2.00 mol sample of helium gas initially at 300 K and 0.400 atm is compressed isothermally to 1.20 atm. Noting that the helium behaves as an ideal gas, find (a) the final volume of the gas, (b) the work done on the gas and (c ) the energy transferred by heat.
View SolutionA heat engine is a system that converts heat into mechanical work. A heat "source" generates thermal energy that brings a working substance to a high temperature. The working substance then generates work in the engine while transferring heat to a sink at a lower temperature . The working of a heat engine is shownn figure 1.
 Heat engines can be modelled using themodynamic cycles. The heat engine given in Figure -2 is of a working substance which is 1.00 mol of a monoatomic ideal gas . The thermodynamic cycle begins at the point designated as '1' and goes clockwise and the values of P and l or V at each point is as given below Fig-2 (P1=1.00atmandV1=24.6L,P2=2.00atm,V3=49.2L,P4=1.00atm)
Heat engines can be modelled using themodynamic cycles. The heat engine given in Figure -2 is of a working substance which is 1.00 mol of a monoatomic ideal gas . The thermodynamic cycle begins at the point designated as '1' and goes clockwise and the values of P and l or V at each point is as given below Fig-2 (P1=1.00atmandV1=24.6L,P2=2.00atm,V3=49.2L,P4=1.00atm)
(a) What is the entropy change of the system for the complete cycle?
(b) If the cycle is reversible , what is the temperature T3?
View SolutionAn ideal gas initially at 300 K undergoes an isobaric expansion at 2.50 k Pa. If the volume increases from 1.00m2 to 3.00m3 and 12.5 kJ is transferred to the gas by heat, what are (a) the change in its internal energy and (b) its final temperature ?
View SolutionAn ideal gas initially at 30 K undergoes an isobaric expansion at 2.50 k Pa. If the volume increases from 1.00m2 to 3.00m3 and 12.5 kJ is transferred to the gas by heat, what are (a) the change in its internal energy and (b) its final temperature ?
View SolutionOne mole of a diatomic ideal gas (γ=1.4) is taken through a cyclic process starting from point A. The process A→B is an adiabatic compression, B→C is isobaric expansion, C→D is an adiabatic expansion, and D→A is isochoric. The volume ratios are VA/VB=16andVC/VB=2 and the temperature at A is TA=300K. Calculate the temperature of the gas at the points B and D and find the efficiency of the cycle.
View SolutionOne mole of a diatomic ideal gas (γ=1.4) is taken through a cyclic process starting from point A. The process A→B is an adiabatic compression, B→C is isobaric expansion, C→D is an adiabatic expansion, and D→A is isochoric. The volume ratios are VA/VB=16andVC/VB=2 and the temperature at A is TA=300K. Calculate the temperature of the gas at the points B and D and find the efficiency of the cycle.
View SolutionAt 27∘C two moles of an ideal monatomic gas occupy a volume V. The gas expands adiabatically to a volume 2V. Calculate
(a) final temperature of the gas
(b) change in its internal energy and
(c) the work done by the gas during the process. [ R=8.31J/mol−K]
View SolutionAt 27∘C two moles of an ideal monatomic gas occupy a volume V. The gas expands adiabatically to a volume 2V. Calculate
(a) final temperature of the gas
(b) change in its internal energy and
(c) the work done by the gas during the process. [ R=8.31J/mol−K]
View Solution
