A uniform circular plate of radius 2R has a circular hole of radius R cut out of it. The centre of the smaller circle is at a distance of R/3 from the centre of the larger circle. What is the position of the centre of mass of the plate?

Video Solution

|

Answer

Step by step video & image solution for A uniform circular plate of radius 2R has a circular hole of radius R cut out of it. The centre of the smaller circle is at a distance of R/3 from the centre of the larger circle. What is the position of the centre of mass of the plate? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Similar Questions

Knowledge Check

Question 1 - Select One
Find the position of centre of mass of a uniform disc of radius R from which a hole of radius is cut out. The centre of the hole is at a distance R/2 from the centre of the disc.

A $\frac{R r^{2}}{2 (R^{2} - r^{2})}$ towards right of O
B $\frac{R r^{2}}{2 (R^{2} - r^{2})}$ towards left of O
C $\frac{2 R r^{2}}{(R^{2} + r^{2})}$ towards right of O
D $\frac{2 R r^{2}}{(R^{2} + r^{2})}$ towards left of O
Question 2 - Select One
A uniform circular disc of radius a is taken. A circular portion of radius b has been removed from it as shown in the figure. If the center of hole is at a distance c from the center of the disc, the distance $x_{2}$ of the center of mass of the remaining part from the initial center of mass O is given by

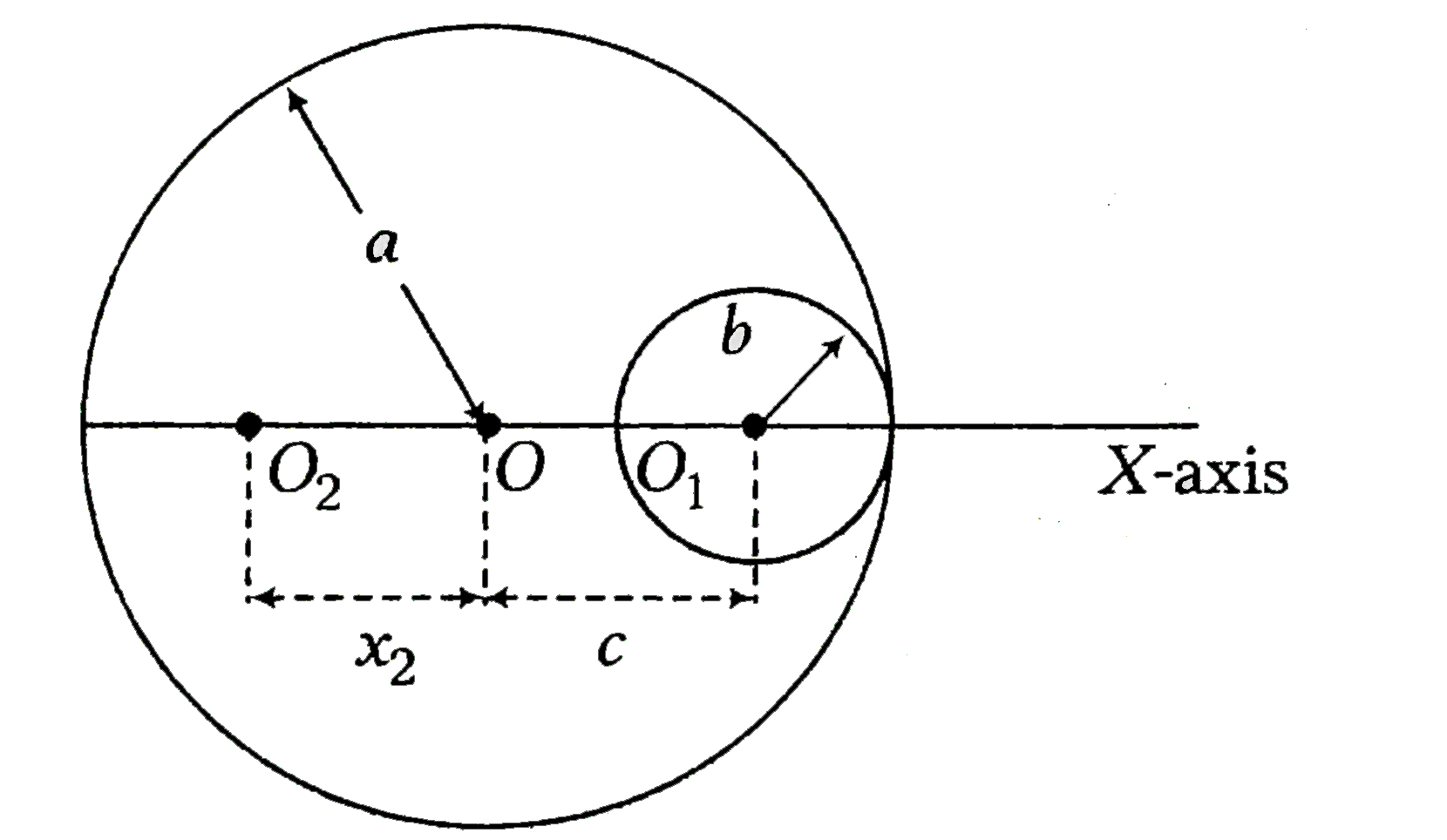A $\frac{π b^{2}}{(a^{2} - b^{2})}$
B $\frac{- c b^{2}}{(a^{2} - b^{2})}$
C $\frac{π c^{2}}{(a^{2} - b^{2})}$
D $\frac{π a^{2}}{(c^{2} - b^{2})}$
Question 3 - Select One
A circular hole of radius $r$ is made in a disk of radius $R$ and of uniform thickness at a distance $a$ from the centre of the disk. The distance of the new centre of mass from the original centre of mass is

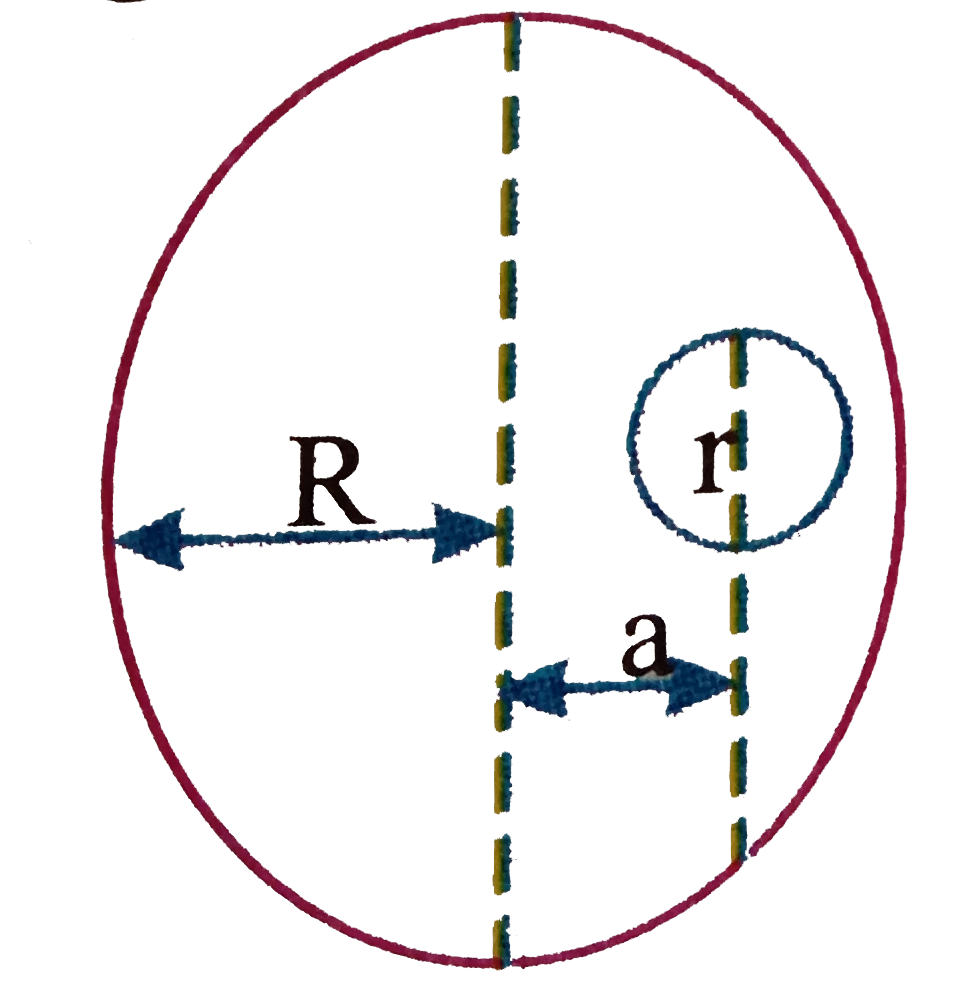A $\frac{a R^{2}}{R^{2} - r^{2}}$
B $\frac{a r^{2}}{R^{2} - r^{2}}$
C $\frac{a (R^{2} - r^{2})}{r^{2}}$
D $\frac{a (R^{2} - r^{2})}{R^{2}}$

Recommended Questions

Home
Ask Doubt
Profile